MaTek 1 Menggambar grafik Fungsi Linier #Part 6#Matematika Teknik 1
Summary
TLDRThis video tutorial guides viewers through graphing mathematical functions, focusing on linear and quadratic equations. The instructor demonstrates how to plot the graphs of y = 2x + 6 and 3x - y = 3, providing step-by-step instructions for creating tables of values and plotting the points on a coordinate grid. The lesson also covers graphing a quadratic function, y = x² - 1, emphasizing the use of multiple points for accuracy. The friendly and informal teaching style aims to make graphing clear and easy to understand for students.
Takeaways
- 😀 The lesson focuses on graphing linear and quadratic functions, starting with the basic principles of plotting points on a coordinate plane.
- 😀 The first function is a linear equation: y = 2x + 6. The teacher demonstrates how to calculate values and plot them on a graph using a table.
- 😀 For the linear function y = 2x + 6, the teacher starts by substituting x = 0, which results in y = 6, and then plots the point (0, 6).
- 😀 The second key point for the linear function is when y = 0, which leads to solving for x. Substituting y = 0 gives x = -3, resulting in the point (-3, 0).
- 😀 Once the points (0, 6) and (-3, 0) are plotted, the teacher emphasizes the importance of drawing a straight line through these points to form the graph of the function.
- 😀 The second linear function, y = 3x - 3, follows a similar process: first, the teacher finds the point (0, -3) and then the point (1, 0).
- 😀 For the second linear function, the teacher highlights how to plot these points and draw the corresponding straight line, just like in the first example.
- 😀 In the third example, the lesson switches to a quadratic function, y = x² - 1. The teacher suggests using a minimum of five points to graph the curve effectively.
- 😀 The teacher demonstrates how to calculate and plot points for the quadratic function, including points such as (0, -1), (1, 0), (-1, 0), (2, 3), and (-2, 3).
- 😀 The lesson concludes with the teacher encouraging students to ensure their lines and curves pass through all plotted points, emphasizing the importance of accuracy in graphing.
Q & A
What is the first step in graphing a linear function like y = 2x + 6?
-The first step is to fill out a table of values for x and calculate the corresponding y values using the given equation.
Why is it recommended to use at least two points when graphing a linear function?
-Using at least two points ensures that you can draw a straight line, which is characteristic of linear functions.
In the linear function y = 2x + 6, what are the coordinates when x = 0?
-When x = 0, y = 6, so the coordinates are (0, 6).
What do you do after plotting the points on the graph for a linear function?
-After plotting the points, connect them with a straight line using a ruler to ensure the line is accurate.
What is the purpose of labeling the line with its equation after graphing?
-Labeling the line with its equation helps identify and clarify which function the graph represents.
How do you graph the linear equation y = 3x - 3?
-First, fill out a table of values, plot the points (0, -3) and (1, 0), then draw a straight line through them.
What is the process for graphing a quadratic function like y = x² - 1?
-Start by filling out a table with values for x, plot the points on the coordinate grid, then connect the points with a curved line to form the 'U' shape of a quadratic function.
Why is it important to use more than two points when graphing a quadratic function?
-Using more than two points helps accurately represent the curve of the quadratic function, ensuring the graph shows the correct 'U' shape.
In the quadratic function y = x² - 1, what is the value of y when x = 0?
-When x = 0, y = -1, so the point is (0, -1).
How do you graph the quadratic function y = x² - 1 with the points (0, -1), (1, 0), and (-1, 0)?
-Plot the points on the graph, then draw a smooth curve through them. The curve should show the 'U' shape characteristic of quadratic functions.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
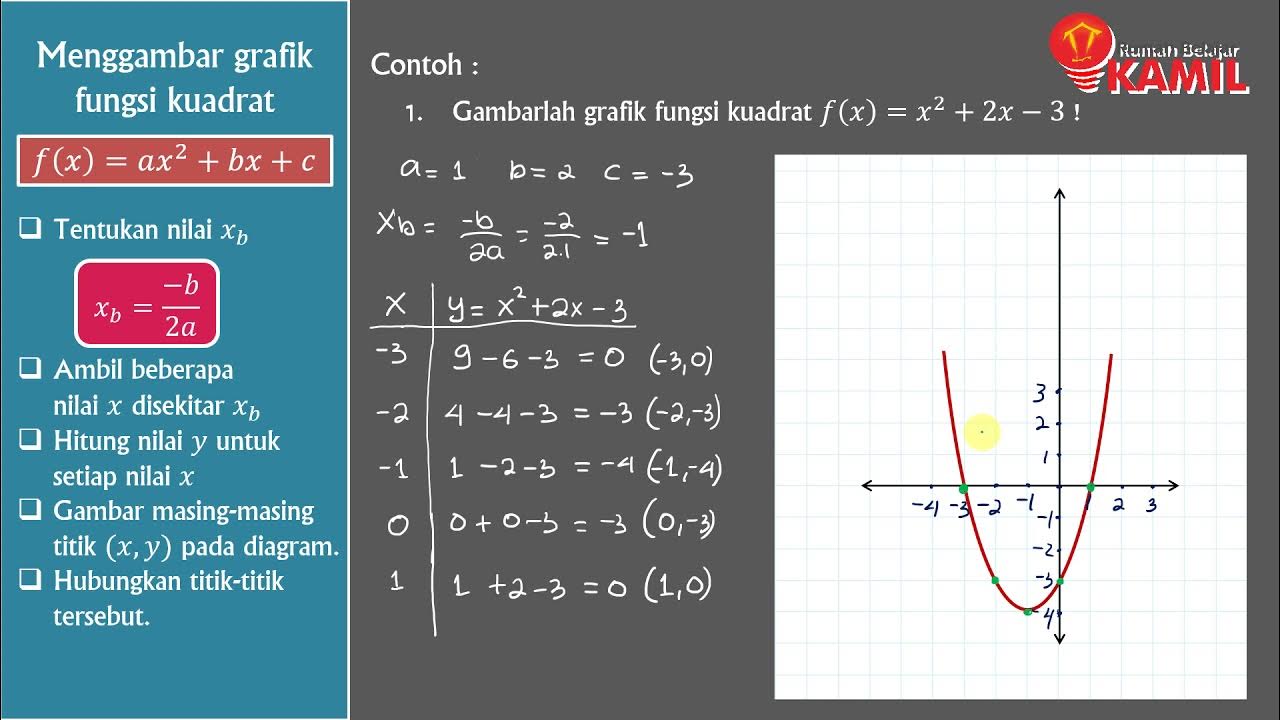
Matematika Kelas 9 : Fungsi Kuadrat (Part 3 : Menggambar grafik fungsi kuadrat)
How to Create Desmos Art 2 - Curves, Waves, Ellipses - Step by Step Guide
SPtDV Matematika Kelas 10 • Part 1: Pertidaksamaan Linear & Kuadrat Dua Variabel

Kwadratische vergelijkingen (HAVO wiskunde B)

Grafik Garis Lurus Hal 207-219 Bab 5 Persamaan Garis Lurus Kelas 8 Kurikulum Merdeka Belajar
Quadrilaterals - Trapezoids, Parallelograms, Rectangles, Squares, and Rhombuses!
5.0 / 5 (0 votes)