MATH 10 : DIFFERENTIATING GEOMETRIC SEQUENCE FROM AN ARITHMETIC SEQUENCE (Taglish)
Summary
TLDRThis script explains the difference between arithmetic and geometric sequences. In an arithmetic sequence, a constant difference is added to each term to find the next, while in a geometric sequence, a constant ratio is multiplied. Examples illustrate these concepts, showing how to identify common differences and ratios. The script also discusses representing these sequences on a Cartesian plane, highlighting the linear nature of arithmetic sequences and the exponential growth of geometric ones.
Takeaways
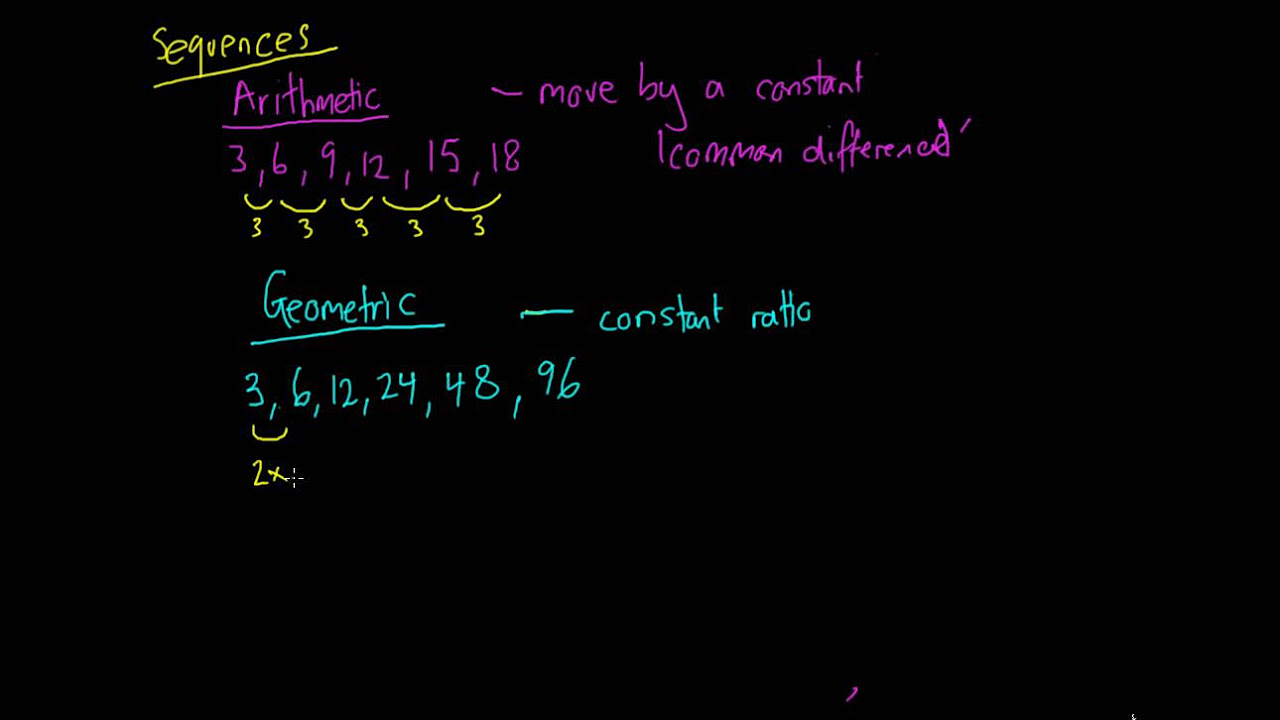
- 🔢 Arithmetic sequences are defined by adding a constant difference (d) to each term to get the next term.
- 🔄 Geometric sequences are defined by multiplying each term by a constant ratio (r) to get the next term.
- 📈 The common difference in an arithmetic sequence can be found by subtracting a term from its previous term.
- 📉 The common ratio in a geometric sequence can be found by dividing a term by its preceding term.
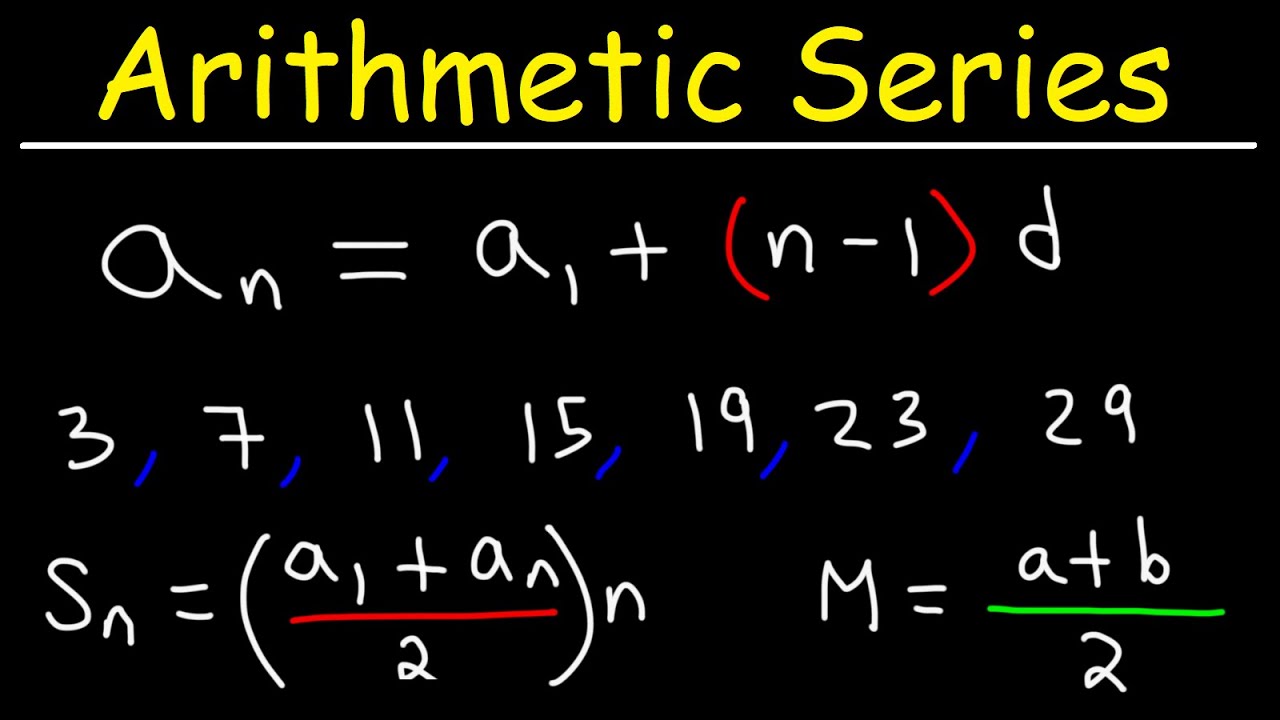
- 🌰 An example of an arithmetic sequence is 10, 15, 20, 25, 30, 35, where the common difference is 5.
- 🌐 An example of a geometric sequence is 3, 6, 12, 24, 48, 96, where the common ratio is 2.
- 📊 Arithmetic sequences can be visualized on a Cartesian plane as a straight line where the difference between points is constant.
- 📏 Geometric sequences, when graphed, may not form a straight line but show a consistent ratio between terms.
- 📋 The script discusses the process of identifying and differentiating between arithmetic and geometric sequences using tables of values.
- 🎓 Understanding the properties of arithmetic and geometric sequences is fundamental for various mathematical applications and functions.
Q & A
What is the main difference between an arithmetic sequence and a geometric sequence?
-An arithmetic sequence involves adding a constant difference to the previous term to get the next term, while a geometric sequence involves multiplying the previous term by a constant ratio to get the next term.
What is the term used for the constant added in an arithmetic sequence?
-The constant added in an arithmetic sequence is called the common difference.
How is the common ratio in a geometric sequence determined?
-The common ratio in a geometric sequence is determined by dividing any term by its preceding term.
Can you provide an example of an arithmetic sequence from the script?
-An example of an arithmetic sequence given in the script is 10, 15, 20, 25, 30, 35, where the common difference is 5.
What is the common ratio for the geometric sequence provided in the script?
-The common ratio for the geometric sequence 3, 6, 12, 24, 48, 96 is 2.
How can you identify the common difference in an arithmetic sequence by looking at its terms?
-You can identify the common difference in an arithmetic sequence by subtracting a term from its previous term; the result should be constant across all terms.
What does the script suggest about the relationship between terms in an arithmetic sequence when plotted on a Cartesian plane?
-When terms of an arithmetic sequence are plotted on a Cartesian plane, they form a straight line where the distance between consecutive points is equal.
What is the significance of the term 'domain' in the context of sequences as mentioned in the script?
-In the context of sequences, 'domain' refers to the set of possible input values, which in the case of sequences are typically the natural numbers starting from 1.
How does the script describe the process of identifying the range of values for a geometric sequence?
-The script describes identifying the range of values for a geometric sequence by calculating the output for each term using the starting value and the common ratio.
What is the significance of the term 'range' in sequences as explained in the script?
-The term 'range' in sequences refers to the set of output values generated by applying the sequence's rule to the domain.
How does the script differentiate between arithmetic and geometric sequences when it comes to their graphical representation?
-The script differentiates between arithmetic and geometric sequences by noting that arithmetic sequences form a straight line on a Cartesian plane with equal intervals between points, while geometric sequences are not explicitly described in terms of their graphical representation.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
Arithmetic vs Geometric Sequences
Arithmetic Sequences and Arithmetic Series - Basic Introduction

GCSE Maths - Types of Number Sequences - Arithmetic vs Geometric

Geometric Series and Geometric Sequences - Basic Introduction

Pembahasan BARISAN DAN DERET (Aritmetika & Geometri) KELAS 11 | #MatematikAsik

Barisan dan deret Geometri kelas 10
5.0 / 5 (0 votes)