GCSE Maths - Types of Number Sequences - Arithmetic vs Geometric

Summary

This video explains the difference between two main types of number sequences: arithmetic and geometric sequences. It highlights how arithmetic sequences involve adding or subtracting a constant value (called the common difference) to each term, while geometric sequences involve multiplying or dividing by a constant ratio (called the common ratio). Through examples, the video demonstrates how to identify the pattern in each sequence and how to find the common difference or ratio. The video concludes with a quick recap of both types of sequences and their properties.

Takeaways

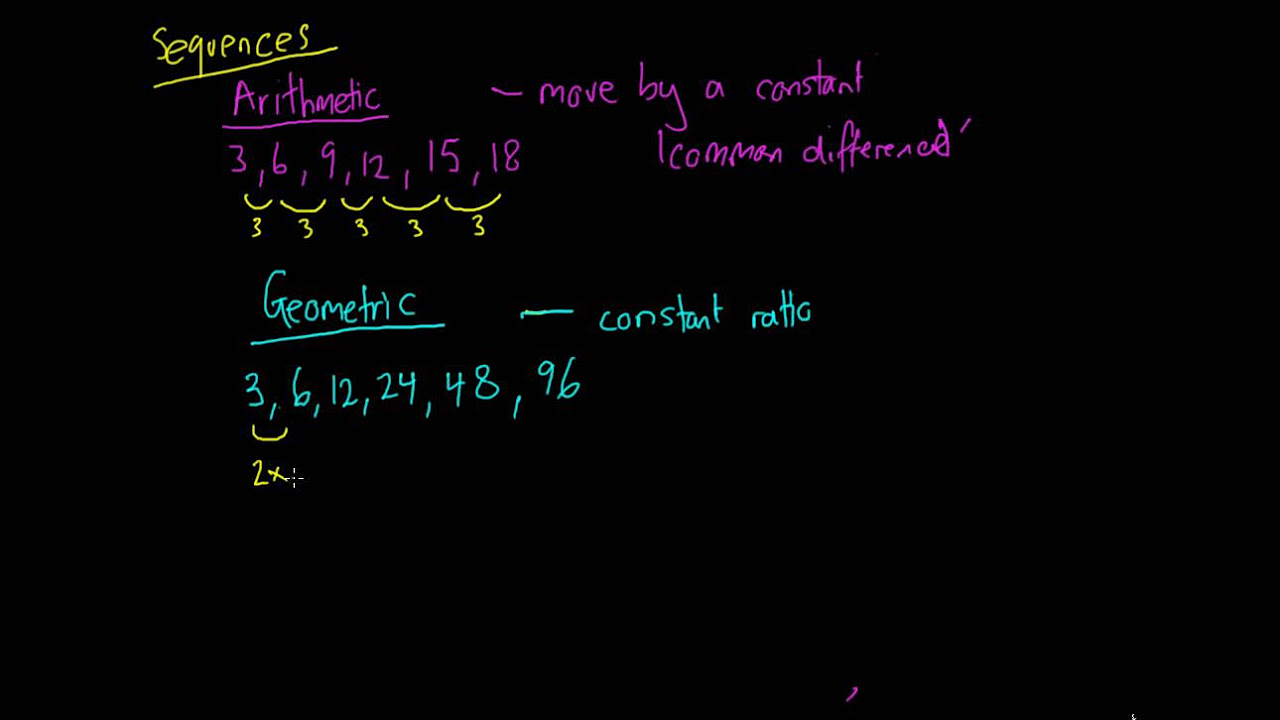
• 😀 Arithmetic sequences involve adding or subtracting a fixed number (common difference) to get from one term to the next.
• 😀 In an arithmetic sequence, the common difference remains constant throughout the sequence.
• 😀 Example of an arithmetic sequence: 4, 7, 10, 13, 16, with a common difference of +3.
• 😀 Geometric sequences involve multiplying or dividing a fixed number (common ratio) to get from one term to the next.
• 😀 In a geometric sequence, the common ratio remains constant throughout the sequence.
• 😀 Example of a geometric sequence: 3, 6, 12, 24, with a common ratio of ×2.
• 😀 To find the common ratio in a geometric sequence, divide any term by its previous term.
• 😀 In a geometric sequence, dividing by a number is the same as multiplying by the reciprocal of that number.
• 😀 Example of another geometric sequence: 27, 9, 3, 1, 1/3, with a common ratio of ÷3, which is the same as multiplying by 1/3.
• 😀 Arithmetic sequences are easy to identify because the terms increase or decrease by a fixed amount each time.
• 😀 Geometric sequences are easy to identify because each term is generated by multiplication or division by a fixed amount.
• 😀 When identifying sequences, it's helpful to check at least the first few terms to confirm the pattern.

Q & A

What is an arithmetic sequence?

An arithmetic sequence is a sequence where each term is obtained by adding or subtracting a constant number (called the common difference) from the previous term.

How do you identify the common difference in an arithmetic sequence?

The common difference in an arithmetic sequence is the amount that is consistently added or subtracted between consecutive terms. For example, in the sequence 4, 7, 10, 13, 16, the common difference is +3.

What is the common difference in the sequence 22, 18, 14, 10, 6?

The common difference in this sequence is -4, since each term is obtained by subtracting 4 from the previous term.

What is a geometric sequence?

A geometric sequence is a sequence where each term is obtained by multiplying or dividing the previous term by a constant number (called the common ratio).

How do you determine the common ratio in a geometric sequence?

The common ratio in a geometric sequence is the constant factor by which each term is multiplied or divided to obtain the next term. For example, in the sequence 3, 6, 12, 24, the common ratio is 2, because each term is multiplied by 2.

What is the common ratio in the sequence 27, 9, 3, 1, 1/3?

The common ratio in this sequence is 1/3, because each term is obtained by dividing the previous term by 3, which is equivalent to multiplying by 1/3.

What is the difference between an arithmetic sequence and a geometric sequence?

An arithmetic sequence involves adding or subtracting the same number (common difference) between terms, whereas a geometric sequence involves multiplying or dividing the terms by the same number (common ratio).

Can an arithmetic sequence involve multiplication or division?

No, an arithmetic sequence only involves addition or subtraction of the same number between terms. If multiplication or division is involved, the sequence is a geometric sequence.

In a geometric sequence, what is the significance of dividing terms instead of multiplying them?

In a geometric sequence, dividing terms by a number is equivalent to multiplying them by the reciprocal of that number. For example, dividing by 3 is the same as multiplying by 1/3.

How can you check if a sequence is geometric or arithmetic?

To check if a sequence is arithmetic, calculate the difference between consecutive terms. If the difference is constant, it's arithmetic. To check if a sequence is geometric, divide each term by its previous term. If the ratio is constant, it's geometric.