Arithmetic vs Geometric Sequences
Summary
TLDRThis video script explains the difference between arithmetic and geometric sequences. Arithmetic sequences progress by a constant difference, as demonstrated by the sequence 3, 6, 9, 12, 15, 18. In contrast, geometric sequences progress by a constant ratio, exemplified by the sequence 3, 6, 12, 24, 48, 96. The script visually contrasts these sequences, showing arithmetic sequences as straight lines and geometric sequences as curves, highlighting their distinct characteristics.
Takeaways
- 🔢 Arithmetic sequences move by a constant amount, known as the common difference.
- 🔄 The common difference is calculated by subtracting consecutive terms in the sequence.
- 📈 An arithmetic sequence forms a straight line when graphed, due to its constant movement.
- 🔢 Geometric sequences move by a constant ratio, known as the common ratio.
- 🔄 The common ratio is determined by dividing consecutive terms in the sequence.
- 📈 Geometric sequences form a curved shape when graphed, due to their constant scaling.
- 🔑 The first term of both arithmetic and geometric sequences in the script is 3.
- 🔄 In the arithmetic sequence, each term increases by 3 to get to the next term.
- 🔄 In the geometric sequence, each term is multiplied by 2 to get to the next term.
- 📊 Both sequences start at the same point (3) on the graph, but diverge as they progress.
- 📚 The video script encourages viewers to watch another video for more details on arithmetic sequences.
Q & A
What is an arithmetic sequence?
-An arithmetic sequence is a sequence of numbers in which each term after the first is obtained by adding a constant difference to the previous term.
What is the common difference in an arithmetic sequence?
-The common difference in an arithmetic sequence is the constant value that is added to each term to get the next term.
How does the arithmetic sequence in the script start?
-The arithmetic sequence in the script starts with the number 3.
What is the common difference between the first two terms of the arithmetic sequence in the script?
-The common difference between the first two terms of the arithmetic sequence in the script is 3 (6 - 3 = 3).
What is a geometric sequence?
-A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a constant ratio.
What is the common ratio in a geometric sequence?
-The common ratio in a geometric sequence is the constant factor by which each term is multiplied to get the next term.
How does the geometric sequence in the script start?
-The geometric sequence in the script starts with the same first number as the arithmetic sequence, which is 3.
What is the common ratio between the first two terms of the geometric sequence in the script?
-The common ratio between the first two terms of the geometric sequence in the script is 2 (6 ÷ 3 = 2).
How do arithmetic sequences differ graphically from geometric sequences?
-Graphically, arithmetic sequences form a straight line because they increase by a constant amount, while geometric sequences form a curve because they increase by a constant ratio.
What happens to the terms in a geometric sequence as you move from one term to the next?
-In a geometric sequence, each term is obtained by multiplying the previous term by the common ratio.
Why does the geometric sequence have a curved shape when graphed?
-The geometric sequence has a curved shape when graphed because each term is a multiple of the previous term, leading to an exponential increase or decrease.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
MATH 10 : DIFFERENTIATING GEOMETRIC SEQUENCE FROM AN ARITHMETIC SEQUENCE (Taglish)
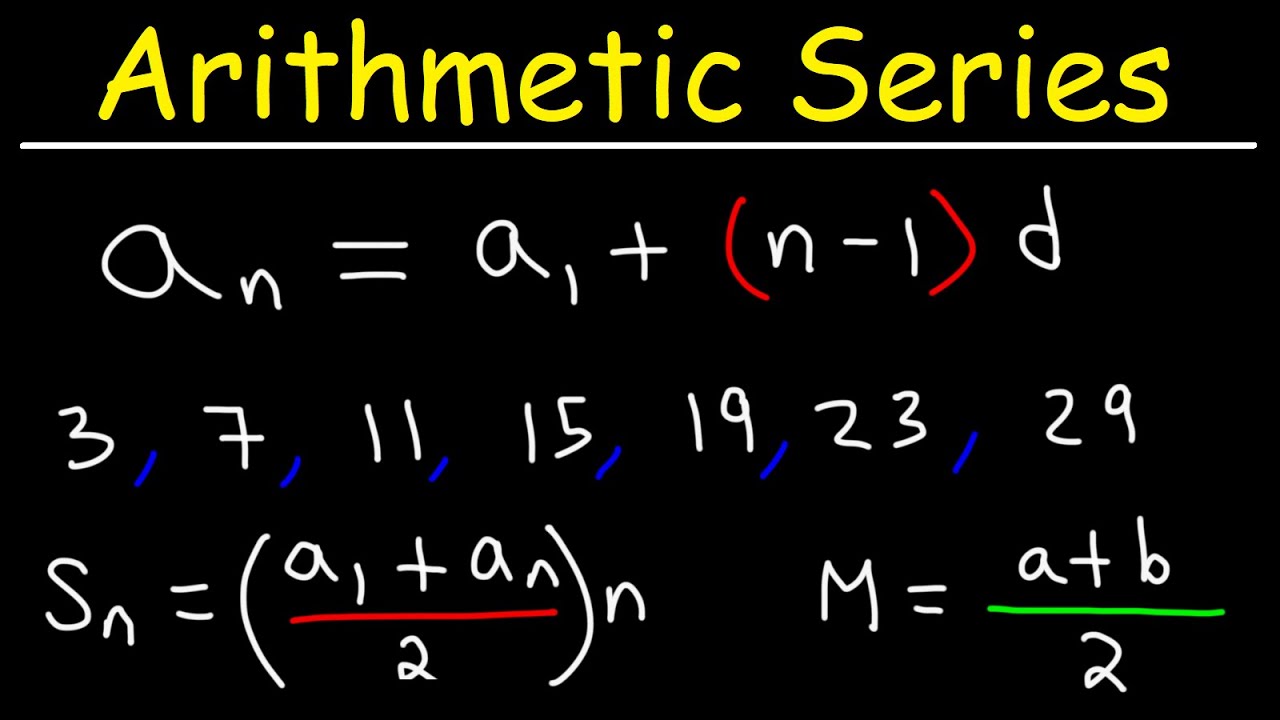
Arithmetic Sequences and Arithmetic Series - Basic Introduction

GCSE Maths - Types of Number Sequences - Arithmetic vs Geometric

Geometric Series and Geometric Sequences - Basic Introduction

Pembahasan BARISAN DAN DERET (Aritmetika & Geometri) KELAS 11 | #MatematikAsik

Barisan dan deret Geometri kelas 10
5.0 / 5 (0 votes)