Writing Equation of a Line

Summary

This educational video script teaches viewers how to derive the equations of lines given specific conditions. It covers various scenarios, including lines passing through certain points, perpendicular bisectors, and lines parallel to others with shared intercepts. The script explains how to calculate slopes, use the point-slope form, and convert equations to general form. Each problem is methodically solved, providing clear steps for finding midpoints, determining slopes of perpendicular lines, and utilizing intercepts to form equations.

Takeaways

• 📚 The video teaches how to derive the equation of a line given certain conditions.
• 📍 To find the equation of a line, one must determine the slope and a point through which it passes.
• 🧭 The midpoint formula is used to find the midpoint of a segment given two points: ((x1 + x2) / 2, (y1 + y2) / 2).
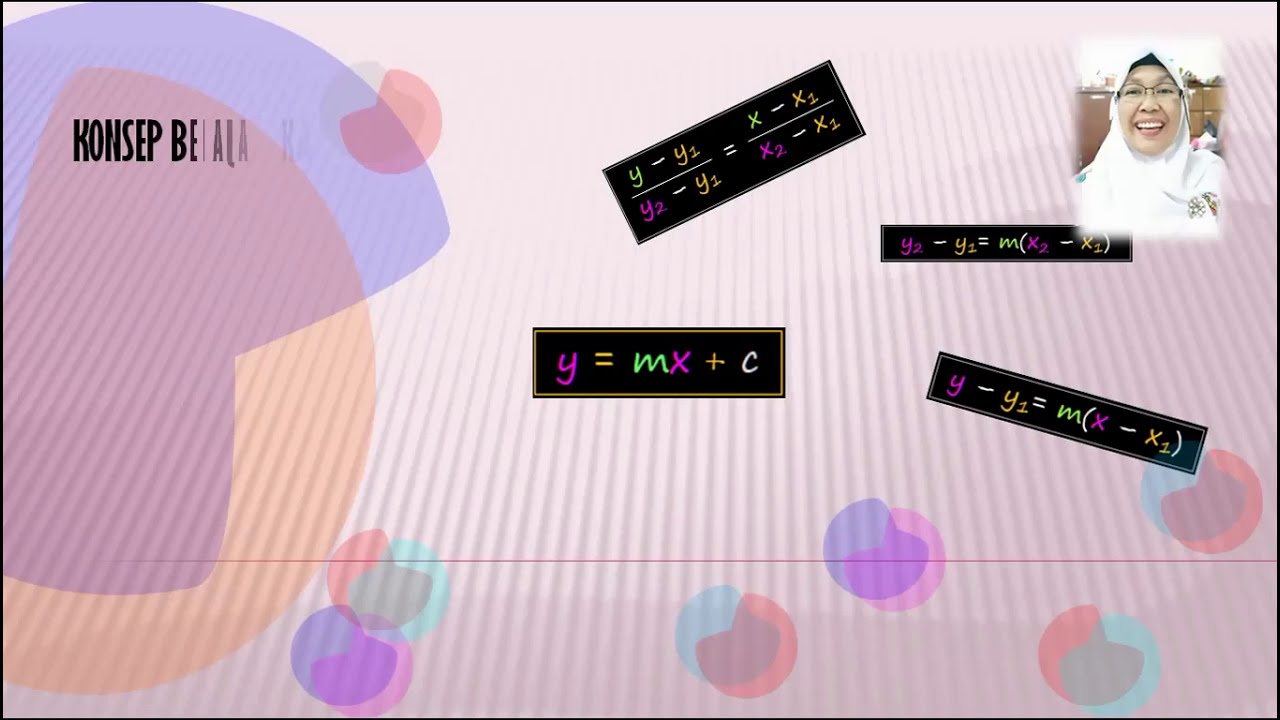
• 🔍 The slope of a line is calculated by (y2 - y1) / (x2 - x1).
• ✍️ The point-slope form of a line's equation is y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.
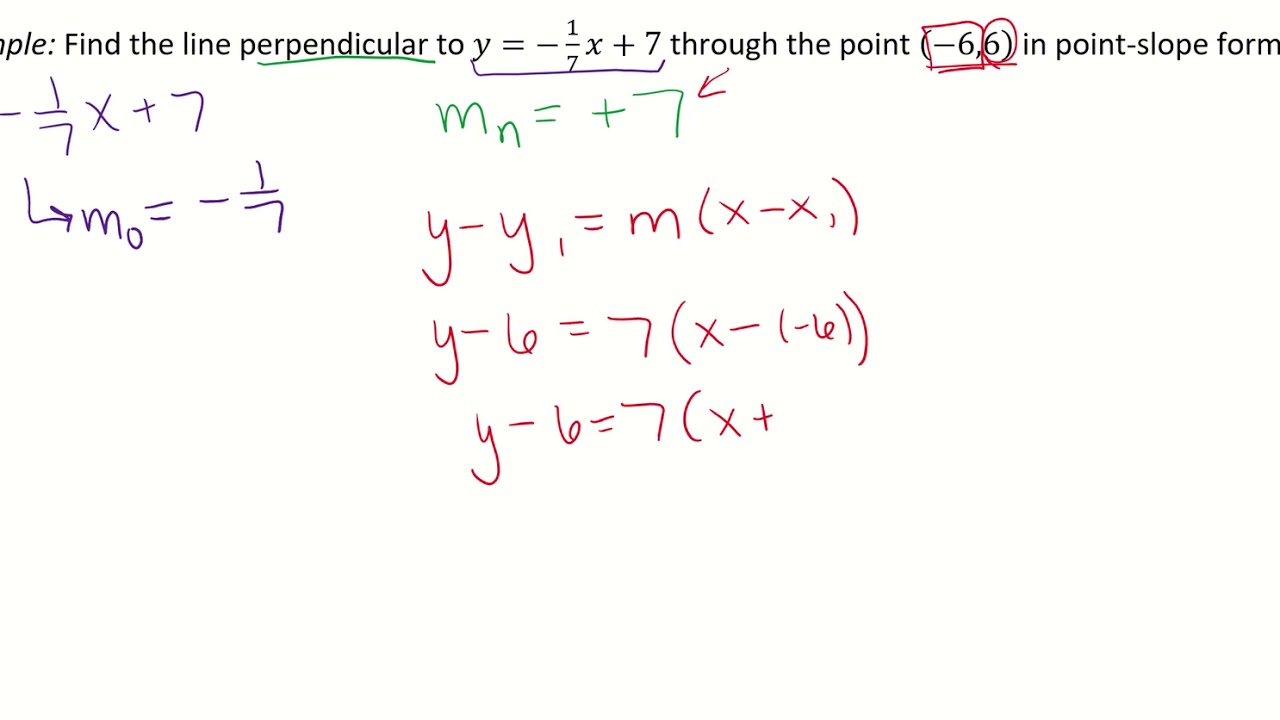
• 🔄 The slope of a line perpendicular to another is the negative reciprocal of the original line's slope.
• 📐 The equation of a perpendicular bisector of a segment is found using the midpoint of the segment and the slope perpendicular to the original line.
• 🔄 For a line parallel to another, the slope remains the same, but the y-intercept may differ.
• 🔢 If a line has intercepts that are numerically equal but opposite in sign, the slope of the line is 1 or -1.
• 📉 To find the x-intercept of a line, set y to 0 in the line's equation and solve for x.
• 📈 The general form of a line's equation is Ax + By + C = 0, which can be derived from point-slope form by rearranging terms.

Q & A

What is the first step in finding the equation of a line that passes through a given point and a midpoint?

The first step is to find the midpoint of the segment joining two given points using the formula (x1 + x2)/2 for the x-coordinate and (y1 + y2)/2 for the y-coordinate.

How do you calculate the slope of a line given two points?

The slope (m) of a line passing through two points (x1, y1) and (x2, y2) is calculated using the formula m = (y2 - y1) / (x2 - x1).

What is the point-slope form of the equation of a line?

The point-slope form of the equation of a line is y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.

How do you find the equation of a line that is perpendicular to another line?

To find the equation of a line perpendicular to another, you first find the slope of the original line, then use the negative reciprocal of that slope for your new line. The new line must also pass through the given point or midpoint.

What is the slope of a line perpendicular to one with a slope of 9?

The slope of a line perpendicular to another with a slope of 9 is the negative reciprocal of 9, which is -1/9.

How do you convert the point-slope form of a line to the general form?

To convert from point-slope form (y - y1 = m(x - x1)) to general form, distribute m across (x - x1), then simplify and rearrange the terms to isolate zero on one side of the equation.

What does it mean for two lines to be parallel?

Two lines are parallel if they have the same slope but never intersect, which means their equations have the same ratio of the coefficients of x and y.

How do you find the x-intercept of a line given its equation?

To find the x-intercept of a line, set y to zero in the equation and solve for x. The point where the line crosses the x-axis is the x-intercept.

What is the relationship between the intercepts of a line with numerically equal but opposite in sign intercepts?

If a line has intercepts that are numerically equal but opposite in sign, it means that if the x-intercept is 'a', the y-intercept is '-a', and vice versa.

How do you find the equation of a line that passes through a given point and has a specific slope?

Use the point-slope form of the equation with the given point and the specific slope. Then, simplify the equation to find its general form.

What is the slope of a line that passes through the points (-2, 1) and (1, 4)?

The slope of a line passing through the points (-2, 1) and (1, 4) is calculated as (4 - 1) / (1 - (-2)) = 3 / 3 = 1.