MATEMATIKA VIII Bab 4 Bagian 2 PERSAMAAN GARIS dengan KEMIRINGAN

Summary

This educational video delves into the concept of linear equations in mathematics, particularly focusing on the slope-intercept form and its applications. The lesson covers key formulas for calculating the slope (gradient) and finding the equation of a line using known coordinates. It includes various examples and problem-solving steps, demonstrating how to derive equations of lines from different types of given data. The video also explores parallel and perpendicular lines, highlighting their unique properties. By the end of the video, viewers gain a clear understanding of how to solve and apply linear equations in real-world contexts.

Takeaways

• 😀 The lesson focuses on solving problems related to the equation of a straight line with a slope.
• 😀 The key learning objective is to enable students to solve problems involving the equation of a line with slope.
• 😀 The script discusses different positions of straight lines, such as those parallel to the x-axis, y-axis, or slanting left or right.
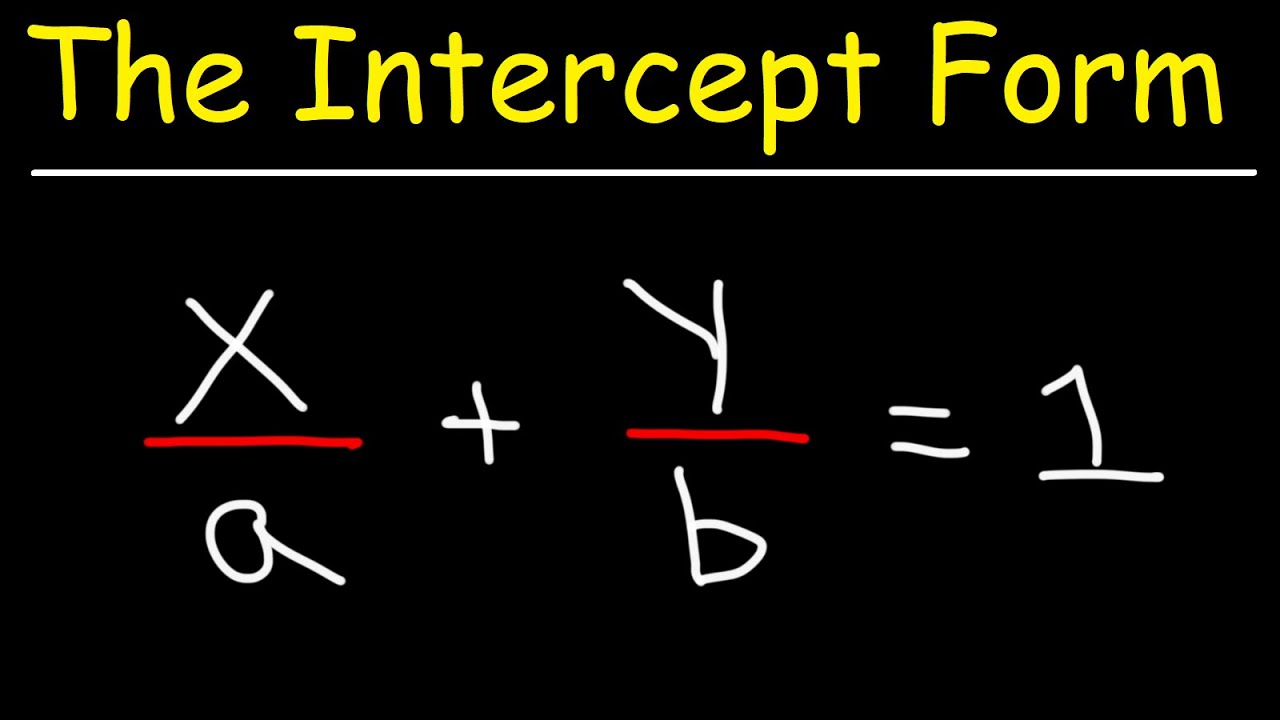
• 😀 The formula for a straight line is introduced as y = MX + C, where 'M' is the slope and 'C' is the y-intercept.
• 😀 The gradient (slope) of a line can be calculated using the formula: (y2 - y1) / (x2 - x1).
• 😀 When a line passes through the origin (0,0), the equation does not have a constant term, and the equation simplifies to y = MX.
• 😀 Examples are given to show how to form equations of lines given the slope and coordinates, such as y = 2x and y = 3x + 3.
• 😀 The script illustrates how to solve equations involving two coordinates using the formula y - y1 = M(x - x1).
• 😀 There are specific examples of equations for lines that are parallel and perpendicular to each other, with slopes demonstrating these relationships.
• 😀 The script concludes by reviewing problems involving lines with given coordinates, helping students understand how to apply the formulas for different types of lines.

Q & A

What is the general form of the equation of a straight line?

The general form of the equation of a straight line is y = MX + C, where 'y' and 'x' are variables, 'M' is the gradient (slope), and 'C' is the y-intercept (constant).

What does the gradient (M) of a straight line represent?

The gradient (M) of a straight line represents the slope or steepness of the line. It indicates how much 'y' changes for a unit change in 'x'.

How do you calculate the gradient (M) of a line given two coordinates?

The gradient (M) of a line through two points (x1, y1) and (x2, y2) is calculated using the formula: M = (y2 - y1) / (x2 - x1).

What happens if the gradient (M) is greater than zero?

If the gradient (M) is greater than zero, the line slopes upwards from left to right, meaning it is facing the right.

What does it mean when the gradient (M) is less than zero?

When the gradient (M) is less than zero, the line slopes downwards from left to right, meaning it is facing the left.

How do you form the equation of a line using a known gradient and a point on the line?

The equation of a line with known gradient (M) and a point (x1, y1) can be formed using the formula: y - y1 = M(x - x1).

How is the equation of a line simplified when the coordinates pass through the origin (0, 0)?

When the coordinates pass through the origin (0, 0), the equation simplifies to y = Mx, as the constant (C) is zero.

How do you determine if two lines are parallel?

Two lines are parallel if they have the same gradient (M). If M1 = M2, the lines are parallel.

How do you determine if two lines are perpendicular?

Two lines are perpendicular if the product of their gradients is -1. That is, M1 * M2 = -1.

Can you give an example of finding the equation of a line given two points (A and B)?

Sure! For example, given two points A(1, -5) and B(-2, 4), you can calculate the gradient M = (4 - (-5)) / (-2 - 1) = 9 / -3 = -3. Then use the formula y - y1 = M(x - x1) to find the equation of the line.