Master Graphing Piecewise Functions
Summary
TLDRThis video tutorial demystifies piecewise functions, showing how they are formed from two or more distinct functions defined over specific intervals. The instructor explains the process of graphing these functions, emphasizing the importance of graphing each function separately before applying constraints. Through clear examples, including linear and quadratic functions, viewers learn to accurately represent these graphs while using open and closed circles to indicate whether endpoints are included. The tutorial concludes with a reminder of the vertical line test, ensuring that the resulting graph is indeed a function.
Takeaways
- 😀 A piecewise function is defined by two or more sub-functions, each applicable to specific intervals of the domain.
- 😀 The notation for piecewise functions clearly indicates which function to use for different ranges of x values.
- 😀 Graphing piecewise functions involves graphing each function separately before combining them according to their constraints.
- 😀 It is important to apply constraints correctly; closed circles indicate included values, while open circles indicate excluded values.
- 😀 Understanding the basic shapes of functions (like lines and parabolas) is essential for graphing piecewise functions.
- 😀 For linear functions, the slope-intercept form (y = mx + b) can help in determining points to graph.
- 😀 Quadratic functions can be stretched or shifted based on their coefficients and constants.
- 😀 Using technology or graphing calculators can help verify the results of hand-drawn graphs.
- 😀 Always check that the final graph passes the vertical line test to confirm it represents a valid function.
- 😀 Practice with various types of piecewise functions helps reinforce understanding of how to graph them correctly.
Q & A
What is a piecewise function?
-A piecewise function is a function composed of two or more sub-functions, each defined over specific intervals of the input variable.
How are constraints used in piecewise functions?
-Constraints are inequalities that specify the intervals for which each sub-function is valid. They determine which parts of the function to graph based on the input values.
What is the first step in graphing a piecewise function?
-The first step is to graph each sub-function separately without considering the constraints. This helps to visualize their shapes and behaviors.
What does the notation 'f(x) = 1/2 x for x ≤ 0' imply?
-This notation indicates that the function f(x) equals 1/2 x only for values of x that are less than or equal to zero.
What is the significance of open and closed circles in graphing?
-An open circle indicates that a point is not included in the function (e.g., x > 0), while a closed circle indicates that the point is included (e.g., x ≤ 0).
What is the vertical line test?
-The vertical line test is a method to determine if a graph represents a function. If any vertical line crosses the graph more than once, it does not represent a function.
How do you graph a function defined as 'y = 3' for x > 0?
-To graph 'y = 3' for x > 0, draw a horizontal line at y = 3 starting from the point (0, 3) with an open circle, indicating that the point (0, 3) is not included.
What does it mean to combine the graphs of piecewise functions?
-Combining the graphs involves overlaying the individual graphs of each sub-function while applying their respective constraints and erasing any parts that don't satisfy the conditions.
What types of functions were used as examples in the tutorial?
-The tutorial used linear functions and quadratic functions as examples of piecewise functions.
Why is it important to understand the parent function when graphing quadratics?
-Understanding the parent function helps in identifying key features of the quadratic, such as its vertex and the direction of its opening, which are essential for accurate graphing.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

FUNGSI PIECEWISE || PRESENTASI KELOMPOK 5 XI 13-SMASTA

PIECEWISE FUNCTIONS || GRADE 11 GENERAL MATHEMATICS Q1
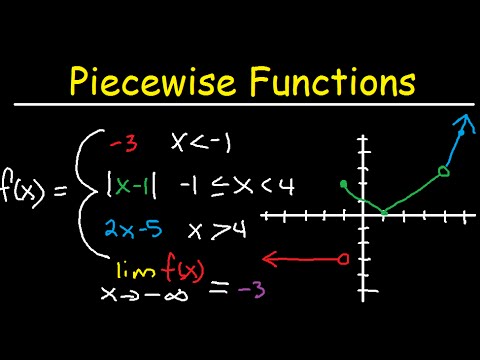
Graphing Piecewise Functions, Domain & Range - Limits, Continuity, & Absolute Value ,

A Tale of Three Functions | Intro to Limits Part I
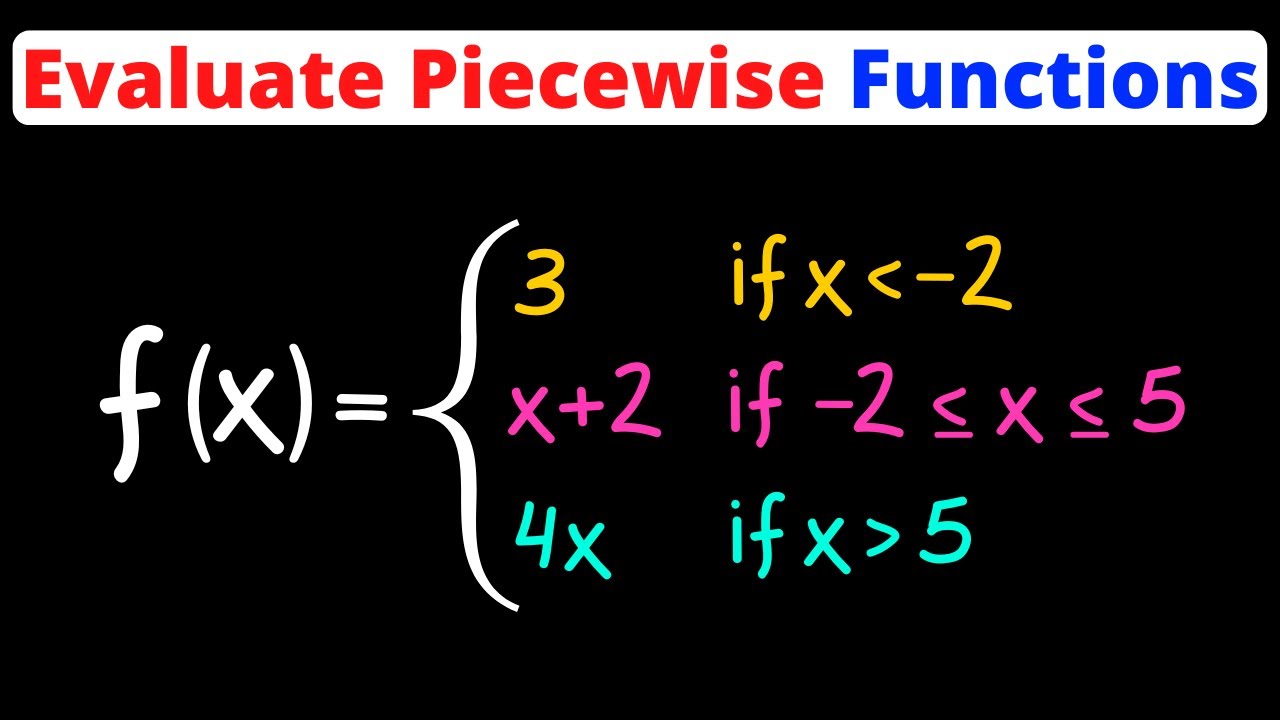
Evaluate a Piecewise Function | Eat Pi

Functions in C programming in Hindi 01 | Type of function | Library & User defined function
5.0 / 5 (0 votes)
