FUNGSI PIECEWISE || PRESENTASI KELOMPOK 5 XI 13-SMASTA
Summary
TLDRThis video explains the concept of piecewise functions, which are defined by multiple expressions depending on the value of the input variable. The script covers how to graph piecewise functions, the importance of intervals, and how the function changes based on conditions. Real-world applications, such as taxi fares, progressive tax rates, and shipping costs, are also explored, demonstrating how piecewise functions model scenarios where rates or rules vary. The video concludes with an example of calculating a taxi fare for a 10 km journey, highlighting the practical use of piecewise functions in everyday problems.
Takeaways
- 😀 Piecewise functions are mathematical functions that are defined by different formulas over specific intervals of their domain.
- 😀 Piecewise functions are useful for modeling complex phenomena that do not follow simple mathematical patterns, such as sudden changes or transitions.
- 😀 Piecewise functions are commonly used in fields like mathematics, economics, social sciences, and engineering to model real-world scenarios.
- 😀 A piecewise function can have multiple sub-functions, each applicable to a specific range of values (domain).
- 😀 The general notation for piecewise functions includes curly braces, with each sub-function indicated for a specific domain interval.
- 😀 An important feature of piecewise functions is the possibility of discontinuities in their graphs, where the function value jumps abruptly.
- 😀 To graph a piecewise function, one must first create a table with X and Y values for each segment, then plot the points on a coordinate plane.
- 😀 The process of graphing a piecewise function includes determining the function's values at boundary points and some random test points, then connecting the points appropriately.
- 😀 In piecewise functions, closed circles (filled) indicate that the value is included at a specific point, while open circles (hollow) indicate that the value is excluded from that interval.
- 😀 Real-life examples of piecewise functions include taxi fare calculations, progressive tax rates, shipping costs, discount structures in stores, and subscription packages for streaming services.
Q & A
What is a piecewise function?
-A piecewise function is a mathematical function that is defined by different formulas over different intervals of its domain. Each part of the domain has its own rule or formula.
Why are piecewise functions important in mathematics?
-Piecewise functions are important because they allow for modeling situations that involve different behaviors depending on conditions. This flexibility makes them useful in both basic mathematics and complex real-world phenomena.
Can you provide an example of a piecewise function?
-Yes. An example of a piecewise function is: f(x) = 2x + 1 if x ≤ 2, and f(x) = x - 4 if x > 2. This function uses different rules for different values of x.
How do you graph a piecewise function?
-To graph a piecewise function, create a table of values for each piece, substitute x-values into the appropriate formulas, plot the points, and connect them while keeping in mind where the function may have discontinuities or jumps.
What is meant by a discontinuity in a piecewise function?
-A discontinuity in a piecewise function refers to a jump or break in the graph where the function does not transition smoothly between intervals. This can occur when the function has different values on either side of a point.
How do you handle points of discontinuity when graphing a piecewise function?
-When graphing a piecewise function, points of discontinuity are often marked with open or closed circles to indicate whether the function includes or excludes certain values at the boundary of the intervals.
What are some real-life applications of piecewise functions?
-Piecewise functions are commonly used to model real-world scenarios such as taxi fares (base fee + additional charge per distance), progressive tax rates (different tax brackets), and shipping costs (depending on weight or distance).
How is the taxi fare example in the transcript modeled using a piecewise function?
-The taxi fare is modeled by a piecewise function where the base fee is 5000 IDR for the first 2 kilometers, and for each kilometer beyond that, the cost is 3000 IDR per kilometer.
What is the purpose of using a piecewise function to model the taxi fare?
-The purpose of using a piecewise function to model the taxi fare is to account for the different rates that apply based on the distance traveled, with one rate for the initial distance and another for any additional distance.
How do you calculate the taxi fare for a 10 km journey based on the piecewise function?
-For a 10 km journey, the taxi fare is calculated by using the second part of the piecewise function. The cost for the first 2 km is 5000 IDR, and the remaining 8 km is charged at 3000 IDR per km, giving a total of 29000 IDR.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
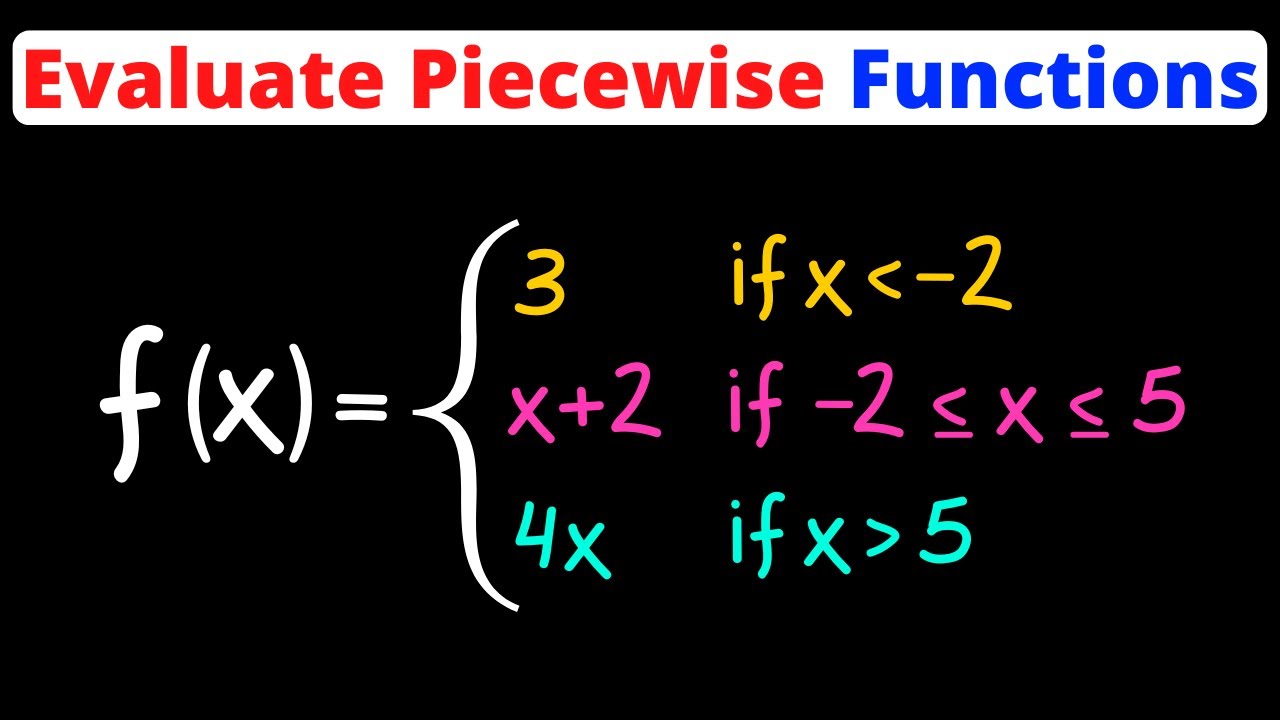
Evaluate a Piecewise Function | Eat Pi

PIECEWISE FUNCTIONS || GRADE 11 GENERAL MATHEMATICS Q1
Integral Lipat | Integral Lipat (Part 1) | Kalkulus

Capítulo 4 - Funções de usuário: Parâmetros

A Tale of Three Functions | Intro to Limits Part I

3 Step CONTINUITY Test | Piecewise Functions | Differential Calculus | Tagalog/Filipino
5.0 / 5 (0 votes)