Writing Equations of Parallel and Perpendicular Lines
Summary
TLDRIn this lesson, the focus is on writing equations for parallel and perpendicular lines in slope-intercept form. The instructor explains how parallel lines have the same slope, demonstrating this with examples, and introduces point-slope form for calculating the equations of lines passing through specific points. The concept of perpendicular lines as having slopes that are opposite reciprocals is also covered, with a detailed walkthrough of transforming standard form equations to slope-intercept form. Each step is clearly illustrated to aid understanding, making it an essential guide for students learning these fundamental concepts in algebra.
Takeaways
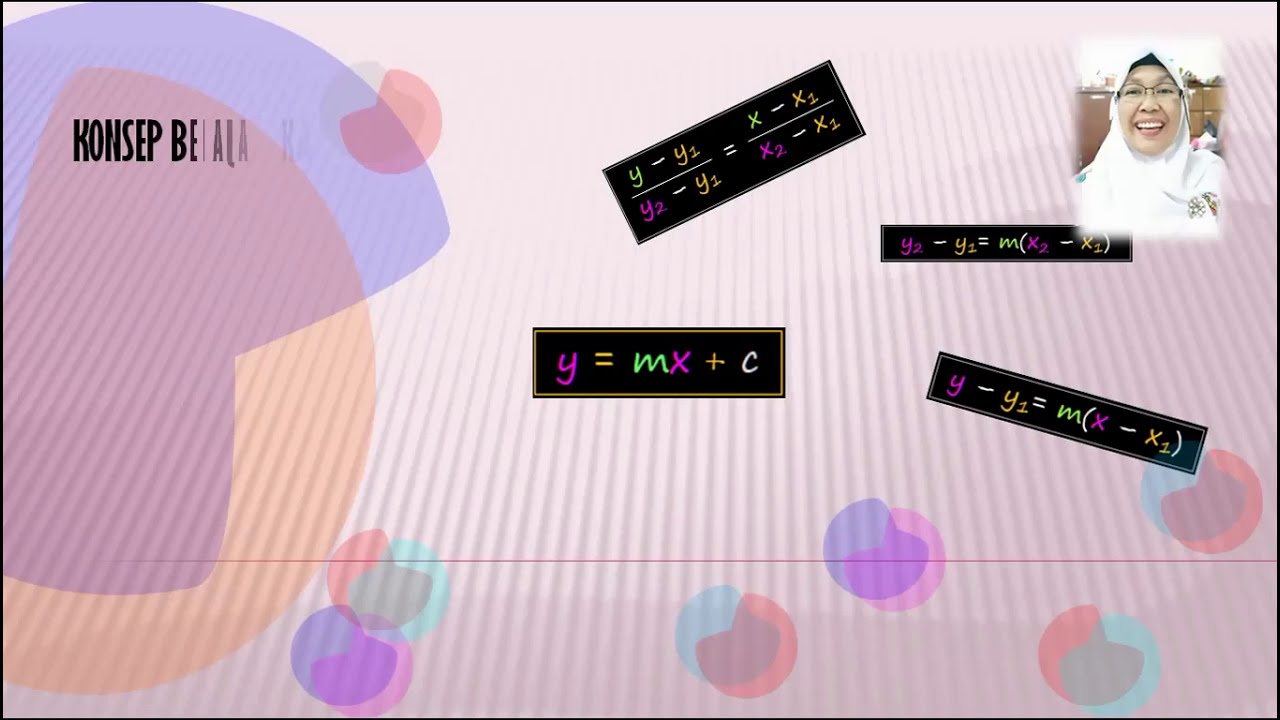
- 😀 Parallel lines have the same slope, which means they never intersect.
- 😀 To write the equation of a parallel line, use the point-slope form with the same slope as the original line.
- 😀 The point-slope formula is Y - y1 = m(X - x1), where (x1, y1) is a point on the line.
- 😀 When converting from standard form to slope-intercept form, isolate y to find the slope.
- 😀 Perpendicular lines have slopes that are opposite reciprocals of each other.
- 😀 The slope of a line in standard form Ax + By = C can be found using the formula -A/B.
- 😀 To write the equation of a perpendicular line, find the opposite reciprocal of the original slope.
- 😀 Simplifying the equation often involves distributing and combining like terms.
- 😀 Practice using various points and slopes to gain mastery over writing line equations.
- 😀 Understanding these concepts is essential for success in algebra and geometry.
Q & A
What is the primary focus of the video transcript?
-The video focuses on writing equations of parallel and perpendicular lines in slope-intercept form.
What do parallel lines have in common regarding their slopes?
-Parallel lines have the same slope.
How do you find the slope of a line given in standard form?
-To find the slope from standard form (Ax + By = C), you can convert it to slope-intercept form (y = mx + b) or use the formula -A/B, where A and B are coefficients of x and y.
What is the point-slope formula used in the video?
-The point-slope formula is given by Y - y1 = m(X - x1), where (x1, y1) is a point on the line and m is the slope.
How do you determine the slope of a line that is perpendicular to another line?
-The slope of a line perpendicular to another is the opposite reciprocal of the original line's slope.
What transformation was done to convert the equation to slope-intercept form?
-The process involves isolating y on one side of the equation by moving terms and simplifying.
What are the steps to write the equation of a line parallel to a given line through a specific point?
-1) Identify the slope of the given line. 2) Use the point-slope form with the given point and identified slope. 3) Simplify to slope-intercept form.
In the example, what is the slope of the line given by the equation y = 3x + 2?
-The slope of the line y = 3x + 2 is 3.
What was the calculated slope of the line that is perpendicular to y = 1/2x + 3?
-The slope of the line that is perpendicular to y = 1/2x + 3 is -2.
What key concept does the example with the equation 2x + 4y = 8 illustrate?
-It illustrates converting from standard form to slope-intercept form to find the slope and write an equation of a perpendicular line.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenant5.0 / 5 (0 votes)