Simplifying Rational Expressions... How? (NancyPi)
Summary
TLDRIn this instructional video, Nancy explains how to simplify rational expressions by factoring both the numerator and denominator, canceling common factors, and noting any restrictions to avoid division by zero. She covers examples, emphasizing the importance of identifying conditions that restrict variable values, particularly when factors are canceled. Nancy provides insights into factoring quadratics and dealing with larger coefficients, while also reminding viewers about maintaining awareness of restrictions throughout the simplification process. This comprehensive guide aims to demystify rational expressions and enhance viewers' understanding of the topic.
Takeaways
- 😀 A rational expression is a fraction with a numerator and denominator that can be simplified by factoring.
- 🛠️ To simplify, first factor the numerator and denominator as much as possible.
- 🔍 Always check if you can factor out any common numbers or variables before proceeding.
- 📉 Use the difference of squares formula for expressions like x^2 minus a perfect square.
- ✂️ After factoring, cancel out any common terms in the numerator and denominator.
- 🚫 When canceling factors, note that any value that makes the denominator zero is a restriction.
- ⚠️ It's important to write conditions for any restrictions after simplifying the expression.
- 📊 For quadratic expressions, look for two numbers that multiply to the constant term and add to the linear coefficient.
- ❌ Only cancel factors, not individual terms; ensure you understand the structure of the expressions.
- 💡 If you're asked to state the domain, write down all values that would make the denominator zero as restrictions.
Q & A
What is a rational expression?
-A rational expression is a fraction that consists of a numerator and a denominator, where both are polynomials.
What is the first step to simplifying a rational expression?
-The first step is to factor the numerator and denominator as much as possible.
What is the difference of squares formula?
-The difference of squares formula states that a^2 - b^2 can be factored into (a + b)(a - b).
Why is it important to identify restrictions when simplifying?
-Identifying restrictions is crucial because it ensures you know the values that would make the denominator zero, which would result in undefined expressions.
How do you factor x^2 - 49?
-x^2 - 49 can be factored using the difference of squares formula into (x + 7)(x - 7).
What happens if you cancel out a factor that leads to division by zero?
-Cancelling such a factor can create an incorrect solution, so it's important to note that the value leading to zero in the denominator remains a restriction.
How do you determine restrictions after simplification?
-To determine restrictions, identify the values that would make any cancelled factors in the denominator equal to zero.
What is a common mistake when simplifying expressions?
-A common mistake is attempting to cancel individual terms instead of entire factors, which is not mathematically valid.
When factoring quadratics, what are you looking for?
-You need to find two numbers that multiply to the constant term and add to the coefficient of the linear term.
What should you do if you're unsure how to factor an expression?
-If you're unsure how to factor, it's advisable to seek additional resources, such as instructional videos on factoring techniques.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

MULTIPLICATION AND DIVISION OF RATIONAL ALGEBRAIC EXPRESSIONS || GRADE 8 MATHEMATICS Q1

SIMPLIFYING RATIONAL ALGEBRAIC EXPRESSION || GRADE 8 MATHEMATICS Q1
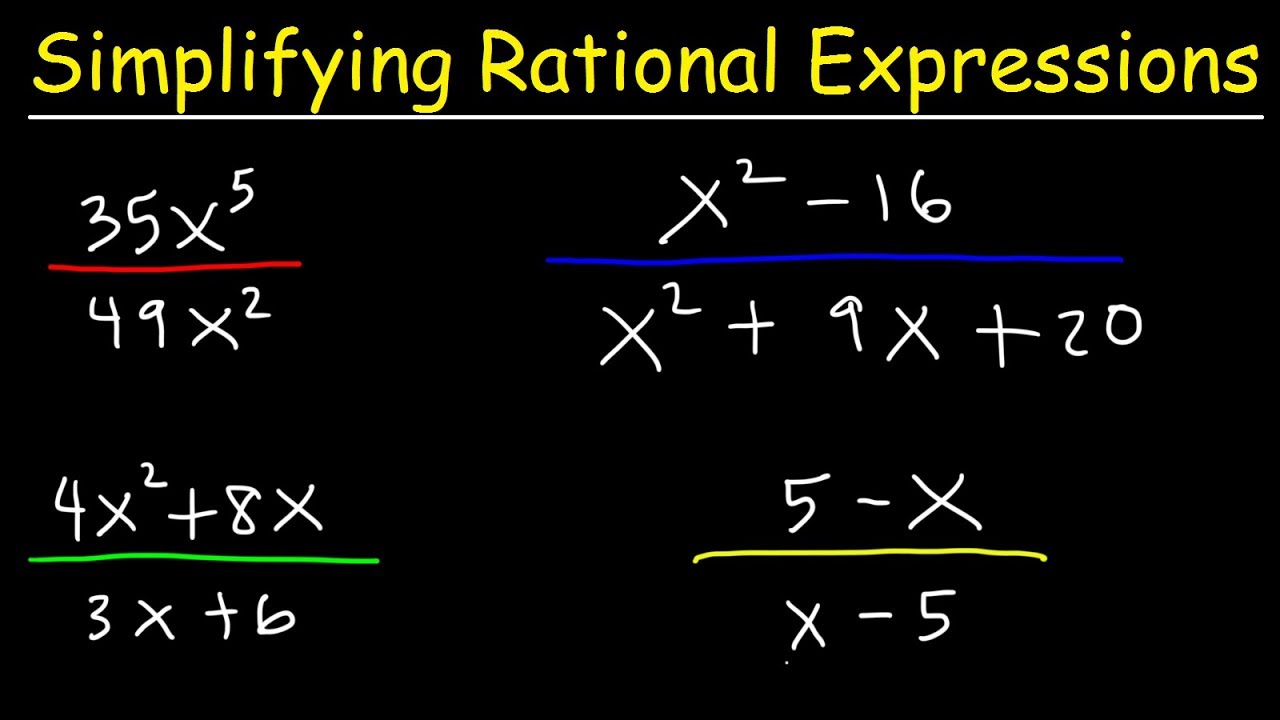
Simplifying Rational Expressions

Adding and Subtracting Rational Algebraic Expressions Part 2 - Grade 8 Mathematics

Solving Rational Equations (Easy Method)
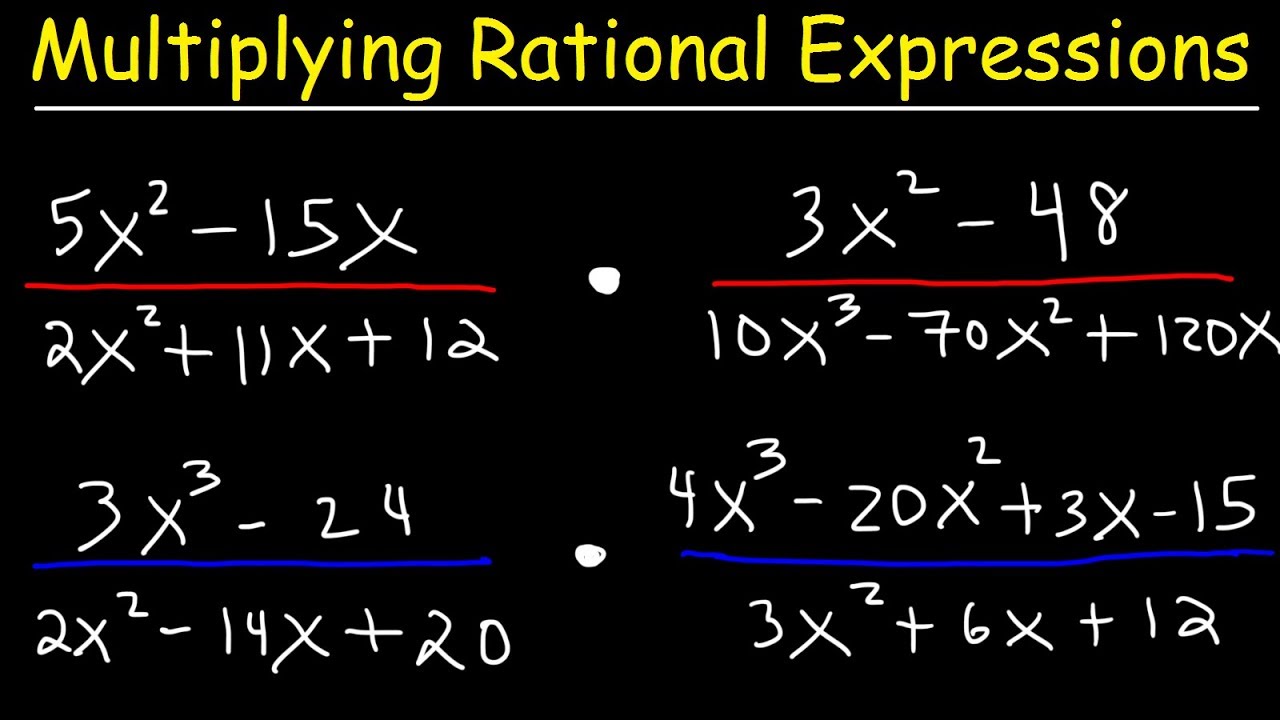
Multiplying Rational Expressions
5.0 / 5 (0 votes)
