SIMPLIFYING RATIONAL ALGEBRAIC EXPRESSION || GRADE 8 MATHEMATICS Q1
Summary
TLDRThis educational video delves into the world of rational algebraic expressions, explaining their definition as a rational expression in one variable, typically in the form of p/q where p and q are polynomials. It emphasizes the importance of the denominator not being zero to avoid undefined expressions. The concept of domain is introduced as the set of permissible variable values, with examples illustrating how to determine it by setting the denominator to zero and solving for the variable. The video also guides viewers on simplifying rational expressions by finding the greatest common factor (GCF) or by canceling common factors, using clear examples to demonstrate the process. The tutorial is designed to enhance understanding and simplify complex mathematical concepts.
Takeaways
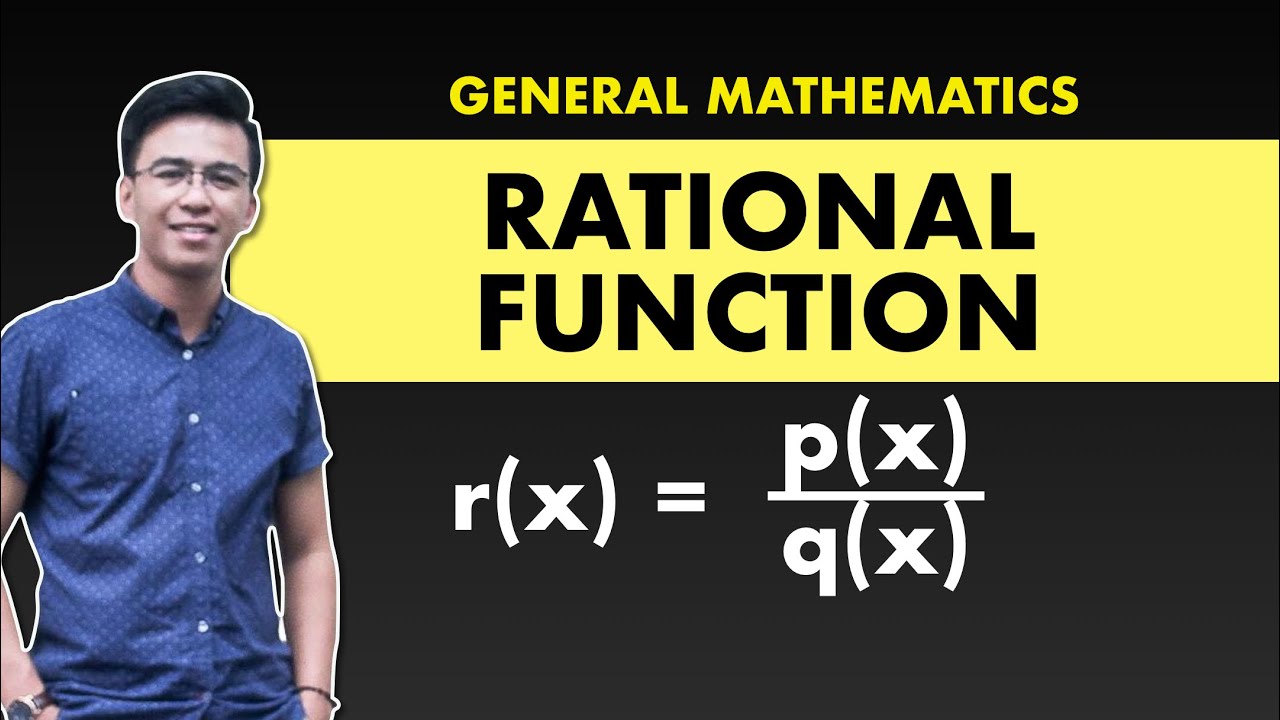
- 📘 A rational algebraic expression is a rational expression in one variable, written in the form p/q, where p and q are polynomials.
- 🚫 The denominator (q) must not be equal to zero, as this would make the rational algebraic expression undefined.
- 🔍 The domain of a rational algebraic expression is the set of all possible values for the variable that do not make the expression undefined.
- 📌 To find the values that make a rational expression undefined, set the denominator equal to zero and solve for the variable.
- 🔢 Examples given include simplifying expressions like 4/(x-2), 4x/(x^2+9), and (x^2+2x-35)/3x+4 by factoring and canceling common factors.
- 🔄 The process of simplifying rational algebraic expressions involves finding the lowest terms and using the greatest common factor (GCF) where possible.
- ✂️ When simplifying, common factors in the numerator and denominator are canceled out to reduce the expression to its simplest form.
- 📉 The video provides a step-by-step guide on how to simplify expressions by factoring and canceling, including examples with variables and coefficients.
- 📚 The tutorial also covers how to handle expressions with multiple variables and how to simplify by subtracting the exponents of like bases.
- 💡 The video concludes with a reminder to practice these skills and encourages viewers to engage with the channel for more math tutorial videos.
Q & A
What is a rational algebraic expression?
-A rational algebraic expression is a rational expression in one variable that can be written in the form p over q, where p and q are polynomials.
Why is it important that the denominator q in a rational algebraic expression is not equal to zero?
-The denominator q must not be equal to zero because it would make the rational algebraic expression undefined.
What is the domain of a rational algebraic expression?
-The domain is the set of all possible values of the variable that are allowed, excluding values that make the expression undefined.
How do you determine the restricted values in the domain of a rational expression?
-Restricted values are determined by setting the denominator equal to zero and solving for the variable, as these values would make the expression undefined.
Can you provide an example of how to find the domain of a rational expression with the denominator x - 4?
-To find the domain, set the denominator x - 4 equal to zero and solve for x, which gives x = 4. Thus, the domain is all real numbers except x = 4.
How do you simplify a rational algebraic expression?
-Simplifying a rational algebraic expression involves factoring the numerator and the denominator and then canceling out or dividing the common factors.
What is the greatest common factor (GCF) and how is it used in simplifying rational expressions?
-The GCF is the largest factor that divides both the numerator and the denominator. It is used to simplify rational expressions by dividing both the numerator and the denominator by the GCF.
Can you give an example of simplifying a rational expression with a quadratic numerator and a linear denominator?
-An example is x^2 - 6x + 8 over 4x - 8. Factoring gives (x - 4)(x - 2) over 4(x - 2). The common factor (x - 2) can be canceled out, simplifying to x - 4 over 4.
What is the difference between canceling out common factors and subtracting exponents when simplifying?
-Canceling out common factors involves dividing both the numerator and the denominator by a common factor. Subtracting exponents is used when the base is the same and involves reducing the exponent in the numerator by the exponent in the denominator.
How do you simplify an expression with variables raised to different powers, such as 3x^2y^4 over 9x^3y^2?
-You simplify by canceling out the common factors. For 3x^2y^4 over 9x^3y^2, the GCF of the coefficients is 3, and you subtract the exponents of like bases: x^(2-3) and y^(4-2), resulting in x^(-1)y^2 or 1/x^2y^2.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade Now5.0 / 5 (0 votes)