Cara Mudah Menentukan Kuartil Bawah dan Atas Pada HISTOGRAM
Summary
TLDRThis tutorial provides a step-by-step guide on how to calculate the lower and upper quartiles (Q1 and Q3) using a histogram. The presenter explains how to interpret histogram data, convert it into a frequency distribution table, and calculate cumulative frequencies. Using these calculations, the tutorial demonstrates how to find Q1 and Q3 using specific formulas. The session also covers how to identify the classes where these quartiles lie, with detailed explanations on the formulas and their components. The tutorial aims to help viewers understand the process and apply it to real-life data analysis scenarios.
Takeaways
- 😀 A histogram shows the distribution of data values, with class boundaries marking the intervals between data points.
- 😀 To calculate quartiles, you first need to convert the histogram data into a frequency distribution table, which lists class intervals and their corresponding frequencies.
- 😀 Cumulative frequency is calculated by adding up the frequencies progressively from the bottom of the table to the top.
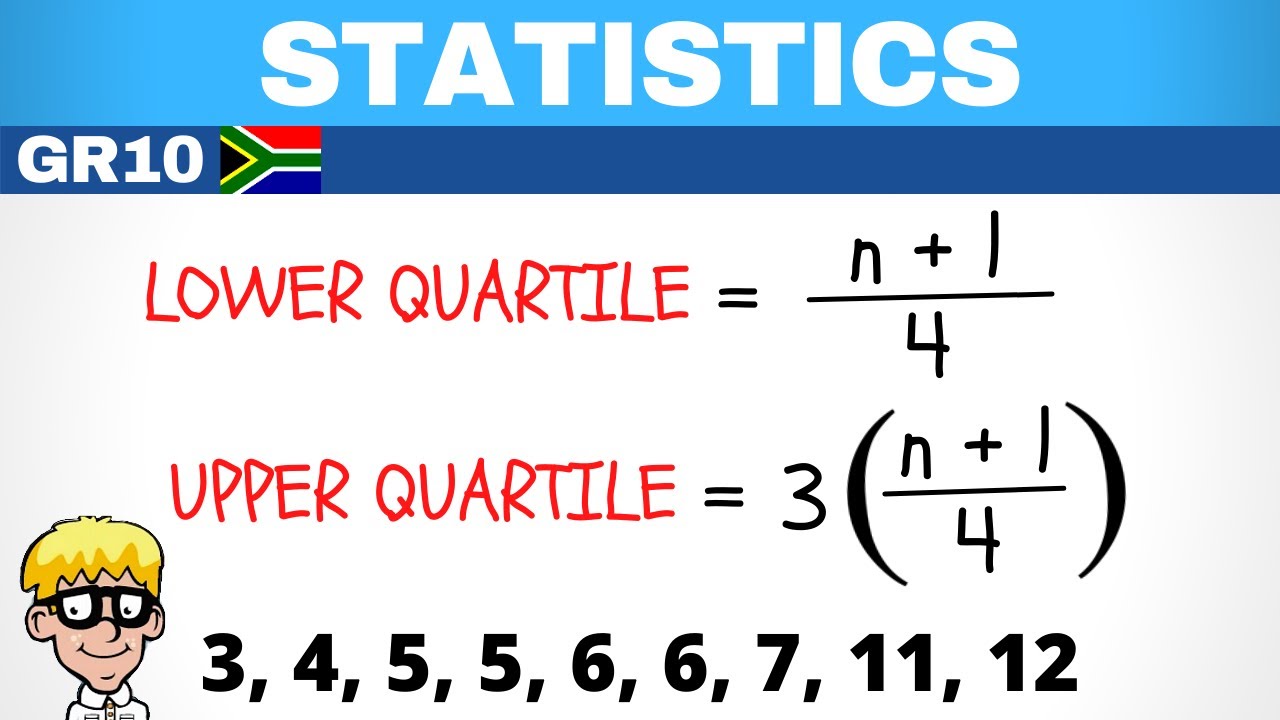
- 😀 The lower quartile (Q1) is the value that separates the first 25% of the data, while the upper quartile (Q3) separates the top 25%.
- 😀 To find Q1, use the formula: Q1 = L + [(N/4 - F) / f] * C, where L is the lower boundary of the Q1 class, N is the total frequency, F is the cumulative frequency before Q1, f is the frequency of the Q1 class, and C is the class width.
- 😀 For Q1, the calculated result should be interpreted as the value that lies at the 17.5th position in a sorted list of data points.
- 😀 The upper quartile (Q3) is calculated using a similar formula: Q3 = L + [(3N/4 - F) / f] * C, where L is the lower boundary of the Q3 class and other variables follow the same logic as Q1.
- 😀 The formula for Q3 helps determine the data point that is at the 52.5th position in the sorted data set.
- 😀 Class boundaries must be carefully identified and adjusted when working with grouped data in histograms, ensuring accurate calculation of quartiles.
- 😀 Both Q1 and Q3 can be interpreted as key data points that provide insight into the distribution and spread of the data, helping in statistical analysis and decision-making.
Q & A
What is the purpose of this tutorial?
-The purpose of the tutorial is to explain how to calculate the lower quartile (Q1) and upper quartile (Q3) from a histogram using a frequency distribution table and cumulative frequencies.
What is the first step in determining the quartiles from the histogram?
-The first step is to convert the histogram into a frequency distribution table, where each class interval is paired with its corresponding frequency.
How do you calculate the cumulative frequency?
-Cumulative frequency is calculated by adding the frequencies sequentially from the first class interval to the last. Each cumulative frequency represents the total number of data points up to that class interval.
What does the value 17.5 represent when calculating Q1?
-The value 17.5 represents the position of Q1 in the ordered dataset, which corresponds to 25% of the total data points (since Q1 divides the lowest 25%).
How is the class interval for Q1 identified?
-The class interval containing Q1 is identified by finding the cumulative frequency that first exceeds the value of 17.5. From the cumulative frequency table, Q1 is located in the class interval 60-63.
What formula is used to calculate Q1?
-The formula to calculate Q1 is: Q1 = L + [(N/4 - F) / f] * h, where L is the lower class boundary of the Q1 class, N is the total number of data points, F is the cumulative frequency before Q1's class, f is the frequency of the Q1 class, and h is the class width.
Why is the class width (h) necessary for calculating the quartiles?
-The class width (h) is necessary because it allows you to scale the cumulative frequency difference to the correct interval size when calculating the precise position of Q1 or Q3 within the class interval.
What does the value 52.5 represent when calculating Q3?
-The value 52.5 represents the position of Q3 in the ordered dataset, which corresponds to 75% of the total data points (since Q3 divides the lowest 75% of the data).
How is the class interval for Q3 identified?
-The class interval containing Q3 is identified by finding the cumulative frequency that first exceeds the value of 52.5. From the cumulative frequency table, Q3 is located in the class interval 68-70.
What is the formula for calculating Q3, and how is it applied in this tutorial?
-The formula for calculating Q3 is: Q3 = L + [(3N/4 - F) / f] * h, where L is the lower class boundary of the Q3 class, N is the total number of data points, F is the cumulative frequency before Q3's class, f is the frequency of the Q3 class, and h is the class width. In this tutorial, Q3 is calculated using this formula with the values for the class interval 68-70.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

Quartil (Data Tunggal dan Kelompok)

Cara Menghitung Kuartil Data Tunggal dan Data Kelompok #STATISTIKA

Perbedaan Jangkauan, Jangkauan Interkuartil dan Kuartil pada Statistika

Kuartil atas dari histogram tersebut adalah ....
Statistics Grade 10: Quartiles

Menentukan Kuartil Q1 Q2 Q3 Median Mean Modus Statistika kelas 12
5.0 / 5 (0 votes)
