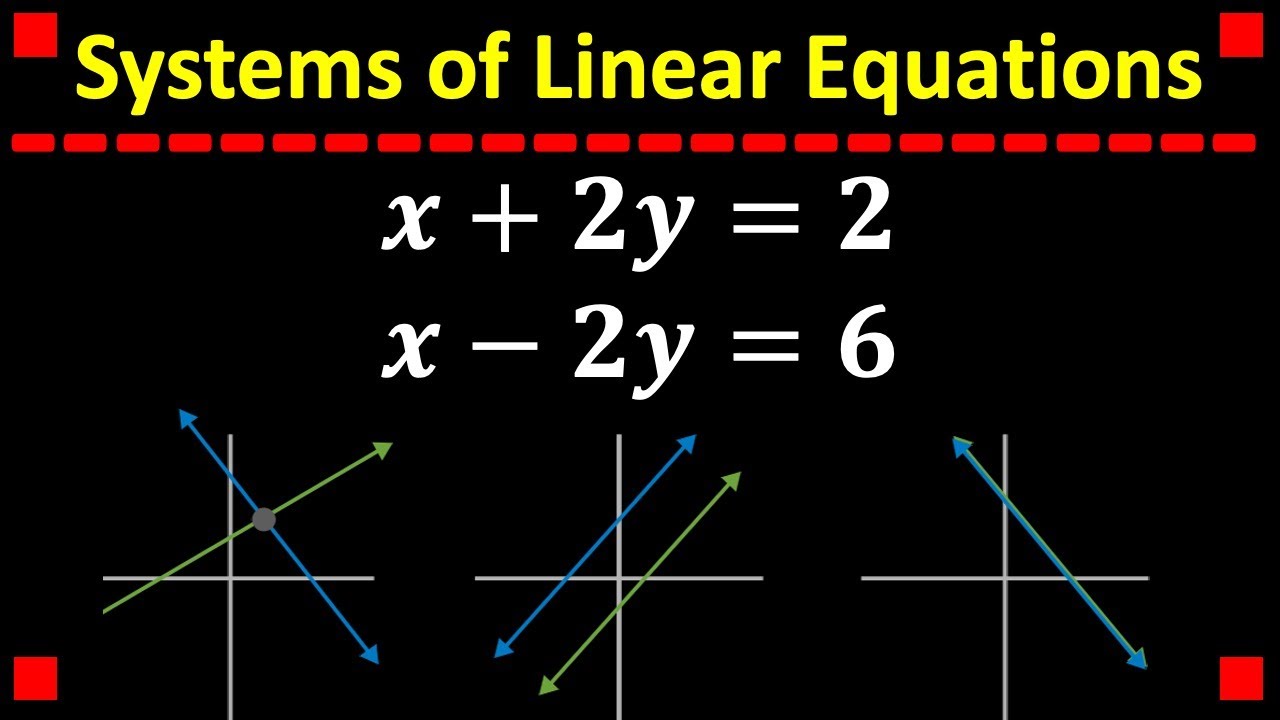
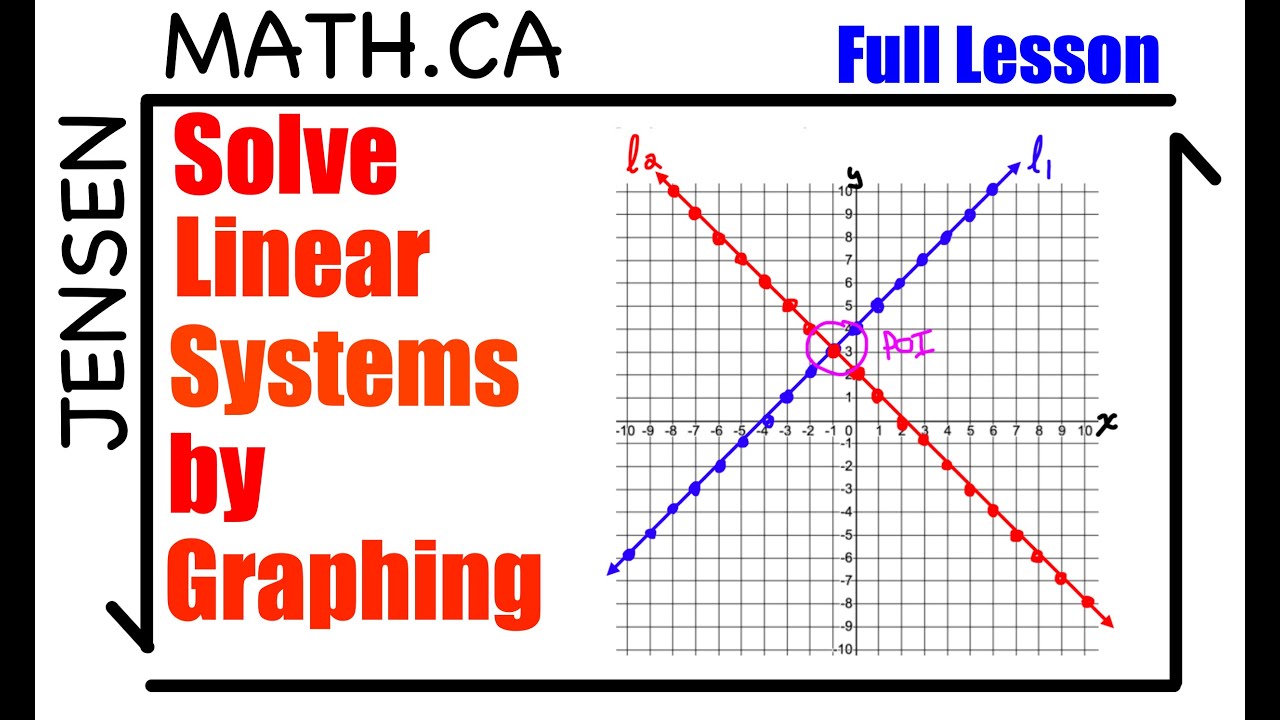
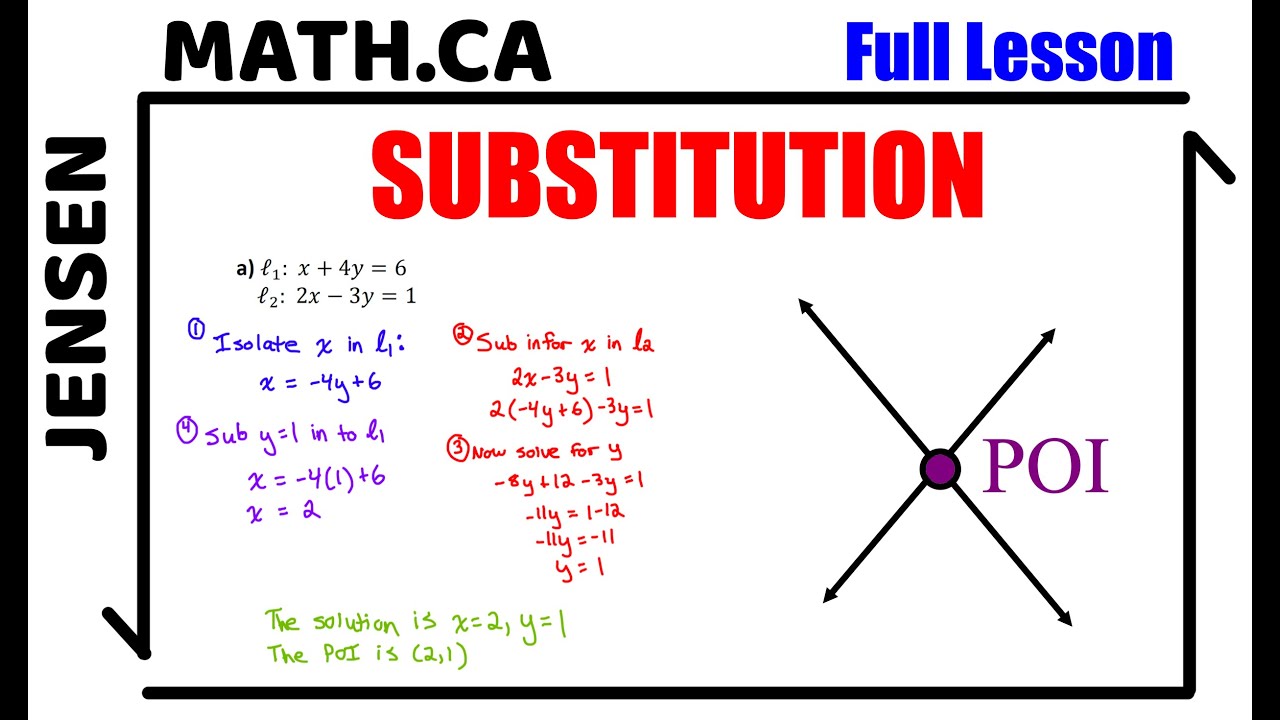
Número de soluciones a ecuaciones lineales
Summary
TLDRThe video explains how to determine the number of solutions for equations, emphasizing three outcomes: one solution, no solutions, or infinitely many solutions. It illustrates these concepts through specific examples, showing how manipulating equations can lead to each scenario. A single solution occurs when a specific value of the variable is found, no solution arises from contradictions, and infinitely many solutions result from true identities. By breaking down the process, the video equips viewers with the tools to analyze equations effectively.
Takeaways
- 😀 A solution exists when you can isolate a variable and obtain a specific value.
- 😀 If simplifying leads to a contradiction, such as 3 = 5, the equation has no solution.
- 😀 Equations can have infinitely many solutions if they reduce to a true identity, like x = x.
- 😀 The first equation resulted in an identity, indicating infinite solutions.
- 😀 The second equation led to a contradiction, confirming there is no solution.
- 😀 The third equation provided a specific value for x, showing it has exactly one solution.
- 😀 Manipulating equations with valid operations is essential for finding solutions.
- 😀 Always check the final form of an equation to determine the type of solution it yields.
- 😀 An equation with the form a = a (like 13 = 13) indicates infinitely many solutions.
- 😀 Understanding these principles helps in effectively solving linear equations.
Q & A
What are the three types of solutions for an equation?
-An equation can have one solution, infinitely many solutions, or no solution.
How do you identify an equation with one solution?
-You identify it when solving results in a specific value for the variable, such as x = a.
What indicates that an equation has no solution?
-If simplification leads to a false statement like 3 = 5, the equation has no solution.
What does it mean when an equation results in a true statement like 0 = 0?
-It indicates that the equation has infinitely many solutions, as any value of x satisfies the equation.
In the first example, what was the result of the simplification?
-The result was -7x = -7x, leading to infinitely many solutions.
What conclusion was reached in the second example where the result was 3 = 2?
-It concluded that there is no solution since 3 cannot equal 2.
What was the solution to the third equation?
-The solution was x = 1/9, indicating that there is exactly one solution.
How can you manipulate equations to analyze solutions?
-You can manipulate equations by adding, subtracting, multiplying, or dividing both sides to simplify and reveal relationships between the terms.
Why is it important to recognize different types of solutions?
-Recognizing different types of solutions helps in understanding the behavior of the equations and in solving real-world problems effectively.
Can an equation ever have more than one solution?
-No, an equation can only have one specific solution, infinitely many solutions, or no solution; it cannot have multiple distinct solutions.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
5.0 / 5 (0 votes)