Pair of Linear Equations in Two Variables Class 10
Summary
TLDRIn this video, the concept of linear equations in two variables is explored, covering unique, infinite, and no solutions through both algebraic and graphical methods. Three students are given different problems, resulting in distinct outcomes: a unique solution, infinite solutions, and no solution. The video explains how the graphical method shows intersecting lines, coincident lines, and parallel lines to represent these solutions. Additionally, a shortcut method is introduced to predict the nature of solutions without solving the equations. The session wraps up with practice questions for the viewers.
Takeaways
- 😀 Linear equations in two variables, also known as a system of linear equations or simultaneous equations, can result in different outcomes: a unique solution, infinite solutions, or no solution.
- 😀 There are two main methods for solving these equations: the algebraic method and the graphical method, each offering a different way to visualize or compute the solution.
- 😀 A unique solution occurs when two lines intersect at exactly one point, as seen in the graphical method, which represents the solution to the system of equations.
- 😀 Infinite solutions arise when the two equations represent the same line, making them coincident in the graph, meaning there are infinitely many points satisfying both equations.
- 😀 No solution occurs when the two equations represent parallel lines that never intersect, indicating that there are no common points of solution.
- 😀 Students’ answers can be categorized into three possible cases: unique solution, infinite solutions, or no solution, based on how the equations are related.
- 😀 The first example illustrates a unique solution, where the two lines intersect at one point. The second example shows infinite solutions, where the two equations represent the same line.
- 😀 The third example demonstrates no solution, with two parallel lines that do not intersect, meaning there are no points that satisfy both equations.
- 😀 A shortcut method based on the coefficients and constants of the equations allows us to predict the type of solution without solving the equations explicitly.
- 😀 The shortcut method uses the ratios of the coefficients and constants (a1/a2, b1/b2, and c1/c2) to determine whether the system has a unique solution, infinite solutions, or no solution at all.
Q & A
What are the three types of solutions a system of linear equations in two variables can have?
-The three types of solutions a system of linear equations in two variables can have are: a unique solution, infinite solutions, or no solution.
What was the outcome of the first student's problem in the video?
-The first student got a unique solution, with the values X = 6 and Y = 4.
What does it mean when a system of linear equations has infinite solutions?
-A system of linear equations has infinite solutions when both equations represent the same line, so every point on the line is a solution.
How do we determine if a system of linear equations has no solution?
-A system has no solution when the lines representing the equations are parallel and never intersect, meaning they have no common points.
In the second problem, what method was used to show that the system has infinite solutions?
-In the second problem, both the algebraic method (substituting and simplifying) and the graphical method (seeing that both lines overlap) were used to show that the system has infinite solutions.
What happens if you divide the second equation by 3 in the second problem?
-When you divide the second equation by 3, it becomes the same as the first equation, showing that the system represents only one equation with two variables, leading to infinite solutions.
Why did the third student get no solution in the third problem?
-The third student got no solution because the two equations represented parallel lines, which never intersect, indicating that there is no common solution.
How can you visually confirm that two equations have no solution using a graph?
-You can confirm that two equations have no solution by plotting their graphs. If the lines are parallel and never intersect, the system has no solution.
What is the shortcut method mentioned for determining the type of solution a system of linear equations has?
-The shortcut method involves comparing the coefficients and constants of the equations. If the ratios of the coefficients are equal, but not the ratio of the constants, the system has no solution. If all ratios are equal, the system has infinite solutions, and if the ratios are not equal, the system has a unique solution.
What is the condition for a system of linear equations to have a unique solution using the shortcut method?
-A system of linear equations will have a unique solution if the ratio of the coefficients of X (a1/a2) is not equal to the ratio of the coefficients of Y (b1/b2).
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Pair of Linear Equations in two variables| Part-1| Class 10| Introduction |Mathematics NCERT / CBSE

PERSAMAAN LINEAR DUA VARIABEL (PLDV) KELAS 9

Lecture 36: System of Linear Equations

ILLUSTRATING SYSTEMS OF LINEAR EQUATIONS IN TWO VARIABLES || GRADE 8 MATHEMATICS Q1

Übungsblatt6 – LGS mit 2 Unbekannten lösen und geometrische Interpretation Aufg.1c, e und f
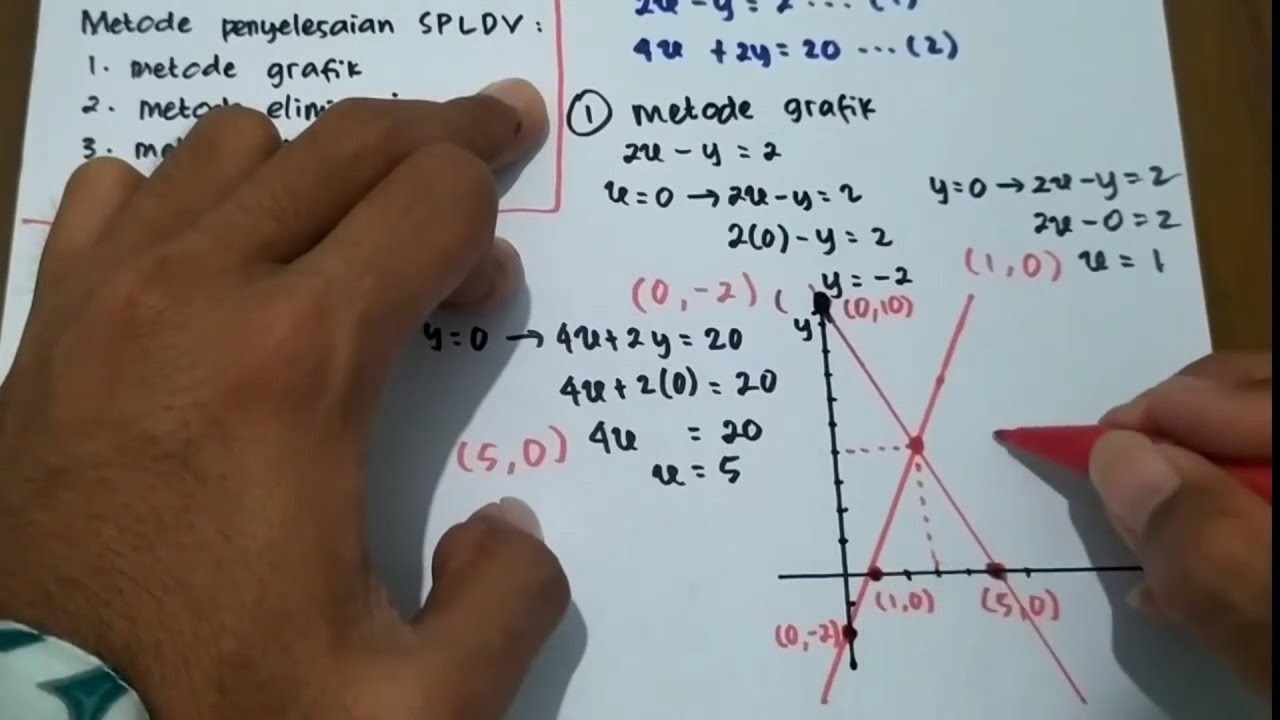
Sistem persamaan linear dua variabel kelas 10 - metode grafik
5.0 / 5 (0 votes)