Interior and Exterior Angles of a Polygon

Summary

This educational script explains the relationship between the interior and exterior angles of polygons. It highlights that the sum of interior angles in a triangle is 180 degrees and demonstrates how this sum increases with the number of sides in a polygon, using examples like squares, pentagons, and hexagons. The formula for calculating the sum of interior angles is presented as (n-2)*180, where 'n' is the number of sides. Additionally, it covers that the sum of exterior angles for any polygon is 360 degrees, and how to find the number of sides using the exterior angle. The script encourages viewers to practice these concepts with provided problems.

Takeaways

• 🔹 The interior angles of a triangle always sum up to 180 degrees.
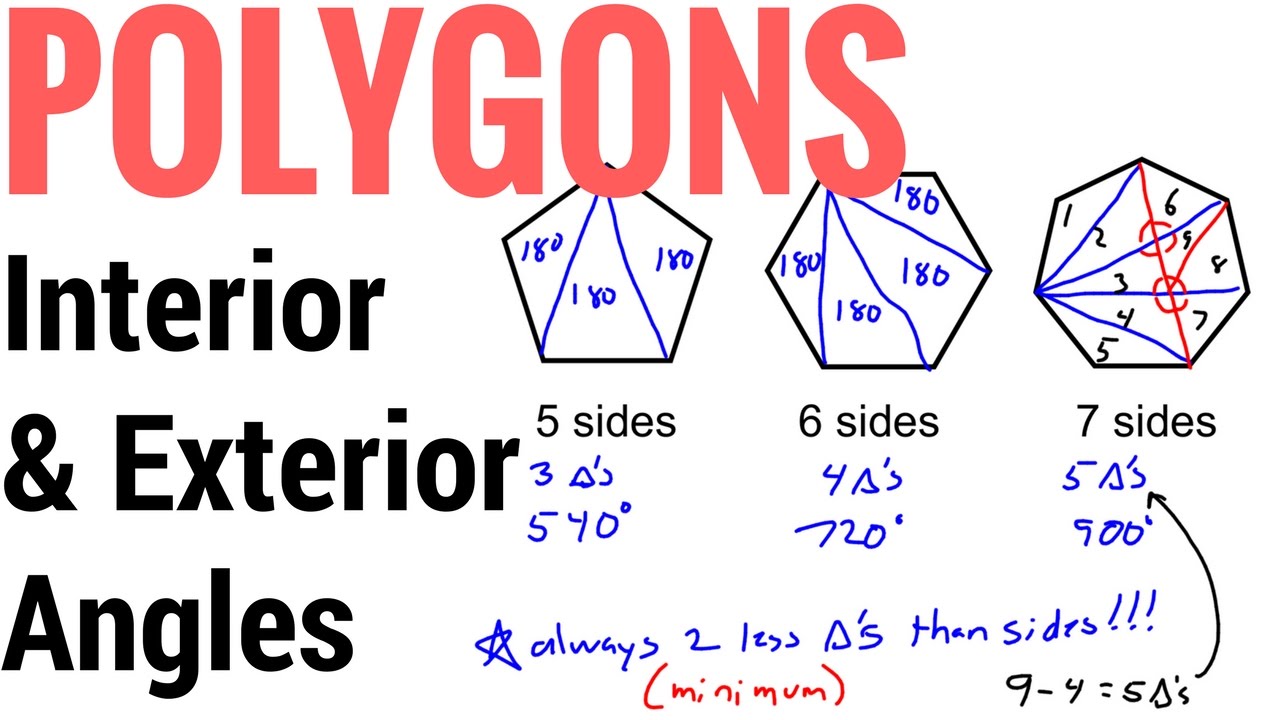
• 🔹 A square, which is a quadrilateral, has interior angles summing to 360 degrees (2 x 180 degrees).
• 🔹 An irregular pentagon can be divided into three triangles, hence its interior angles sum to 540 degrees (3 x 180 degrees).
• 🔹 A hexagon can be divided into four triangles, with interior angles summing to 720 degrees (4 x 180 degrees).
• 🔹 The number of triangles that can fit inside a polygon is always two less than the number of its sides.
• 🔹 The formula to find the sum of the interior angles of any polygon is (n - 2) * 180 degrees, where 'n' is the number of sides.
• 🔹 The exterior angles of any polygon always sum up to 360 degrees.
• 🔹 The exterior angle of a polygon can be calculated by subtracting the interior angle from 180 degrees, using the fact that angles on a straight line sum to 180 degrees.
• 🔹 For a regular polygon, the number of sides can be determined by dividing 360 degrees by the measure of one exterior angle.
• 🔹 Each interior angle of a regular polygon can be calculated by dividing the sum of all interior angles by the number of sides.

Q & A

What is the sum of the interior angles of a triangle?

The sum of the interior angles of a triangle is always 180 degrees.

How can you determine the sum of the interior angles of a square?

Since a square can be divided into two triangles, the sum of its interior angles is two lots of 180 degrees, which equals 360 degrees.

What is the relationship between the number of triangles that can fit inside a polygon and the number of sides of the polygon?

The number of triangles that can fit inside any polygon is always two less than the number of sides of the polygon.

How do you calculate the sum of the interior angles of a polygon given the number of sides?

To find the sum of the interior angles of a polygon, you take the number of sides 'n', subtract two (to find how many triangles can fit inside), and then multiply by 180 degrees.

What is the sum of the exterior angles of any polygon?

The sum of the exterior angles of any polygon is always 360 degrees.

How can you find the exterior angle of a polygon if you know the interior angle?

If you know the interior angle, you can calculate the exterior angle by using the fact that angles on a straight line add up to 180 degrees.

For a regular polygon, how can you determine the number of sides using the exterior angle?

For a regular polygon, you can find the number of sides by dividing 360 degrees by the measure of the exterior angle.

How can you calculate each interior angle of a polygon?

You can calculate each interior angle of a polygon by dividing the sum of the interior angles by the number of angles or sides.

What is the significance of the number 180 degrees in the context of polygon angles?

The number 180 degrees is significant because it represents the sum of the interior angles of a triangle, which is the building block for calculating the sum of interior angles of any polygon.

Can the formula for the sum of the interior angles be applied to irregular polygons?

Yes, the formula for the sum of the interior angles can be applied to both regular and irregular polygons, as it is based on the number of sides and the geometric property that the sum of angles in a triangle is 180 degrees.

Outlines

00:00

🔢 Interior and Exterior Angles of Polygons

This paragraph explains the relationship between the interior and exterior angles of polygons. It starts by stating that the interior angles of a triangle always sum up to 180 degrees. The concept is then extended to other polygons like squares, pentagons, and hexagons, illustrating that the sum of interior angles is directly proportional to the number of triangles that can fit inside the polygon. The formula for calculating the sum of interior angles of any polygon is introduced as (n-2)*180, where 'n' is the number of sides. The paragraph also covers how to find the sum of exterior angles, which always totals 360 degrees for any polygon. For regular polygons, the number of sides can be determined by dividing 360 by the measure of the exterior angle. The script encourages viewers to practice these concepts with provided examples and pause the video to work through the questions.

Keywords

💡Interior Angles

Interior angles are the angles formed by two adjacent sides of a polygon. In the video, it is emphasized that the sum of interior angles of a triangle, which is a three-sided polygon, always adds up to 180 degrees. This concept is extended to polygons with more sides, where the sum of interior angles can be calculated by multiplying the number of triangles that can fit inside the polygon by 180 degrees. The video uses the example of a square, where two triangles can be formed, thus the sum of interior angles equals two lots of 180 degrees.

💡Exterior Angles

Exterior angles are the angles formed by one side of a polygon and the extension of an adjacent side. The video explains that the sum of exterior angles of any polygon always adds up to 360 degrees. This is a fundamental property used to calculate the exterior angles when the interior angles are known, using the fact that angles on a straight line add up to 180 degrees.

💡Polygon

A polygon is a closed two-dimensional shape with straight sides, formed by three or more line segments. The video discusses various polygons, including triangles, squares, pentagons, and hexagons, and how the number of sides affects the sum of interior and exterior angles.

💡Sum of Interior Angles

The sum of interior angles is a property of polygons that refers to the total measure of all the interior angles. The video provides a formula to calculate this sum for any polygon, which is based on the number of sides (n) and the number of triangles that can fit inside the polygon. The formula is (n - 2) * 180 degrees.

💡Triangles Inside a Polygon

The video explains that the number of triangles that can be formed inside a polygon is always two less than the number of sides of the polygon. This relationship is key to the formula for calculating the sum of interior angles of any polygon.

💡Irregular Pentagon

An irregular pentagon is a five-sided polygon where not all sides and angles are equal. The video uses an irregular pentagon as an example to illustrate that it can be divided into three triangles, hence the sum of its interior angles would be three times 180 degrees.

💡Hexagon

A hexagon is a six-sided polygon. In the video, a hexagon is used to demonstrate that it can fit four triangles inside, and therefore, the sum of its interior angles would be four times 180 degrees.

💡Formula

The video introduces a formula for calculating the sum of the interior angles of any polygon. The formula is derived from the relationship between the number of sides of the polygon and the number of triangles that can fit inside it, which is (n - 2) * 180 degrees.

💡Interior Angle Calculation

The video explains how to calculate each individual interior angle of a polygon by dividing the sum of the interior angles by the number of sides or angles in the polygon. This method is applicable after determining the total sum of interior angles using the formula.

💡Exterior Angle of a Regular Polygon

The video discusses a method to find the number of sides of a regular polygon (where all sides and angles are equal) by dividing 360 degrees by the measure of one exterior angle. This is based on the property that the sum of exterior angles of any polygon is 360 degrees.

Highlights

Interior angles of a triangle always add up to 180 degrees.

A square's interior angles equal two lots of 180 degrees since it can fit two triangles.

An irregular pentagon's interior angles equal three lots of 180 degrees fitting three triangles.

A hexagon's interior angles equal four lots of 180 degrees as it can fit four triangles inside.

The number of triangles fitting inside a polygon is always two less than the number of sides.

A formula for any polygon's interior angles is (n-2)*180, where 'n' is the number of sides.

Exterior angles of any polygon always add up to 360 degrees.

Exterior angles can be calculated from interior angles using the fact that angles on a straight line add up to 180 degrees.

For a regular polygon, the number of sides can be found by dividing 360 by the exterior angle.

Each interior angle can be calculated by dividing the sum of interior angles by the number of sides.

The video provides an example to illustrate the calculation of interior angles.

The video encourages viewers to pause and work through practice questions.

Understanding the relationship between the number of sides and the number of triangles is key to solving polygon problems.

The formula (n-2)*180 is a versatile tool for calculating the sum of interior angles in any polygon.

The sum of exterior angles is a constant 360 degrees, regardless of the polygon's shape.

The video explains how to use the sum of interior and exterior angles to solve for unknown angles in polygons.

The concept of angles on a straight line is crucial for calculating exterior angles from interior angles.

The video demonstrates how to determine the number of sides of a regular polygon using exterior angles.

Transcripts

Interior and exterior angles.

The first and most important thing to note  is that the interior angles of a triangle  

will always add up to 180 degrees.

Here we have a square, notice that we can fit two  

triangles in the square. Therefore the interior  angles will equal two lots of 180 degrees.

Next we have an irregular pentagon in  which we can fit three triangles inside  

Therefore the interior angles will  be equal to three lots of 180 degrees.

Here we have a hexagon which  can fit four triangles inside,

so the interior angles will be equal to four lots  of 180 degrees, as there are four triangles inside.

Noticed anything?

The number of triangles which can fit inside  any polygon will always be two less than the  

number of sides. So now we can create a  formula which we can use for any polygon

to find the sum of the interior angles. 'n' is  the number of sides, take away two (this gives  

us how many triangles can fit inside  the polygon), we then times this by 180.

Have a look at this example:

from this we can also  calculate each interior angle

by dividing our answer by  the number of angles or sides.

Now we are looking at exterior angles. The exterior  angles of any polygon will always add up to

360 degrees. If we are given the interior angle we  can calculate the exterior by using the

angles on a straight line fact. This is, that angles on a  straight line will always add up to 180 degrees

For a regular polygon only, we can find  the number of sides of the polygon  

by dividing 360 by the exterior angle.

Have a go at these questions. Pause  the video whilst you work them out.