Matematika Kelas 8 Bab 4 - Sudut Dalam Segi Banyak - hal. 110 - 112 - Kurikulum Merdeka

Summary

This educational video script focuses on teaching eighth-grade students about the properties of geometric shapes, particularly the sum of interior angles in polygons. The script outlines methods for calculating the interior angles of polygons such as pentagons, quadrilaterals, hexagons, and more, using visual aids and step-by-step explanations. It introduces key concepts like dividing polygons into triangles to determine angle sums and uses formulas involving the number of sides to calculate interior angles. The video aims to enhance students' understanding of geometry through practical examples and various problem-solving techniques.

Takeaways

• 😀 The script covers a mathematics lesson for 8th-grade students, focusing on the properties of geometric shapes, specifically polygons.
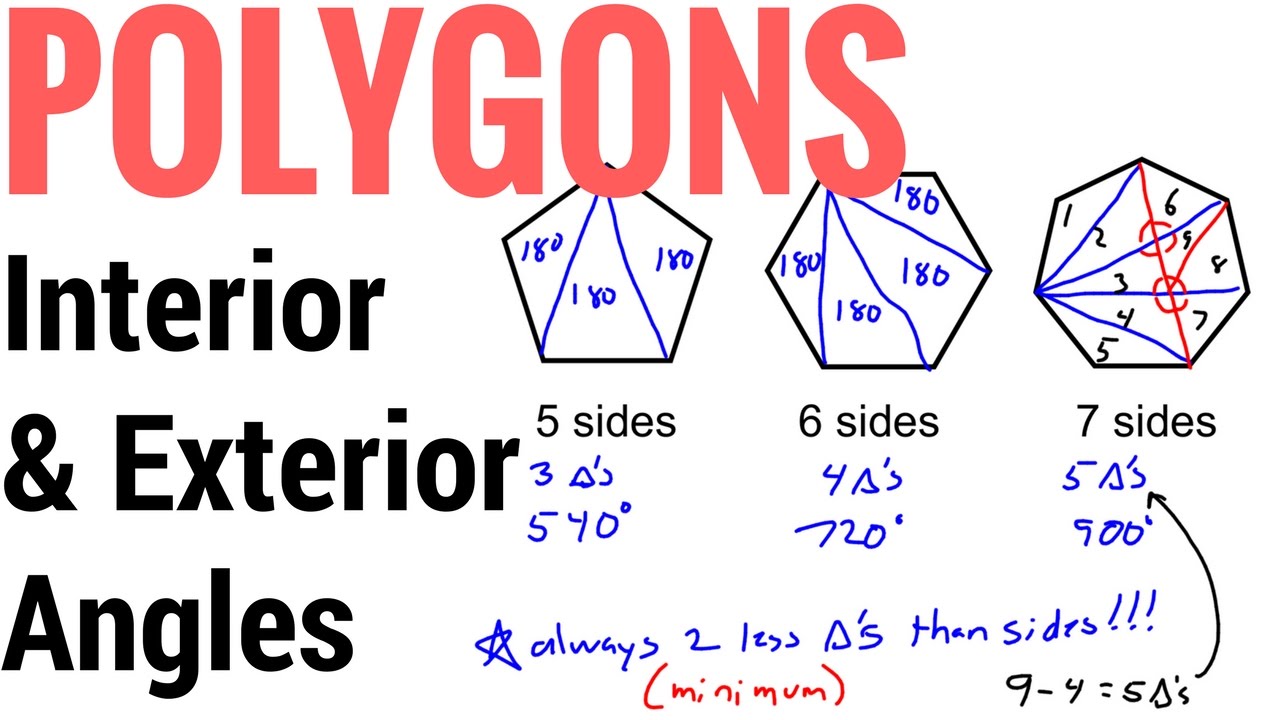
• 😀 The lesson introduces the concept of calculating the sum of interior angles in polygons, starting with a pentagon (five-sided polygon).
• 😀 The method used to calculate the sum of interior angles of a polygon is by dividing the polygon into triangles and summing their angles.
• 😀 The sum of interior angles of a polygon is calculated by multiplying the number of triangles formed (n - 2) by 180 degrees.
• 😀 For a pentagon, the polygon is divided into three triangles, each contributing 180 degrees, resulting in a sum of 540 degrees for the pentagon's interior angles.
• 😀 The lesson includes various examples for different polygons, such as a triangle, quadrilateral, hexagon, heptagon, and octagon, using the same method.
• 😀 For each polygon, the number of triangles and the corresponding sum of interior angles are calculated and presented in a table format.
• 😀 A pattern is identified, where the number of triangles in a polygon can be derived by subtracting 2 from the number of vertices (or corners) of the polygon.
• 😀 The formula for calculating the sum of interior angles of a polygon with 'n' vertices is (n - 2) * 180 degrees.
• 😀 The lesson concludes with an exercise where students are tasked with applying the formula to calculate the sum of interior angles for different polygons, such as a dodecagon (12 sides).

Q & A

What is the main topic of the lesson in the script?

The main topic of the lesson is about investigating the properties of geometric shapes, particularly focusing on calculating the sum of interior angles of polygons.

What method is used to calculate the sum of interior angles of a Pentagon in the script?

The method used involves dividing the Pentagon into triangles by drawing two diagonals from one vertex, which results in three triangles. The sum of the interior angles of the Pentagon is then calculated as 3 * 180° = 540°.

How does the script explain the sum of interior angles of different polygons?

The script explains that the sum of interior angles of a polygon can be found by dividing the polygon into triangles. The sum of the angles of each triangle is 180°, and the number of triangles is determined by subtracting 2 from the number of sides (n - 2).

What formula is provided in the script to calculate the sum of interior angles for any polygon?

The formula provided is (n - 2) * 180°, where 'n' is the number of sides (or vertices) of the polygon.

How is the number of triangles related to the number of sides of a polygon in the script?

The number of triangles formed by drawing diagonals in a polygon is equal to (n - 2), where 'n' is the number of sides of the polygon.

What was the approach used by Dewi to find the sum of the interior angles of a Pentagon?

Dewi's approach involved drawing lines from an internal point (P) to each vertex of the Pentagon, creating five triangles. She then calculated the sum of the angles of these triangles as 5 * 180° = 900°, and subtracted the central angle (360°) to get 540°.

How do the sum of interior angles and the number of sides of a polygon relate to each other?

The sum of interior angles of a polygon is directly related to the number of sides. The formula (n - 2) * 180° shows how the sum increases as the number of sides increases.

What is the sum of interior angles for a polygon with 12 sides?

Using the formula (n - 2) * 180°, for a polygon with 12 sides, the sum of the interior angles is (12 - 2) * 180° = 10 * 180° = 1800°.

How does the script calculate the measure of each interior angle in a regular polygon?

To calculate the measure of each interior angle in a regular polygon, the total sum of interior angles is divided by the number of sides. For a 12-sided regular polygon, each angle is 1800° ÷ 12 = 150°.

What geometric concept does the script emphasize in its explanation of polygons and their angles?

The script emphasizes the concept of dividing polygons into triangles to simplify the calculation of their interior angles, and also highlights the relationship between the number of sides and the sum of interior angles.