Trigonometric Graphs - GCSE Higher Maths
Summary
TLDRThis educational video explores the graphs of trigonometric functions, focusing on y = sin(x), y = cos(x), and y = tan(x). It explains how to sketch these graphs for 0° to 360°, identifying key points and shapes. The video also covers the periodic nature of the graphs, with periods of 360° for sine and cosine, and 180° for tangent. Practical problem-solving using these graphs is demonstrated, showing how to estimate solutions to trigonometric equations by finding where horizontal lines intersect the graphs. The tutorial is designed to aid in understanding and applying trigonometric concepts.
Takeaways
- 📊 The video covers the trigonometric graphs of y = sin(x), y = cos(x), and y = tan(x), focusing on their shapes and applications.
- 🔢 Key points for sketching y = sin(x) include (0,0), (90,1), (180,0), (270,-1), and (360,0), reflecting fundamental trigonometric values.
- 📈 The graph of y = cos(x) is similar to y = sin(x) but starts at (0,1) and has its peak at 360°, shifted by 90°.
- 🔄 Both y = sin(x) and y = cos(x) are periodic with a period of 360°, repeating their wave pattern infinitely in both directions.
- 🚫 The graph of y = tan(x) is distinct, with vertical asymptotes at 90° and 270° where the function is undefined.
- 🔢 For y = tan(x), the key points include (0,0), (180,0), and (360,0), and it has a period of 180°, different from sin and cos.
- 🔍 To solve equations using these graphs, one can estimate x values where the graph intersects a horizontal line at a given y value.
- 📏 The video demonstrates how to use symmetry and periodicity to find solutions for trigonometric equations when graphs are not drawn to scale.
- 📝 Examples are provided to solve for sin(x) = 0.6, cos(x) = 0.3, and tan(x) = K, showing how to find x values from the graph intersections.
- 🔄 The video also addresses how to deal with periodicity to find solutions outside the initially provided x range, such as finding the lowest value of M for cos(m) = K where m is between 360 and 720.
Q & A
What are the three trigonometric functions whose graphs are discussed in the video?
-The three trigonometric functions whose graphs are discussed in the video are y = sin(x), y = cos(x), and y = tan(x).
What are the key points that define the graph of y = sin(x) within the range of 0° to 360°?
-The key points that define the graph of y = sin(x) within the range of 0° to 360° are (0, 0), (90, 1), (180, 0), (270, -1), and (360, 0).
How does the graph of y = cos(x) differ from that of y = sin(x)?
-The graph of y = cos(x) is similar in shape to y = sin(x) but is translated 90° to the left, starting at (0, 1) and reaching (90, 0), (180, -1), (270, 0), and (360, 1).
Why are there vertical asymptotes on the graph of y = tan(x)?
-There are vertical asymptotes on the graph of y = tan(x) because tan(x) is undefined at 90° and 270°, which are the points where the graph approaches infinity.
What is the period of the graph for y = sin(x) and y = cos(x)?
-The period of the graph for both y = sin(x) and y = cos(x) is 360°, as they repeat their pattern every 360°.
How does the graph of y = tan(x) differ in terms of period compared to y = sin(x) and y = cos(x)?
-The graph of y = tan(x) has a period of 180°, which is different from y = sin(x) and y = cos(x) whose periods are 360°.
What is the significance of the point (90, 1) on the graph of y = sin(x)?
-The point (90, 1) on the graph of y = sin(x) signifies that the sine of 90° is equal to 1, which is one of the exact trigonometric values that need to be memorized.
How can you use the graph of y = sin(x) to solve an equation like sin(x) = 0.6 for x values between 0 and 360?
-You can use the graph of y = sin(x) to solve sin(x) = 0.6 by drawing a horizontal line at y = 0.6 and finding the x-intercepts, which represent the solutions to the equation within the given range.
What symmetry property can be used to find the value of 'a' in the equation sin(a) = K, where a is between 90 and 360, given sin(37) = K?
-The symmetry property of the graph of y = sin(x) can be used by recognizing that the graph is symmetrical about the line x = 180°. The distance from the origin to 37° is 37, so the same distance from 180° to 'a' is also 37, leading to the calculation of 'a' as 180° - 37° = 143°.
How can you estimate the solutions for an equation using the graph of a trigonometric function without a grid?
-You can estimate the solutions by using the symmetry properties of the graph and the known exact trigonometric values to find corresponding x-values that yield the given y-value.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео
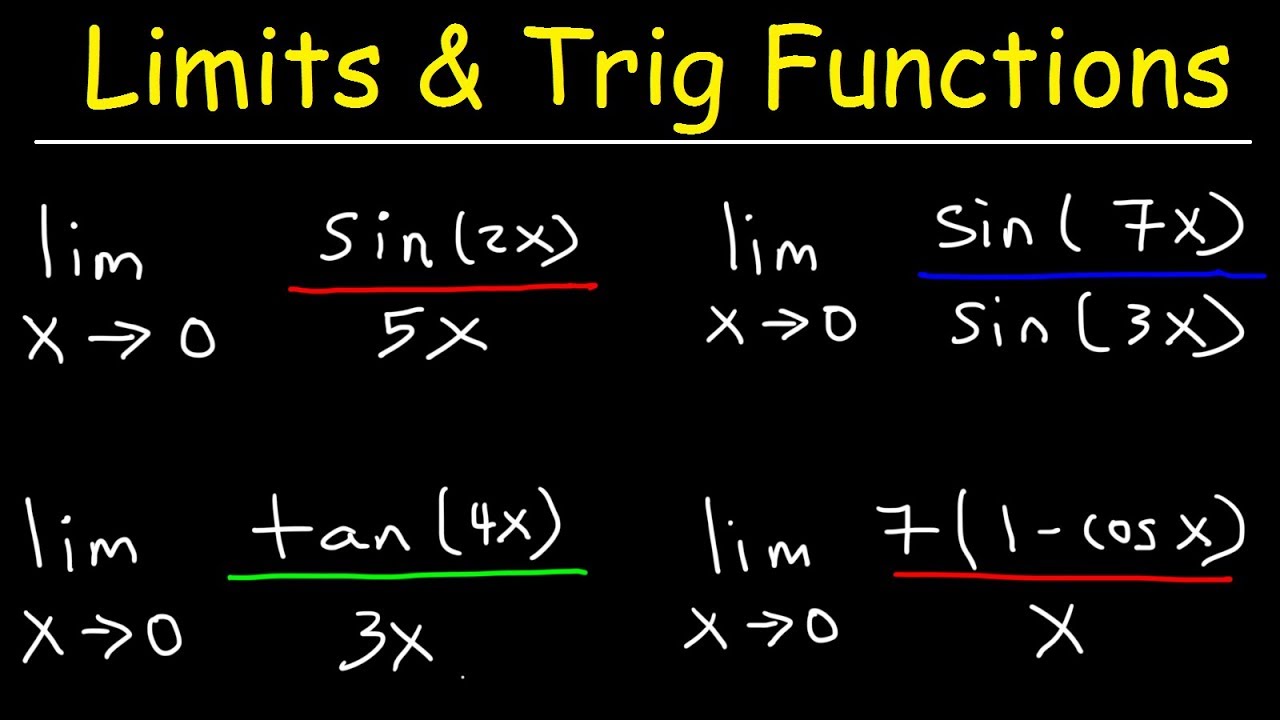
Limits of Trigonometric Functions
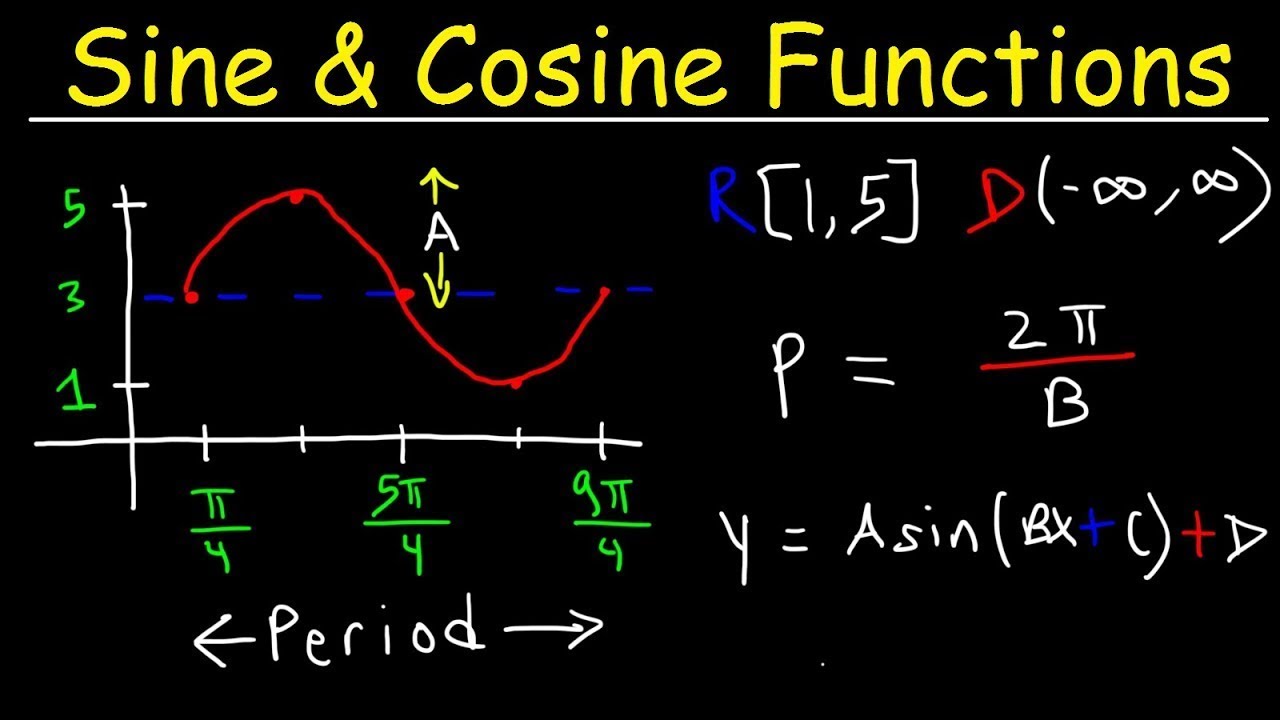
How To Graph Trigonometric Functions | Trigonometry

Turunan Fungsi Trigonometri 2: Dalil-Dalil Turunan Fungsi Trigonometri

prove geometrically that cos(x+y) = cosx cosy - sinx siny | 💯 guaranteed question | class : 11
Turunanfungsi Trigonometri Bag 1
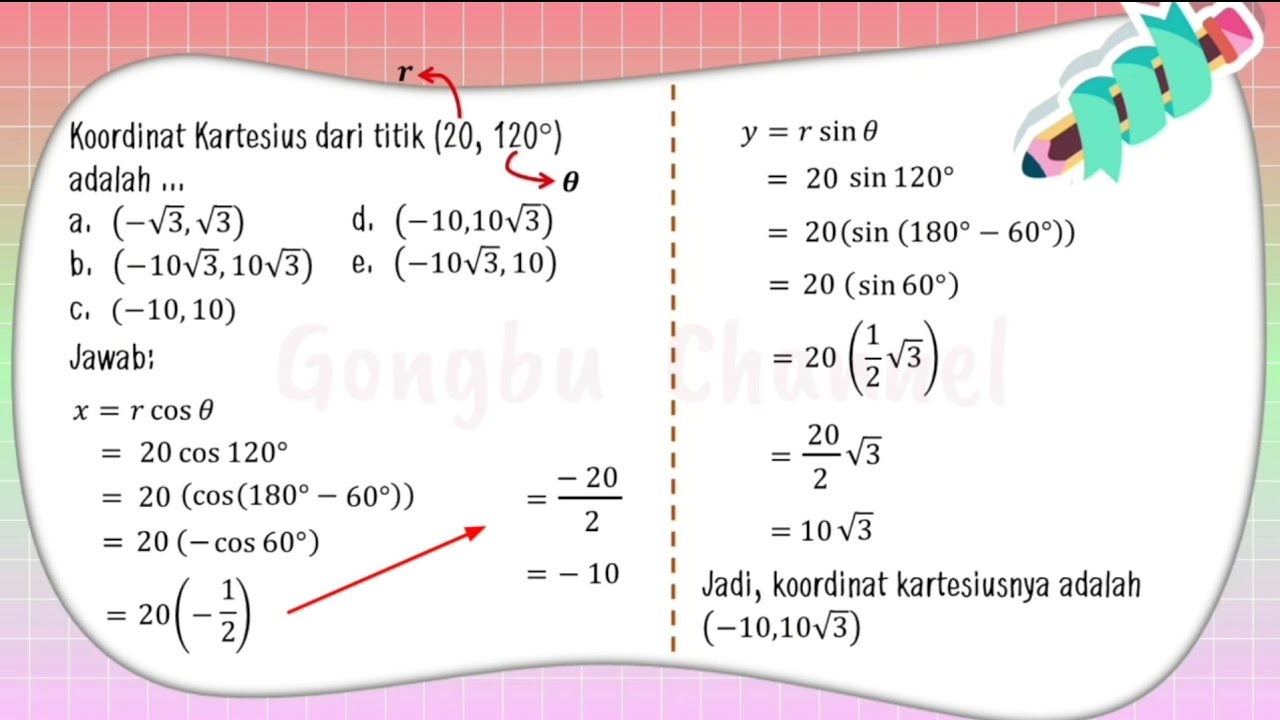
TRIGONOMETRI (Konversi Koordinat Kutub Ke Koordinat Kartesius)
5.0 / 5 (0 votes)
