Pre Calculus - Graph of Parabola | Finding Vertex, Focus, Directrix and Axis of Symmetry of Parabola
Summary
TLDRIn this educational video, the teacher guides viewers through the process of identifying key features of a parabola using its equation. The focus is on determining the vertex, focus, directrix, and axis of symmetry for the parabola given by the equation x^2 = -4y. The video explains that the vertex is at the origin, the orientation is downward due to the negative coefficient, and the focus is calculated to be at (0, -1). The directrix is a line at y = 1, and the axis of symmetry is the x-axis. The video concludes with a practical demonstration of sketching the parabola, making it an informative resource for learners.
Takeaways
- 📚 The video's main focus is on teaching how to determine the vertex, focus, directrix, and axis of symmetry of a parabola given its equation.
- 🔍 The equation provided for the parabola is x^2 = -4y, which is used to identify the parabola's key features.
- 📍 The vertex of the parabola is identified as being at the origin (0,0), which is the center of the coordinate plane.
- ⬇️ The orientation of the parabola is determined to be downward due to the negative coefficient in the equation.
- 🔍 The value of 'c', which represents the distance from the vertex to the focus and directrix, is calculated to be 1 unit.
- 📍 The focus of the parabola is located at coordinates (0, -1), one unit below the vertex.
- 📏 The directrix is described as a line, perpendicular to the y-axis, and is given by the equation y = 1.
- 🔄 The axis of symmetry for the parabola is the y-axis, as the vertex lies on the origin, making the x-axis (x = 0) the axis of symmetry.
- 📈 The endpoints of the parabola are calculated to be at (-2, -1) and (2, -1), which helps in graphing the parabola.
- 🎨 The video concludes with a sketch of the parabola, demonstrating how to graph it using the identified parts.
Q & A
What is the main topic of the video?
-The main topic of the video is determining the vertex, focus, directrix, and axis of symmetry of a parabola given its equation, and sketching the parabola in a coordinate plane.
What is the given equation of the parabola in the video?
-The given equation of the parabola is \( x^2 = -4y \).
What does the equation \( x^2 = -4y \) indicate about the orientation of the parabola?
-The equation \( x^2 = -4y \) indicates that the parabola opens downwards since the coefficient of \( y \) is negative.
Where is the vertex of the parabola located according to the video?
-The vertex of the parabola is located at the origin, which is at coordinates (0, 0).
How is the value of 'c' determined from the given equation?
-The value of 'c' is determined by taking the absolute value of the coefficient of \( y \) in the equation, which is 4 in this case, so \( c = 1 \).
What are the coordinates of the focus of the parabola?
-The coordinates of the focus of the parabola are (0, -1), as it is one unit below the vertex along the y-axis.
What is the equation of the directrix of the parabola?
-The equation of the directrix of the parabola is \( y = 1 \), which is one unit above the vertex along the y-axis.
What is the axis of symmetry for this parabola?
-The axis of symmetry for this parabola is the y-axis, which is represented by the equation \( x = 0 \).
How are the endpoints of the parabola determined in the video?
-The endpoints of the parabola are determined by using the value of \( 2c \), which is 2 in this case, and calculating the points at (-2, -1) and (2, -1).
What is the significance of the value \( 2c \) in the context of the parabola?
-The value \( 2c \) represents the distance from the vertex to the endpoints of the parabola along the x-axis.
What is the key takeaway from the video for sketching a parabola?
-The key takeaway is that by identifying the vertex, focus, directrix, axis of symmetry, and endpoints of the parabola, one can accurately sketch the parabola given its equation.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
Solving Quadratic Equation Using Quadratic Formula

Gramática no Enem [Prof. Noslen] #NoEnemcomoNoslen #enem #português

How to Solve Quadratic Equations by Completing the Square? Grade 9 Math
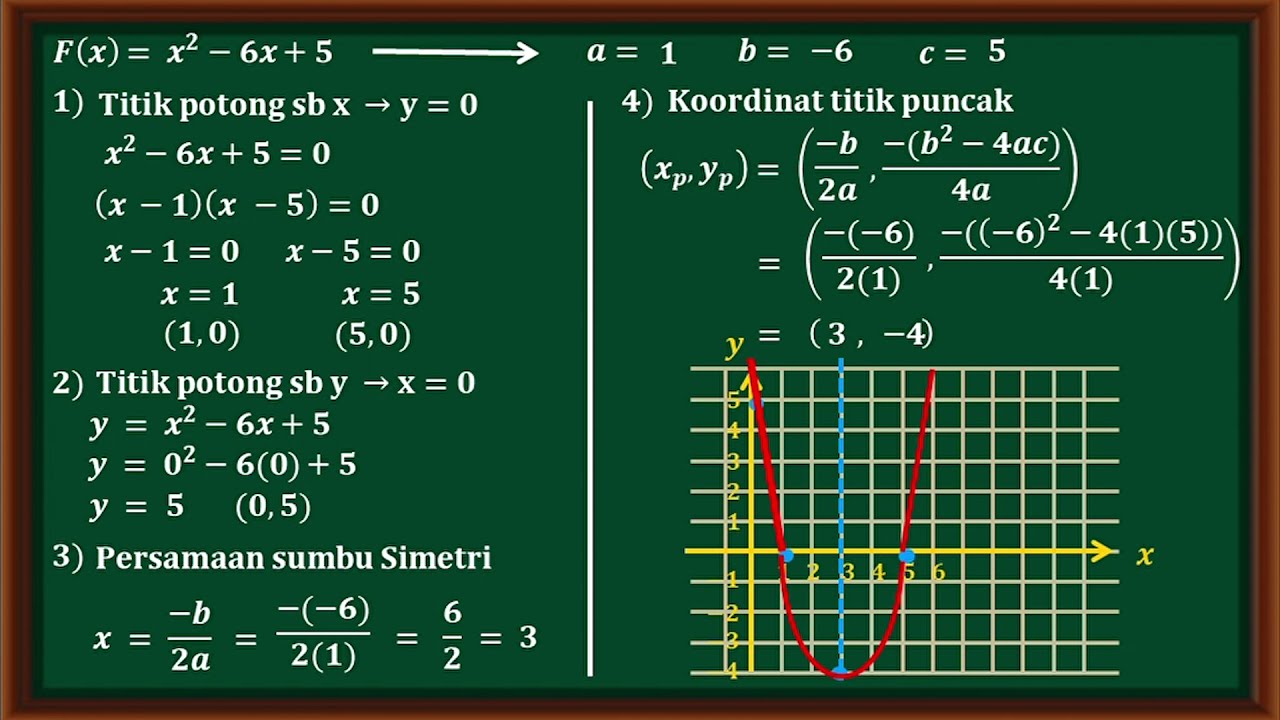
Cara membuat grafik fungsi kuadrat
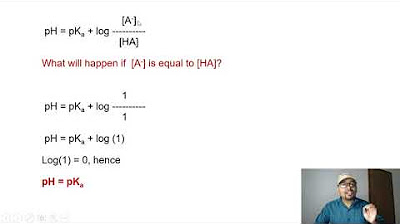
Measurement of pKa by Potentiometry
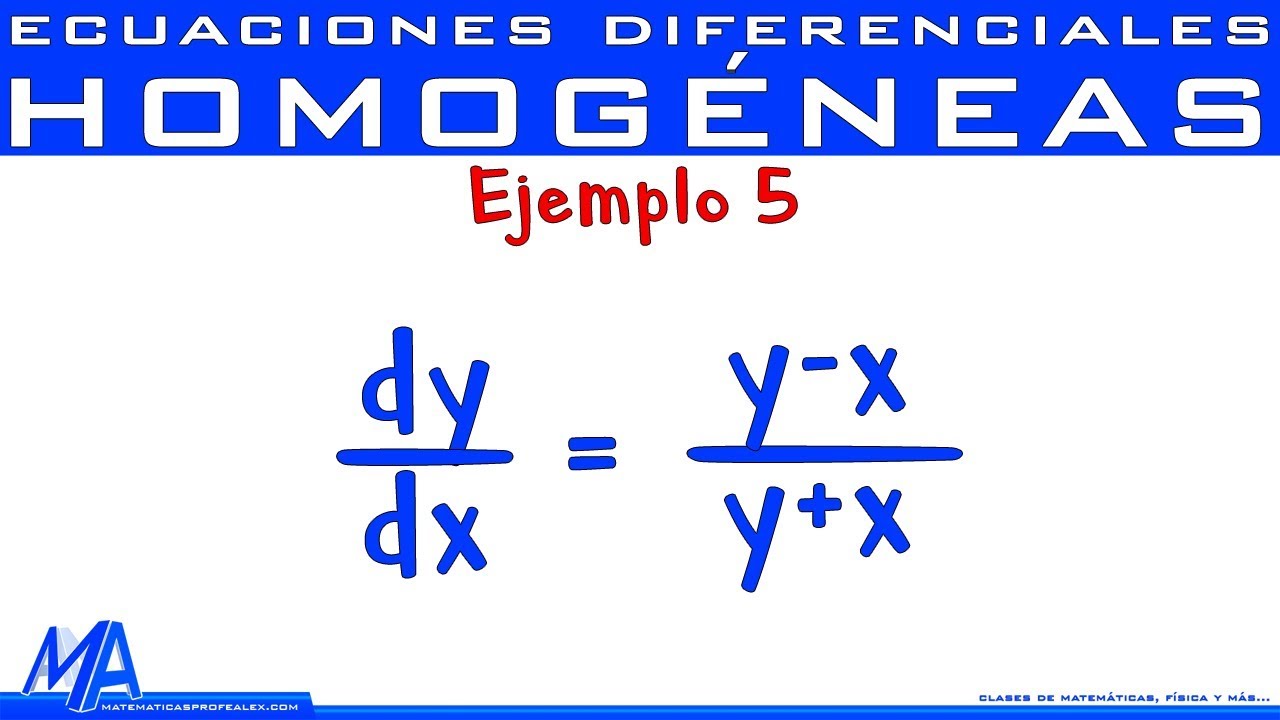
Ecuaciones diferenciales Homogéneas | Ejemplo 5
5.0 / 5 (0 votes)
