Statistics - Module 3 - Mean, Median, Mode, Percentiles and Quartiles - Problem 3-1B
Summary
TLDRThis educational video script delves into statistical measures of central tendency, focusing on mean, median, and mode. It explains the arithmetic mean, or average, by demonstrating its calculation with a simple dataset. The script contrasts the mean's sensitivity to extreme values with the median's resilience, which divides a dataset into two equal halves. It also introduces the mode as the most frequent value, noting the possibility of multiple modes leading to a multimodal distribution. The presenter emphasizes the importance of understanding these basic concepts and their formal notation, as they form the foundation for more complex statistical analyses.
Takeaways
- 📊 The video discusses three measures of central tendency: mean, median, and mode.
- 🔢 The arithmetic mean (average) is calculated by summing all data points and dividing by the number of points.
- 📈 The mean is influenced by the magnitude of data points, unlike the median.
- 🎯 The median is the middle value in a data set, where 50% of observations are greater and 50% are lesser.
- 🔠 The mode is the value that occurs most frequently in a data set; a set can be unimodal, bimodal, or multimodal.
- ✅ The video demonstrates how to calculate mean, median, and mode using a sample of 30 company share prices.
- 📚 The script emphasizes the importance of understanding the notation and formulas for calculating mean, as they will be foundational for more complex concepts.
- 💡 The video provides a detailed walkthrough of calculating the mean, including the formula and its notation.
- 📋 The median is calculated by sorting the data and finding the middle value(s), which is crucial for even-numbered data sets.
- 🔍 The mode is identified by observing the frequency of each value in the sorted data set.
- 📝 The video concludes by reinforcing the importance of comfort with basic statistical calculations and notation for future learning.
Q & A
What are the three measures of central location discussed in the video?
-The three measures of central location discussed in the video are mean, median, and mode.
What is the formula for calculating the arithmetic mean?
-The formula for calculating the arithmetic mean is the sum of all data points divided by the number of data points.
How does the arithmetic mean differ from the median in terms of how it is influenced by the data points?
-The arithmetic mean is influenced by the magnitude of the data points, while the median is not affected by the size of the values but rather their order.
What is the median and how is it found in a dataset?
-The median is the value that separates the higher half from the lower half of a data sample. It is found by arranging the data points in order and picking the middle value or the average of the two middle values if there is an even number of observations.
What is the mode and how is it determined?
-The mode is the value that occurs most frequently in a data set. It is determined by identifying the observation(s) that repeat the most.
Can a dataset have more than one mode? If so, what is it called?
-Yes, a dataset can have more than one mode. If it has two modes, it is called bimodal, and if it has more than two, it is called multimodal.
What is the difference between the mean of a population and the mean of a sample?
-The mean of a population is calculated using the entire dataset, while the mean of a sample is calculated using a subset of the population.
What is the notation used for the mean of a sample?
-The notation used for the mean of a sample is x-bar (x̄).
How is the mean calculated when the number of observations is even?
-When the number of observations is even, the mean is calculated by taking the average of the two middle values after the data has been sorted.
What is the importance of understanding the notation and formula for calculating the mean?
-Understanding the notation and formula for calculating the mean is important because it provides a foundation for more complex statistical concepts and calculations that will be encountered in more advanced material.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

Ch 3 Lecture Video, Fall 2024: Measures of Central Tendency
Mean, Median and Mode in Statistics | Statistics Tutorial | MarinStatsLectures
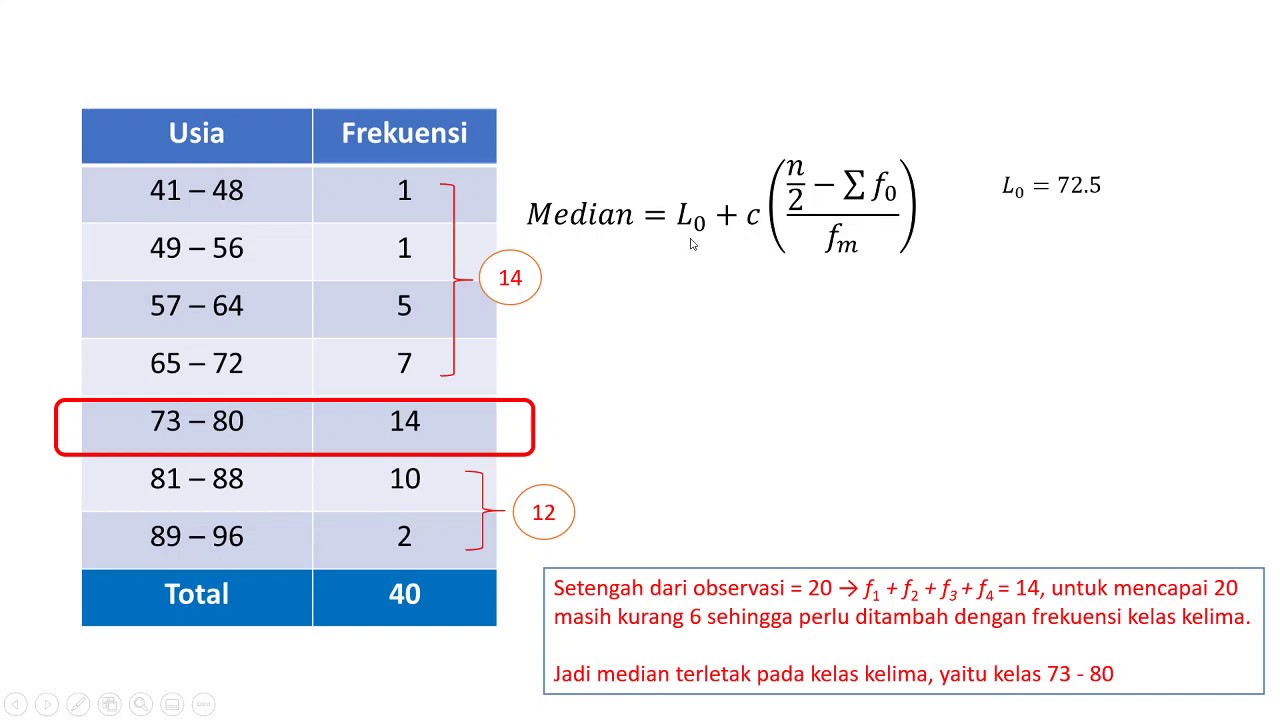
UKURAN PEMUSATAN DATA BERKELOMPOK | Rataan Median Modus Kuartil Desil Persentil

STATISTIKA: Ukuran gejala pusat dan ukuran letak 1

Mode, Median, Mean, Range, and Standard Deviation (1.3)

Ukuran pemusatan data, mean median modus, Statistika
5.0 / 5 (0 votes)
