Evaluating composite functions | Mathematics III | High School Math | Khan Academy

Summary

The video script explains the concept of function composition using two functions, g(x) = x^2 + 5x - 3 and h(y) = 3(y - 1)^2 - 5. It demonstrates how to find h(g(-6)) by first calculating g(-6), which results in 3, and then using this output as the input for h, yielding h(3) = 7. The script emphasizes the importance of understanding function composition notation and provides a step-by-step approach to solving such problems.

Takeaways

• 📘 The function g(x) is defined as x squared plus five x minus three.
• 📙 The function h(y) is defined as three times (y minus one) squared minus five.
• 🔁 Function composition is represented by a circle symbol between two functions, indicating that one function is applied after the other.
• 🤔 The process of function composition involves evaluating the inner function first and then using its result as the input for the outer function.
• 🔢 To find h(g(-6)), first calculate g(-6) by substituting -6 into the function g(x).
• 🧮 After evaluating g(-6), the result is 3, which is then used as the input for the function h(y).
• 📐 The calculation for h(3) involves squaring the result from g(-6), multiplying by three, and then subtracting five.
• 📈 The final result of h(g(-6)) is 7, which is obtained by following the steps of function composition.
• 📝 Understanding function composition is crucial for solving problems that involve nested functions.
• 📖 The script emphasizes the importance of taking a step-by-step approach to solve complex problems involving function composition.

Q & A

What is the mathematical expression for g(x) as described in the transcript?

The mathematical expression for g(x) is g(x) = x^2 + 5x - 3.

What is the mathematical expression for h(y) as described in the transcript?

The mathematical expression for h(y) is h(y) = 3(y - 1)^2 - 5.

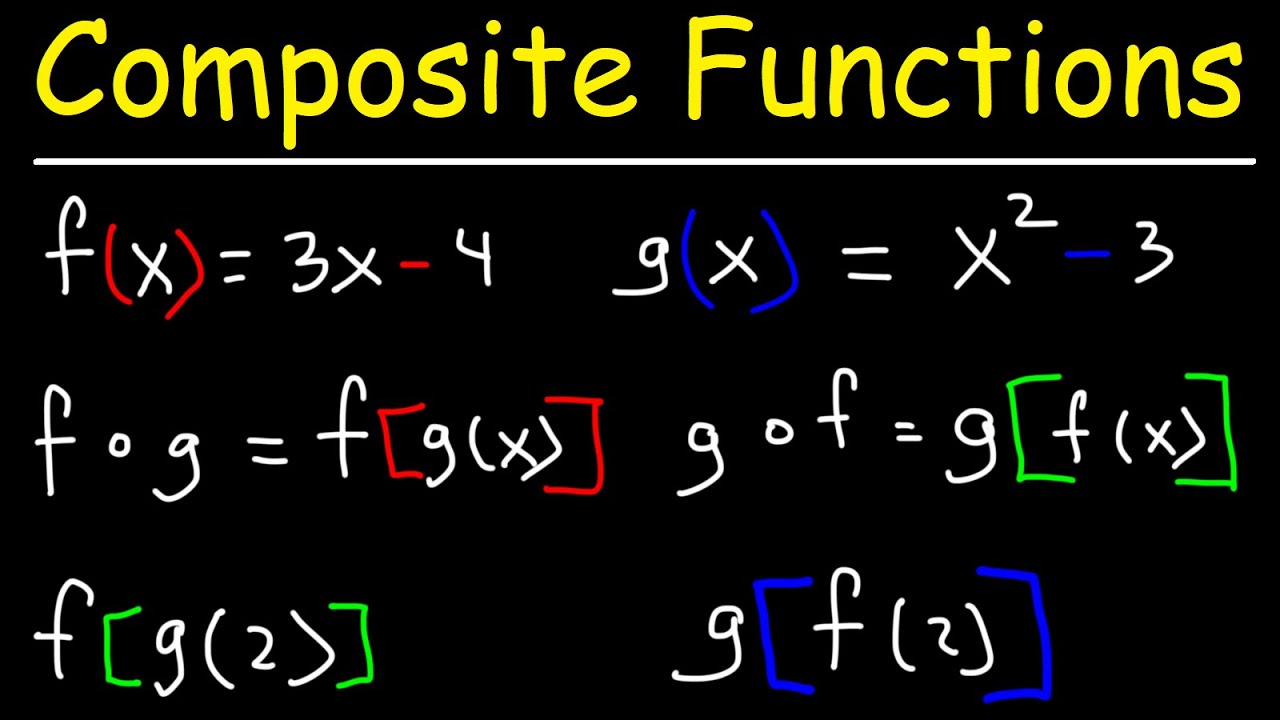
What does the function composition symbol '∘' represent?

The function composition symbol '∘' represents the application of one function to the result of another function.

How is h(g(-6)) expressed in terms of function composition?

h(g(-6)) can be expressed as h(g(x)) where x is -6, which means you first apply g(x) to -6 and then apply h(y) to the result.

What is the first step in calculating h(g(-6))?

The first step in calculating h(g(-6)) is to find the value of g(-6) by substituting -6 into the function g(x).

What is the value of g(-6) after substituting -6 into the function g(x)?

The value of g(-6) is calculated as (-6)^2 + 5*(-6) - 3, which equals 36 - 30 - 3, resulting in 3.

After finding g(-6), what is the next step in calculating h(g(-6))?

The next step is to substitute the value of g(-6), which is 3, into the function h(y) to find h(3).

What is the value of h(3) after substituting 3 into the function h(y)?

The value of h(3) is calculated as 3*(3 - 1)^2 - 5, which equals 3*(2)^2 - 5, resulting in 12 - 5, which is 7.

What is the final result of h(g(-6))?

The final result of h(g(-6)) is 7, after substituting -6 into g(x) and then substituting the result into h(y).

Why is it important to understand function composition?

Understanding function composition is important because it allows you to analyze and solve problems involving multiple functions and their interactions.

What advice does the voiceover give for dealing with function composition?

The voiceover advises to take a breath and take it one step at a time when dealing with function composition to avoid confusion.