Composite Function | General Mathematics @MathTeacherGon

Summary

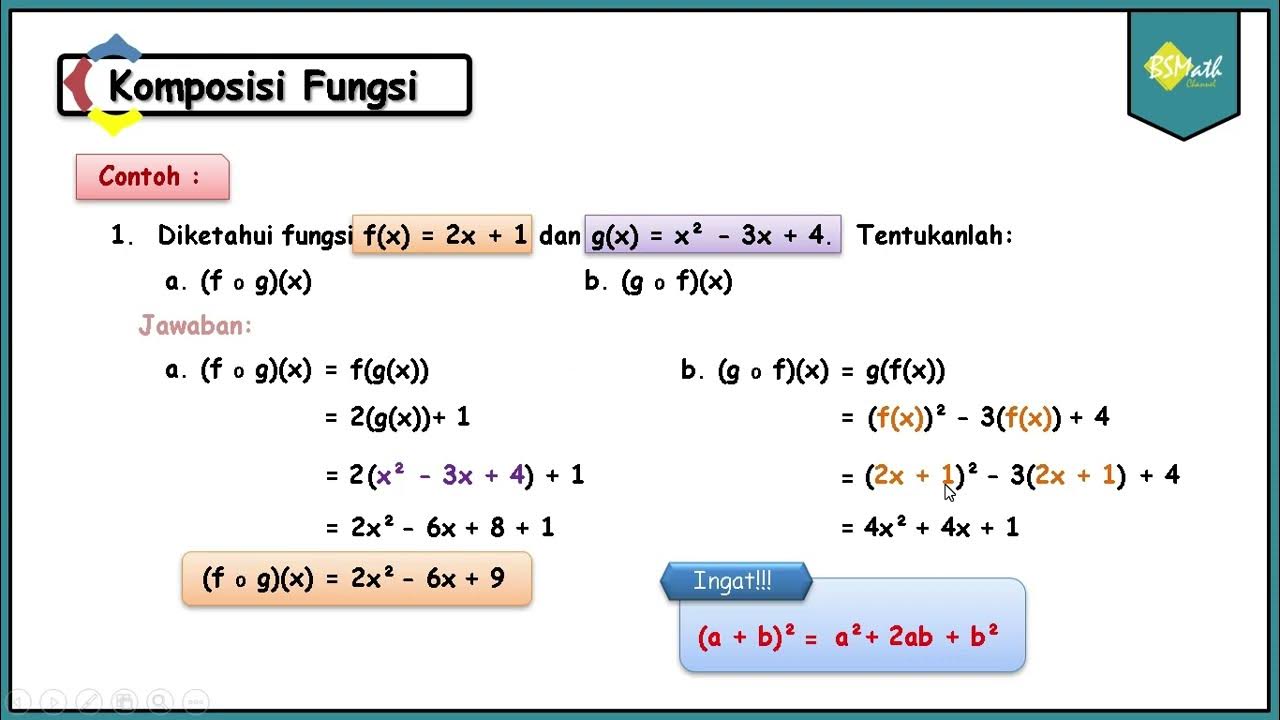
In this educational video, the host, 'Teacher,' delves into the concept of function composition, a topic distinct from operational function evaluation due to its reliance on substitution. The video explains the process using two functions, f(x) = x^2 + 5x + 6 and g(x) = x + 2. Through step-by-step examples, the host demonstrates how to compute f(g(x)), g(f(x)), and specific values like f(g(4)), guiding viewers through the substitution and simplification process. The tutorial is designed to help viewers understand and perform function composition effectively.

Takeaways

• 📚 The video discusses the concept of function composition, which is a method of combining two functions to create a new function.
• 🔢 The functions used as examples are f(x) = x^2 + 5x + 6 and g(x) = x + 2, where f and g are the primary functions being composed.
• 🎯 The video explains how to find the composition of functions, specifically f(g(x)) and g(f(x)), by substituting one function into another.
• 📐 The process involves replacing the variable x in the function with the entire expression of the other function, demonstrating the substitution method.
• 🧮 The video provides a step-by-step calculation for f(g(x)) by substituting g(x) = x + 2 into f(x), resulting in a simplified expression.
• 📉 For g(f(x)), the process is reversed, with f(x) substituted into g(x), leading to another simplified expression.
• 📝 The video emphasizes the importance of simplifying expressions by combining like terms and using algebraic properties.
• 📈 An example with a specific value (f(g(4))) is used to demonstrate how to evaluate the composition of functions at a given point.
• 👨‍🏫 The presenter, Teacher, encourages viewers to practice and understand the concept of function composition, suggesting it's different from evaluating operational functions.
• 📢 The video concludes with a call to action for viewers to like, subscribe, and stay updated with the channel for more educational content.

Q & A

What is the main topic of the video?

The main topic of the video is the composition of functions.

What are the two functions provided in the video?

The two functions provided are f(x) = x^2 + 5x + 6 and g(x) = x + 2.

What does the notation 'f ∘ g' represent?

The notation 'f ∘ g' represents the composition of the function f with the function g, which means applying g first and then f to the result.

How is the composition of functions different from evaluating operational functions?

The composition of functions involves a lot of substitution, where one function is used as the input for another, which is different from evaluating operational functions where you directly substitute the variable.

What is the result of the composition f(g(x))?

The result of the composition f(g(x)) is f(x + 2), which simplifies to x^2 + 9x + 20.

What is the result of the composition g(f(x))?

The result of the composition g(f(x)) is g(x^2 + 5x + 6), which simplifies to x^2 + 5x + 8.

What is the value of f(g(4))?

The value of f(g(4)) is 72, after evaluating g(4) to get 6 and then substituting 6 into f(x) to get f(6).

What is the process for evaluating a composition of functions?

The process involves substituting the inner function into the outer function, simplifying the expression, and then evaluating if a specific input is given.

What is the significance of the distributive property in the context of the video?

The distributive property is significant as it is used to expand and simplify the expressions when substituting and evaluating the compositions of functions.

How does the video script guide viewers through the process of function composition?

The video script guides viewers step-by-step through the process of function composition by providing clear examples and explanations of how to substitute and simplify expressions.

Outlines

00:00

📘 Introduction to Composition of Functions

This paragraph introduces the concept of function composition, a topic that is distinct from evaluating operational functions due to the extensive use of substitution. The teacher promises to explain the process thoroughly. The functions given are f(x) = x^2 + 5x + 6 and g(x) = x + 2. The composition f(g(x)) is explained, where g(x) is substituted into f(x), resulting in f(g(x)) = f(x + 2). The process involves substituting x with (x + 2) in the function f(x), leading to a new expression which is then simplified using algebraic methods. The final simplified form of f(g(x)) is x^2 + 9x + 20.

05:00

🔢 Function Composition: g(f(x)) and Specific Value Example

The second paragraph continues the discussion on function composition but focuses on g(f(x)) and includes an example with a specific value. It starts with explaining g(f(x)) by substituting f(x) into g(x), which results in g(x^2 + 5x + 6). The substitution leads to a new expression that simplifies to x^2 + 5x + 8. The paragraph then presents an example where the composition of functions is evaluated at a specific input, x = 4. The process involves first evaluating g(4), then substituting this result into f(x) to get f(g(4)). The final result of f(g(4)) is calculated to be 72, showcasing a step-by-step approach to evaluating function compositions at specific values.

10:01

👋 Conclusion and Channel Engagement

The final paragraph is a brief conclusion where the teacher signs off with a goodbye. It also serves as a call to action for viewers, encouraging them to like and subscribe to the channel for updates on the latest uploads. The teacher, identified as 'turgon', uses this opportunity to remind viewers of the channel's purpose and to engage with the audience.

Keywords

💡Composition of Functions

The composition of functions is a mathematical operation that involves applying one function to the result of another. In the video, this concept is central as the teacher explains how to perform operations like f(g(x)) and g(f(x)), which are examples of function composition. The video demonstrates how to substitute the output of one function as the input for another, showcasing this with specific functions f(x) and g(x).

💡Function

A function in mathematics is a relation between a set of inputs and a set of permissible outputs, with the property that each input is related to exactly one output. In the script, functions f(x) = x^2 + 5x + 6 and g(x) = x + 2 are given as examples. The video discusses how to manipulate these functions to understand their compositions.

💡Substitution

Substitution is a method used in mathematics where one expression is replaced with another to simplify or transform an equation. The video script heavily features substitution, particularly when explaining the composition of functions. For instance, when finding f(g(x)), the value of g(x) is substituted into f(x), as seen when x + 2 is substituted into f(x) to get f(x + 2).

💡Squaring

Squaring is the mathematical operation of multiplying a number by itself. In the context of the video, squaring is used when the teacher expands expressions like (x + 2)^2 during the composition of functions. This operation is part of simplifying the composed function f(g(x)) where g(x) is x + 2.

💡Distributive Property

The distributive property is a fundamental property in arithmetic that states a(b + c) = ab + ac. The video mentions this property when simplifying expressions that result from function composition. For example, when expanding (x + 2)^2, the teacher uses the distributive property to express it as x^2 + 4x + 4.

💡Like Terms

Like terms in algebra are terms that have the same variables raised to the same power. The video script refers to combining like terms when simplifying the results of function compositions. For instance, when simplifying the expression x^2 + 4x + 5x + 6, the script shows how to combine the like terms 4x and 5x.

💡Evaluation

Evaluation in the context of functions refers to finding the value of a function for a specific input. The video includes an example of evaluating a composed function at a specific point, such as f(g(4)). The teacher demonstrates how to compute this by first finding g(4) and then substituting that value into f(x).

💡Operational Functions

Operational functions, as mentioned in the video, likely refer to functions that involve operations like addition, subtraction, multiplication, and division. The script contrasts these with compositional functions, indicating that operational functions are simpler as they don't involve the complex substitution characteristic of function composition.

💡Binomial

A binomial is an expression composed of two terms, typically in the context of algebra. The video script uses the term 'binomial' when discussing the expansion of expressions like (x + 2)^2, which is a binomial squared. The teacher explains how to apply the formula for squaring a binomial to simplify the expression.

💡Domain

In mathematics, the domain of a function is the set of all possible input values (x-values) for which the function is defined. While not explicitly detailed in the script, the concept of domain is implicitly relevant when discussing function composition, as it affects the possible values that can be substituted into the functions.

Highlights

Introduction to the composition of functions and its difference from evaluating operational functions.

Explanation of the composition method or pattern of g(f(x)) and f(g(x)).

Definition of the given functions f(x) = x^2 + 5x + 6 and g(x) = x + 2.

Step-by-step process to find f(g(x)) by substituting g(x) into f(x).

Simplification of the expression f(g(x)) by expanding and combining like terms.

Final expression for f(g(x)) which is x^2 + 9x + 20.

Process to find g(f(x)) by substituting f(x) into g(x).

Simplification of g(f(x)) resulting in the expression x^2 + 5x + 8.

Explanation of how to handle specific values in composition, using f(g(4)) as an example.

Evaluation of g(4) to find the input for f(x).

Substitution of the value from g(4) into f(x) to find f(g(4)).

Final calculation of f(g(4)) which results in the value 72.

Emphasis on the importance of understanding the composition of functions for those new to the topic.

Encouragement for viewers to like and subscribe to the channel for updates on similar educational content.

Closing remarks by the teacher, signing off with a friendly tone.

Transcripts

hi guys hi guys hi guys hi guys hi guys

it's me teacher

in this video

hi guys it's me teacher going in our

today's video we will talk about the

composition of function

so

this topic

but i will try my best to deliver this

one namaste

because in compositional function this

one is quite different from evaluating

an operational functions because she

will do a lot of

substitution here so without further ado

let's do this topic guys so we have here

the home position of function in general

formation method or pattern atom

of g of x so these two guys i will

explain first

f

g so get the information

on your i

g

of

we have here the given functions f of x

is equal to x squared plus five x plus

six

and then g of x is equal to x plus two

we are given two functions and then we

need to try these three examples

we have here f compose of g

of x so facility input

f

so

we have f

of g

time

f

of g

of x

so copy that into

f

of g

of x where in again

your function g is the input so what we

will do here is we will

get this value because g of x is equal

to x plus 2 since they are equal we can

replace it by x plus 2 so it will become

f

of

f

of x plus 2

we will use x plus 2 as our input

satin expression okay as a function f of

function f this is your function f atom

again input and icg or x plus 2 it will

become x squared

plus 5 x plus 6 again this is your g of

g of x y

so what we will do is we'll replace all

the x variable here and it will become

x plus 2

squared so considering your x naught n

squared negative x plus 2 squared

tables plus 5 times x plus two

plus six

and ito

we can simplify this

by

square binomial that will express the

squared data is the same as

x squared

plus four x

plus four and shortcut and now guys

perpendicular method theta determined by

distributive property it will become

plus five x

equal five times 2 plus 10

plus 6

okay

now

for this time domain guys combine like

terms you have x squared

beta you have plus 9x because 4x sub 5x

for the constant we have 4 plus 10 that

is

14 plus 6 that will give you

the answer of 20. if you said

i'm adding

f

of x plus 2 is equal to x squared plus 9

x plus 20 or eternity

we have

f

of g

of x so again

we have x squared

plus

nine x plus one e okay so brighten up

now let's move on with item number two

for item number two we are given g of f

of x

okay basically information here if you

have g

of f

of x

that is this one i said g that's another

parenthesis c function f

now

since your adding input is f of x

it will become

g

of x squared

plus five x

plus six eternal initial

plus 5x plus six

is equal to and copenhagen function g

x plus two hang on again this is your

uh this is your x and you know the input

and

so we will replace x by x squared plus

five x plus six

that will become x squared

plus five x

plus six and then plus

two and simplifying this your g of x

squared plus five x

plus six

is equal to

x squared

plus five x

plus this is eight

and capacition it up so we will write

here our answer which is x squared

plus five x

plus e

for number one number two second one

as a basic fine given function

i'm again

lots of exponents you do not know

how to perform operations

so let's continue let's try having

item number three writer number three

this one is quite different because

our input here we have a specific value

for our input which is four we're all

going for

we have

f

compose g or f circle g of four

and that is the same as

f

and this log means

of

4 okay

so what we will do here is we will

try to announce try to evaluate g

function g so

g

of 4

is equal to

x plus 2. this is

and your g of four

is simply

four plus two

or in other words this is

g of four

is equal to six

methanol in geo format

okay so

cf

of g

again

uh in g of four negative divided by six

because they are equal top was

contaminated function at the

x squared plus five x

plus six atom in six metal gallium satin

geo four we will replace excess here by

six it will become

six x six squared

plus five times six

plus six

okay so

guys

we have here 36

plus 30 plus 6. but in advan your f of 6

is equal to 36 plus

30 this is 66 plus 6 this will give you

72. in this opinion guys if f of 6 is

equal to 7 2 your value num

f of g

of 4 is equal to 72.

so i'm going to show you guys on how to

do compositional functions so

on how to do composition of functions so

again if you are new to my channel don't

forget to like and subscribe but hit

like button for you to be updated at the

latest uploads again it's me turgon

bye