Bernoulli's Principle Derivation
Summary
TLDRThis educational video explains Bernoulli’s Principle, which describes how fluid pressure changes with changes in speed and height. The instructor takes the audience through a step-by-step derivation using an ideal fluid flow in a pipe. Key concepts like pressure, speed, and gravitational forces are explored, with the work-energy principle applied to derive Bernoulli’s equation. The equation is shown to reflect the conservation of energy in fluid flow along a streamline. The video concludes with a promise of upcoming demonstrations to visualize Bernoulli's Principle in real-world applications.
Takeaways
- 😀 Bernoulli’s Principle describes how pressure in a fluid changes as its speed and height above the Earth’s surface change. It was first established by Daniel Bernoulli in the 18th century.
- 😀 The script discusses an ideal fluid flow through a pipe with changing diameter and height, assuming the fluid is incompressible, nonviscous, and follows laminar flow.
- 😀 The flow system is represented by two planes (plane 1 and plane 2) within the pipe, and the cross-sectional area at these planes plays a crucial role in determining the fluid's speed.
- 😀 The principle of conservation of volumetric flow rate is emphasized: the product of cross-sectional area and speed is constant across the system, meaning that the speed increases where the pipe diameter decreases.
- 😀 The work done by pressure forces and gravity are essential in deriving Bernoulli’s equation. Work is calculated by multiplying force by displacement, and this energy transformation relates to changes in kinetic energy.
- 😀 Three main forces are identified in the system: the pressure from the fluid to the left of plane 1, the pressure from the fluid to the right of plane 2, and the force of gravity affecting the fluid's height change.
- 😀 The force of gravity does work on the system as the fluid changes its vertical height, which is an important part of the energy equation that leads to Bernoulli’s Principle.
- 😀 The script illustrates how the work done by gravity is independent of the path taken, and it's only dependent on the change in vertical height of the fluid volume.
- 😀 The change in kinetic energy of the fluid is represented by the difference in the kinetic energies at plane 1 and plane 2, with mass and velocity factors incorporated into the equation.
- 😀 Bernoulli’s equation, derived from conservation of mechanical energy, describes the relationship between pressure, fluid speed, and height in a streamline, and it applies only to specific points along a streamline, not the entire pipe or over a period of time.
Q & A
What is Bernoulli's Principle?
-Bernoulli's Principle states that in a fluid flow, as the speed of the fluid increases, the pressure decreases, and vice versa. This principle was formulated by Daniel Bernoulli in the 18th century.
What are the assumptions made in the derivation of Bernoulli's Principle?
-The derivation assumes that the fluid is incompressible, nonviscous, and the flow is laminar and irrotational. Additionally, the flow is considered ideal, with no energy loss.
What is meant by 'ideal fluid flow'?
-Ideal fluid flow refers to the flow of a fluid that is incompressible (its density does not change), nonviscous (no internal friction), and exhibits laminar and irrotational flow patterns.
How does the cross-sectional area of the pipe affect the fluid speed?
-According to the continuity equation, the speed of the fluid increases when the cross-sectional area of the pipe decreases. This is because the volumetric flow rate remains constant.
What forces are acting on the fluid in the system?
-The three main forces acting on the fluid are the pressure from the fluid to the left of plane 1 (pushing the fluid to the right), the pressure from the fluid to the right of plane 2 (pushing the fluid to the left), and the force of gravity (affecting the vertical displacement of the fluid).
What does the Net Work equals Change in Kinetic Energy theorem imply in this context?
-This theorem states that the work done on a system is equal to the change in its kinetic energy. In the context of Bernoulli's Principle, it is used to relate the work done by the forces on the fluid to its change in kinetic energy.
What role does gravity play in the derivation of Bernoulli’s Principle?
-Gravity does work on the fluid as it moves through different heights. The change in height above the horizontal zero line causes a change in the gravitational potential energy, which contributes to the overall work-energy equation.
How is the work done by gravity calculated in this system?
-The work done by gravity is calculated as the product of the fluid's mass, the gravitational field strength, and the vertical change in height (delta y). This work is negative, as gravity acts in the opposite direction of the fluid's displacement.
What is Bernoulli's equation, and what does it describe?
-Bernoulli's equation is: P + (1/2)ρv² + ρgh = constant, where P is pressure, ρ is fluid density, v is velocity, g is gravitational acceleration, and h is height. It describes the relationship between pressure, velocity, and height in a fluid flow along a streamline.
Why is Bernoulli's equation only valid along a streamline?
-Bernoulli’s equation applies to specific points along a streamline because it assumes steady, ideal flow. The assumptions break down in regions of turbulent flow or where external forces affect the system, making it only valid in regions where the flow is smooth and steady.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级浏览更多相关视频

7 Fun Demos of Bernoulli’s Principle Explained
Mekanika Fluida FM01 (Lecture3: 6/8). Venturimeter - Aplikasi persamaan Bernoulli
Physics 34 Fluid Dynamics (1 of 7) Bernoulli's Equation
Bernoulli's Equation for Fluid Flow Video in Physics
Fluida Dinamis • Part 4: Venturimeter / Pipa Venturi
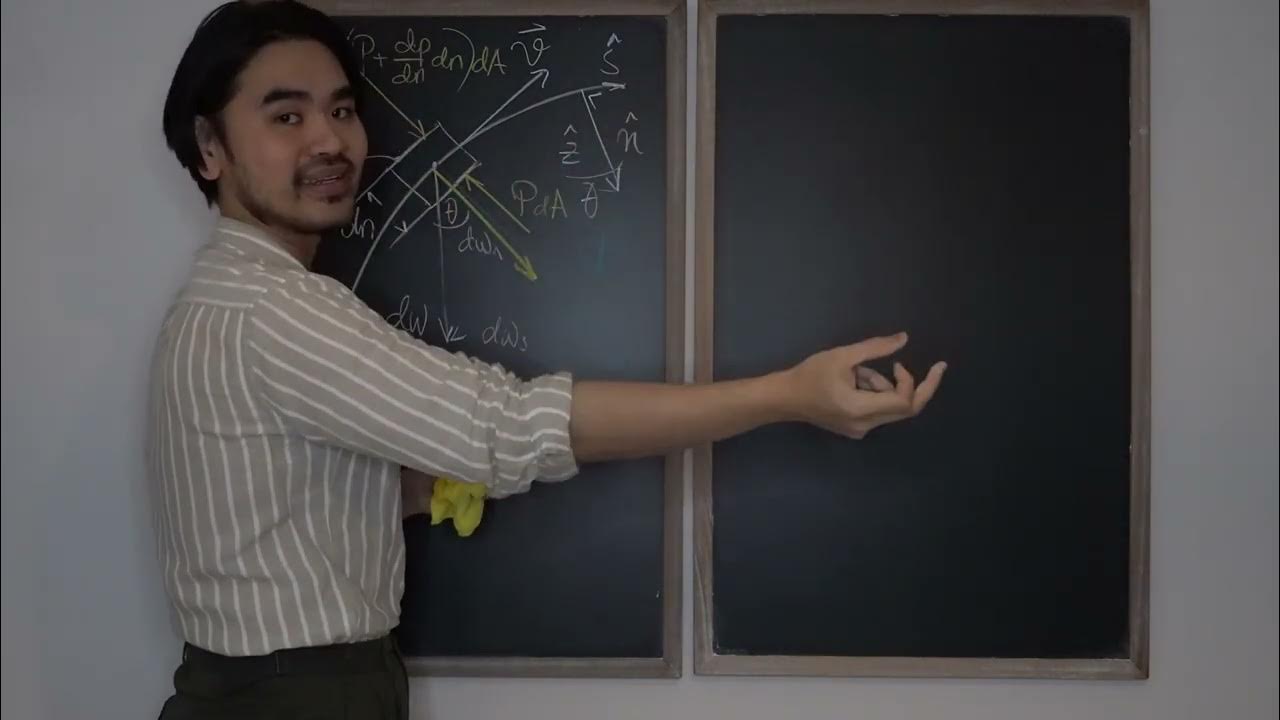
Mekanika Fluida FM01 (Lecture3: 3/8). Pressure across streamline
5.0 / 5 (0 votes)
