Physics 34 Fluid Dynamics (1 of 7) Bernoulli's Equation

Summary

In this video, the speaker introduces Bernoulli's equation in fluid dynamics, explaining how pressure, velocity, and height are interconnected in fluid flow, particularly through pipes. Using practical examples, the speaker demonstrates how changes in height and velocity impact pressure within the system. A specific calculation is presented where the pressure at point 2 in a pipe is calculated, given a height change. The speaker walks through the steps of applying Bernoulli’s equation and unit conversions to arrive at the pressure result. This engaging explanation offers insights into fluid dynamics and its real-world applications.

Takeaways

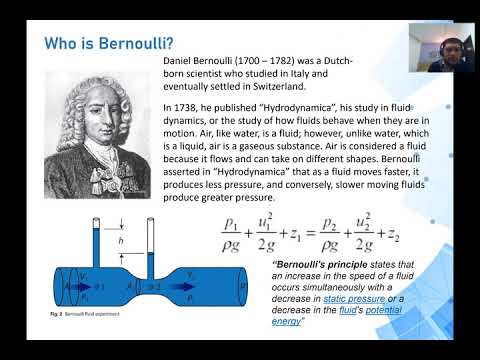
• 😀 Bernoulli's equation relates pressure, velocity, and height of a fluid and states that these three factors are interconnected.
• 😀 The equation is given as: P + ρgh + ½ρv² = constant, where P is pressure, ρ is density, g is acceleration due to gravity, h is height, and v is velocity.
• 😀 The equation shows that when one of the three factors (pressure, velocity, or height) increases, the others must adjust to maintain a constant total.
• 😀 Bernoulli’s equation is commonly applied to fluid flow through pipes but can be used in other fluid flow scenarios as well.
• 😀 If the height of a fluid increases, the pressure must decrease in order to keep Bernoulli's equation balanced.
• 😀 In a pipe with a constant diameter, the velocity of fluid remains constant at different points, as shown by the equation A1V1 = A2V2, where A is the cross-sectional area.
• 😀 If velocity does not change, and height increases, the pressure decreases due to the relationship in Bernoulli's equation.
• 😀 An example problem shows how to calculate the pressure at a second point in a pipe where the height increases, using Bernoulli’s equation and given values like fluid density, gravity, and velocity.
• 😀 The formula to find the pressure change is: P2 = P1 + ρg(h1 - h2), where h1 and h2 are the heights at points 1 and 2 respectively.
• 😀 In the example, pressure at point 2 was calculated to be 1.52 atmospheres, considering a height difference of 5 meters in the pipe.

Q & A

What is Bernoulli's equation used for?

Bernoulli's equation is used to relate the pressure, velocity, and height of a fluid in motion, typically in a pipe, and explains how changes in one factor influence the others to maintain a constant sum.

What are the key components of Bernoulli's equation?

The key components of Bernoulli's equation are pressure, velocity (kinetic energy), and height (potential energy) of the fluid.

How does the velocity of a fluid change in a pipe when the height changes, according to Bernoulli's equation?

If the height increases in the pipe, the velocity of the fluid must decrease, assuming the flow area (diameter of the pipe) remains constant, to keep the equation balanced.

In the example where the pipe height changes from 5 meters to 10 meters, how does this affect the pressure?

As the height increases from 5 meters to 10 meters, the pressure at the second point decreases, as shown by the calculation that results in a pressure difference of 0.48 atmospheres.

What is the significance of the term 'row g h' in Bernoulli's equation?

'row g h' represents the potential energy per unit volume due to gravity, where 'row' is the fluid density, 'g' is the acceleration due to gravity, and 'h' is the height of the fluid above a reference point.

Why does the pressure change when the height of the pipe changes, according to Bernoulli's principle?

According to Bernoulli’s principle, if the height increases, the potential energy increases, and to maintain the constant sum of pressure, velocity, and height, the pressure must decrease.

What assumptions are made in the example about the pipe and fluid flow?

In the example, it's assumed that the fluid is incompressible, the pipe's diameter does not change, and the fluid velocity remains constant throughout the pipe.

How is the pressure difference calculated in the example with water flowing through the pipe?

The pressure difference is calculated by using Bernoulli's equation, where the pressure at the second point is equal to the pressure at the first point, adjusted by the difference in height (H1 - H2), and taking into account the fluid's density and gravitational acceleration.

What is the significance of the term '12 row v^2' in Bernoulli's equation?

'12 row v^2' represents the kinetic energy per unit volume of the fluid, where 'row' is the density of the fluid and 'v' is the velocity of the fluid.

How do you convert the pressure from Newtons per square meter to atmospheres in the example?

To convert the pressure from Newtons per square meter (Pascals) to atmospheres, divide the pressure in Pascals by the value of one atmosphere in Pascals, which is 101,300 N/m².