Percentiles (1.7)

Summary

The video script explains the concept of percentiles in statistics, illustrating how they represent the distribution of data points. It uses an example of a class taking a math test to clarify that the 50th percentile, or median, means half the scores are below or equal to it. The script also distinguishes between percentiles and percentages, noting that a score of 31 equates to 62 percent but roughly places a student in the 23rd percentile. It emphasizes the importance of understanding percentiles for data interpretation, while acknowledging the variability in their calculation.

Takeaways

• 📊 A percentile is a statistical measure that indicates the value below which a given percentage of observations in a group of observations fall.
• 🔢 The 50th percentile, or median, is the middle value in a data set where 50% of the data points are lower and 50% are higher.
• 📚 To determine percentiles, data must be numerically ordered.
• 👥 In a class example with 15 students, the median score represents the 50th percentile, meaning half the class scored below and half scored above this value.
• 🏁 The 'small or smaller' and 'large or larger' parts of the definition emphasize the inclusivity of the data point itself when calculating percentiles.
• 🔄 The 50th percentile is not merely about half the data points being smaller; it includes the data point itself as part of the 'smaller' group.
• 📈 Quartiles are also percentiles; for instance, the third quartile (Q3) is the 75th percentile, where 75% of data points are below or equal to this value.
• 💯 Scoring in the 75th percentile means outperforming 75% of the class.
• 🚫 Percentage and percentile are not interchangeable; a score of 62% does not equate to being in the 62nd percentile.
• 🤔 Calculating a percentile can vary in method and thus may yield slightly different results, but for the course, specific calculation methods are not required.
• 📝 Understanding the concept of percentiles is crucial for interpreting statistical data correctly and not confusing it with raw percentages.

Q & A

What is a percentile and how does it relate to data values?

A percentile describes the percentage of data values that fall at or below a specific data value. It helps to understand the relative standing of a particular data point within a dataset.

How do you determine the 50th percentile in a dataset?

The 50th percentile, also known as the median, is the middle value of a dataset when it is ordered numerically. It divides the dataset into two equal halves, with 50% of the data points being as small or smaller, and 50% as large or larger.

Why is it important to include 'as small or smaller' when describing the 50th percentile?

Including 'as small or smaller' ensures that the median itself is counted in the 50% of data points that are on the lower side. Without this, the statement would inaccurately imply that only data points less than the median are considered.

What is the relationship between the 50th percentile and the data point at that percentile?

The 50th percentile is the actual data point that has 50% of the dataset values below it and 50% above it, including the point itself.

How is the 75th percentile, also known as the third quartile, described in terms of data points?

The 75th percentile is a value below which 75% of the data points fall, meaning 75% are as small or smaller, and 25% are as large or larger.

What does it mean if someone scored in the 75th percentile on a test?

Scoring in the 75th percentile means that the individual has performed better than 75% of the class or group taking the test.

Why should one not confuse percentage with percentile?

Percentage refers to a proportion of a whole, while percentile ranks a data point relative to others. A score of 62% does not equate to the 62nd percentile, as these are fundamentally different measures.

How might the calculation of a percentile vary and lead to different answers?

Different methods of calculating percentiles, such as interpolation, can yield slightly different results. However, for the purposes of a specific course, the exact method may not be necessary to know.

Why might a score of 31 on a test be considered roughly the 23rd percentile instead of the 62nd?

The score of 31 corresponds to the 23rd percentile because it places the individual below approximately 77% of the class, not 62%. The misconception arises from confusing raw score percentage with the relative standing percentile.

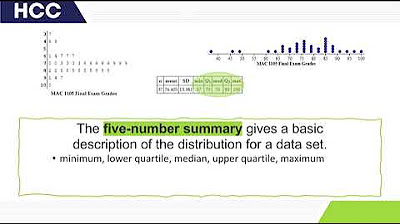
What is the significance of quartiles (Q1 and Q3) in understanding a dataset?

Quartiles divide the dataset into four equal parts, with Q1 being the 25th percentile and Q3 the 75th percentile. They provide insights into the distribution and spread of the data beyond just the median.

How can understanding percentiles help in interpreting test scores or performance?

Percentiles provide a way to compare individual performance relative to a group, offering a clearer picture of how one stands in comparison to peers, which can be more informative than raw scores alone.