09-05 Tegangan geser pada balok
Summary
TLDRThis lecture discusses shear forces and shear stress in beams under various loads. The instructor explains the relationship between shear force (V), normal stress (σ), and how these stresses impact the beam's cross-section. It delves into the complexities of shear stress distribution, particularly in beams experiencing bending moments. The script also explores how to calculate shear stress by considering factors like the moment of inertia and cross-sectional geometry. Emphasis is placed on understanding how forces are balanced and distributed within structural elements, providing an essential foundation for analyzing shear in beam design.
Takeaways
- 😀 Shear force (V) in beams leads to shear stress, which is different from the normal stress discussed earlier, and is calculated using the area of the cross-section.
- 😀 For beams under bending, the deformation must ensure that the cross-section remains flat without any part moving further than another.
- 😀 Shear stress is calculated by considering the internal forces, which are influenced by the applied loads and distributed across the cross-section.
- 😀 When dealing with shear in beams, a segment of the beam is taken to analyze the shear force, assuming equilibrium is maintained.
- 😀 Shear stress distribution varies along the height of the beam's cross-section, with the maximum shear stress typically occurring at the neutral axis.
- 😀 To calculate shear stress, the change in bending moment (ΔM) is considered over a small segment (ΔX) to determine the effect of shear force over that length.
- 😀 The relationship between shear force and bending moment is important for understanding the distribution of shear stress in the beam.
- 😀 Shear stress can be computed using the formula involving the moment of inertia (I) of the beam's cross-section and the distance from the centroid to the point of interest.
- 😀 The shear force V is often used directly to compute shear stress using formulas derived from the distribution of the bending moment and the geometry of the beam.
- 😀 Calculating shear stress requires careful attention to the beam’s geometry, including the cross-sectional area, moment of inertia, and the position where the stress is being measured.
Q & A
What is shear force (V) in the context of the beam analysis?
-Shear force (V) refers to the internal force that acts along the cross-section of a beam due to external loads. It causes the material to experience shear stress, which leads to a sliding motion of particles along the plane of the section.
How is shear stress calculated for a beam under shear force?
-Shear stress is calculated by dividing the shear force (V) by the cross-sectional area (A) of the beam. The formula is: Shear Stress = V / A.
What is the significance of the normal stress (Sigma) in beam analysis?
-Normal stress (Sigma) is the stress acting perpendicular to the cross-section of the beam, caused by axial forces like tension or compression. It's calculated using the formula: Sigma = Force / Area.
What does the term 'shear flow' (Q) refer to in the analysis?
-Shear flow (Q) is a measure of how the shear force is distributed across the beam's cross-section. It is calculated using the moment of inertia and the first moment of area relative to the section’s centroid.
Why is the deformation of a beam due to shear force important in structural analysis?
-The deformation due to shear force affects the beam's overall stability and performance. It’s essential to calculate accurately to ensure that the material's integrity is maintained and that no excessive deformation occurs.
What is the difference between shear stress and normal stress in a beam?
-Shear stress acts parallel to the beam’s cross-section and results from shear force, while normal stress acts perpendicular to the cross-section and is caused by axial forces like tension or compression.
How does the moment of inertia affect shear stress in a beam?
-The moment of inertia (I) influences the distribution of shear stress across the beam's cross-section. A higher moment of inertia typically leads to lower shear stress, as it resists the deformation caused by the shear force.
Why is the first moment of area important in shear force analysis?
-The first moment of area (Q) is critical because it helps determine how the shear force is distributed across the beam’s cross-section. It is used in the calculation of shear stress in non-uniform cross-sections.
How do you calculate the shear stress at a specific point in the beam?
-To calculate the shear stress at a specific point, you need to find the shear force at that point (V), the area of the section above or below the point (A), and the moment of inertia (I). The formula is: Shear Stress = (V * Q) / (I * b), where b is the width of the section at the point of interest.
What role does beam geometry play in shear stress calculations?
-Beam geometry, including the shape and size of the cross-section, directly affects the shear stress distribution. The larger the cross-sectional area and the moment of inertia, the lower the shear stress for a given shear force.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Understanding Shear Force and Bending Moment Diagrams
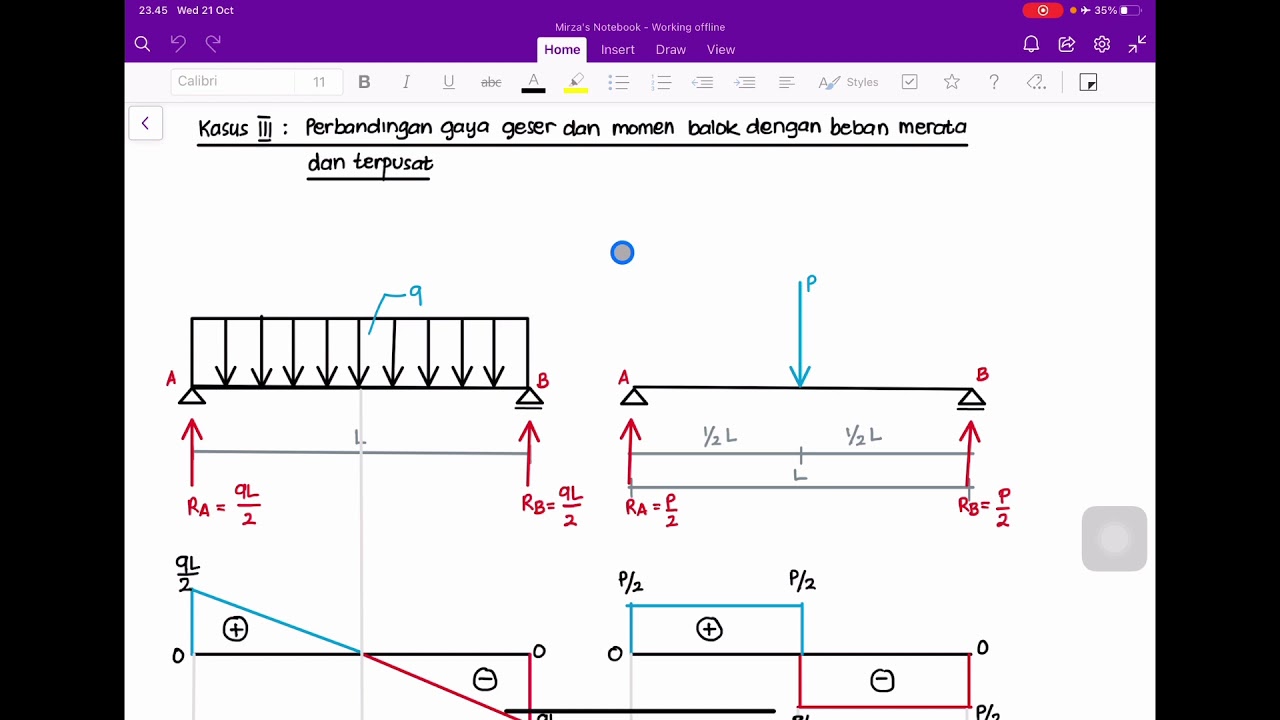
Mekanika Statis Tentu: Perbandingan Gaya Geser Dan Momen Pada Balok Dengan Beban Merata Dan Terpusat
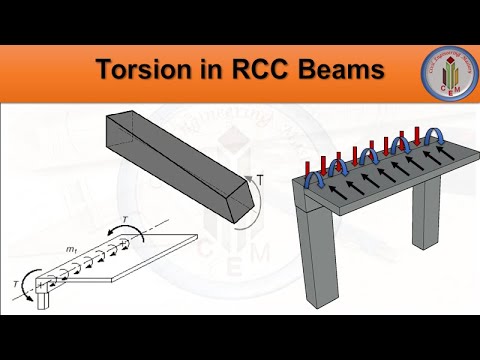
Torsion in Beams | Twisting moment in RCC beams |Primary & Secondary Torsion |IS-456:2000 provisions
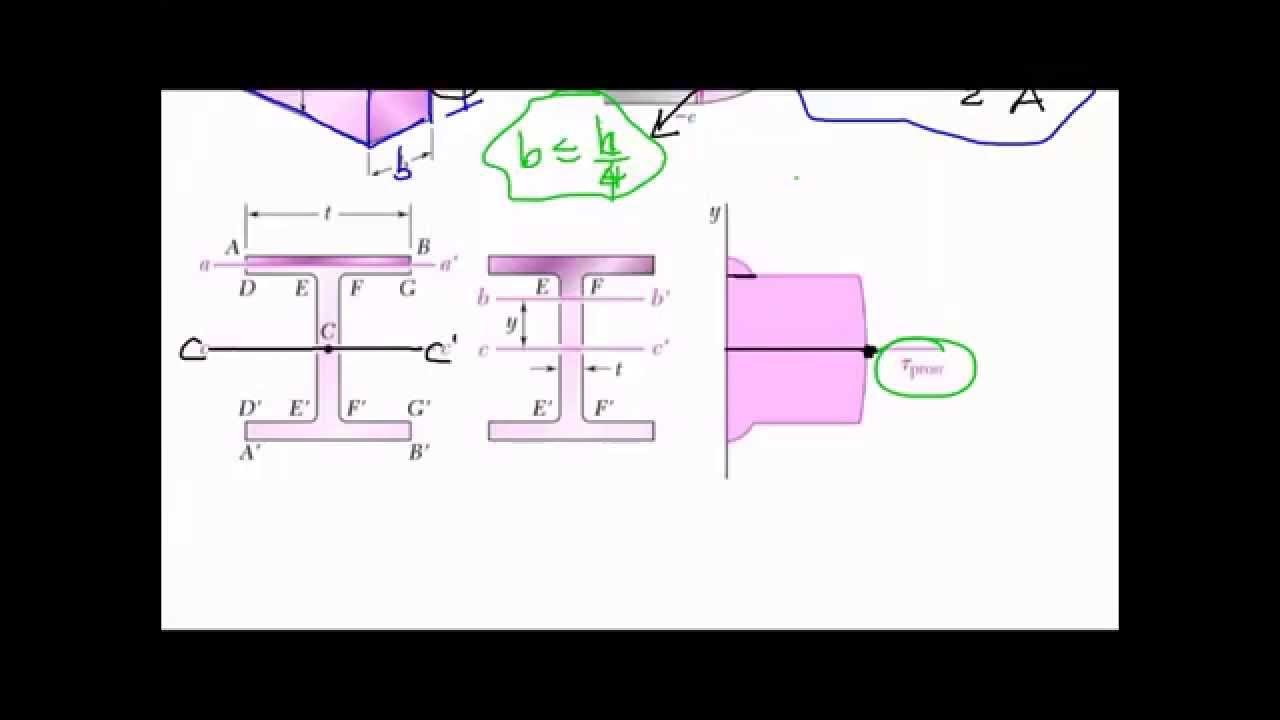
Resistencia de Materiales: Concepto de esfuerzos cortantes en vigas

Understanding Stresses in Beams

Statika/Mekanika Teknik #4: Gaya Dalam
5.0 / 5 (0 votes)