Resistencia de Materiales: Concepto de esfuerzos cortantes en vigas
Summary
TLDRThis video explains the distribution of shear forces in prismatic beams, particularly focusing on rectangular and standard-profile beams. It discusses the concept of shear force variation along the beam, explaining the maximum shear force at the center for rectangular beams. The video highlights the importance of calculating shear force using different formulas depending on beam shape and dimensions. For rectangular beams, a simplified equation is provided, while for standard-profile beams, an approximation based on shear force over the web area is suggested. The video aims to make shear force calculations easier by explaining key relationships and equations.
Takeaways
- 😀 Shear stresses in prismatic beams are distributed differently across the beam's length, with the maximum shear stress occurring at the center and decreasing towards the edges.
- 😀 Prismatic beams can be analyzed by cutting through them and studying the shear forces acting on a section of the beam.
- 😀 There are two primary types of shear stresses: vertical shear (denoted as τ_x) and horizontal shear (denoted as τ_y). Both are present in prismatic beams and vary across the beam's cross-section.
- 😀 For rectangular beams, the shear stress is calculated using a formula that applies when the beam's width is less than or equal to a quarter of its height.
- 😀 The maximum shear stress in a rectangular beam occurs at the center, and the distribution of shear stress varies from zero at the top to a maximum value at the center of the beam.
- 😀 If the width of a rectangular beam exceeds a quarter of its height, a different, simplified method must be used to calculate shear stress, which involves the total shear force and the beam's area.
- 😀 For standard-profile beams, such as I-beams or W-beams, shear stress is calculated using the area of the web of the beam, without using complex formulas for shear force distribution.
- 😀 The shear stress in standard-profile beams is highest at the centroid and decreases as the distance from the centroid increases, especially toward the flanges.
- 😀 The simpler method of calculating shear stress for standard-profile beams is to divide the shear force by the area of the web (central vertical section) of the beam.
- 😀 Custom beams or beams with non-standard cross-sections require the general shear formula τ = VQ / It, which takes into account the first moment of area (Q), second moment of area (I), and thickness of the section (t).
Q & A
What is a prismatic beam, and how is it analyzed in the script?
-A prismatic beam is a beam with a constant cross-section along its length. The script explains how the shear forces are distributed along such beams and focuses on the distribution of vertical and horizontal shear stresses within the beam.
How is the shear force distribution visualized for a prismatic beam?
-The shear force distribution is shown graphically, where it is observed that the shear force is greatest at the ends of the beam and decreases toward the center. The shear force at the center is minimal, and the calculation of shear force is complex at specific points, so a simplified average shear force is often used.
What is the significance of the maximum shear force in a rectangular beam?
-The maximum shear force in a rectangular beam occurs at the center of the beam, and it is crucial for structural calculations. The script explains that for rectangular beams, this shear force can be calculated using a specific formula when certain geometric conditions are met.
What is the relationship between the base (B) and height (H) of a rectangular beam for calculating shear force?
-For a rectangular beam, the width of the beam (B) must be less than or equal to one-quarter of the height (H) of the beam to use the simplified formula for calculating the shear force. If this condition is not met, a more general formula must be used.
What is the simplified approach for calculating shear force in beams that do not meet the B/H ratio?
-If the base (B) of the beam is not less than or equal to one-quarter of its height (H), the simplified formula cannot be used. Instead, the shear force is calculated by dividing the total load by the area of the beam's cross-section.
How is the shear force distributed in beams with a non-rectangular cross-section, such as standard profile beams?
-In standard profile beams (like I-beams or W-beams), the shear force is maximum at the centroid of the section and decreases towards the edges. The variation in shear force is shown to increase as the shear stress moves towards the flange of the beam.
What is the maximum shear force for a beam with a non-rectangular profile, and how is it calculated?
-For beams with a non-rectangular profile, such as standard profile I-beams, the maximum shear force is calculated by dividing the load by the area of the 'web' or core of the beam. This is a simpler method compared to using the general shear force equation.
What is the importance of the 'web' area in standard profile beams?
-The 'web' of a standard profile beam refers to the central portion of the beam, excluding the flanges. The area of the web is critical for calculating the shear force in standard profile beams, as the shear force is considered based on this area.
Can the formula for calculating shear force be applied to custom or non-standard beams?
-No, the simplified formulas for calculating shear force apply only to commercial standard profile beams. For custom or non-standard beams, the more complex equation involving the geometry of the cross-section must be used.
What key factors must be considered when calculating the shear force in beams with different cross-sectional shapes?
-The key factors to consider include the beam's cross-sectional shape, the distribution of forces within the beam, and the relationship between the dimensions of the cross-section (such as base and height in rectangular beams). For non-standard beams, the specific geometric properties must be considered, and the appropriate formulas must be applied.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

09-05 Tegangan geser pada balok

Understanding Stresses in Beams
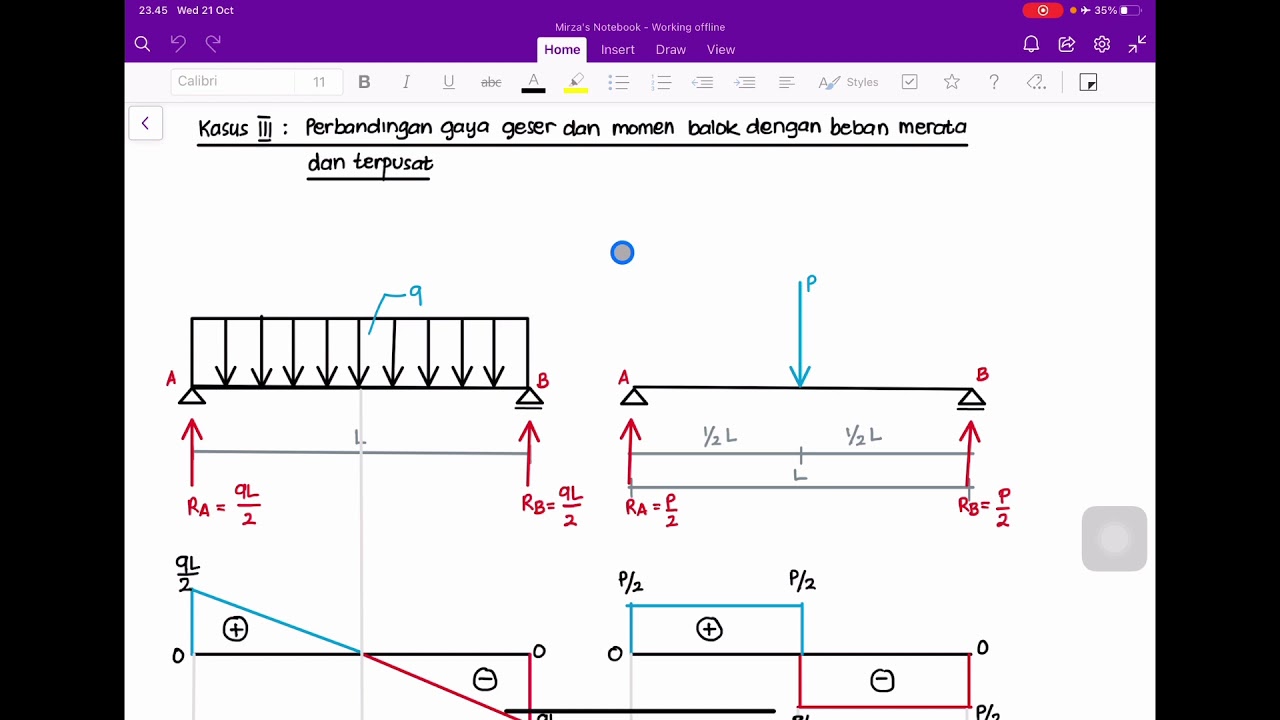
Mekanika Statis Tentu: Perbandingan Gaya Geser Dan Momen Pada Balok Dengan Beban Merata Dan Terpusat

Understanding Shear Force and Bending Moment Diagrams
Torsion in Beams | Twisting moment in RCC beams |Primary & Secondary Torsion |IS-456:2000 provisions

Video #4 Balok Kantilever
5.0 / 5 (0 votes)