Cara Menentukan Fungsi Kuadrat Jika Diketahui Titik Potong Terhadap Sumbu-X

Summary

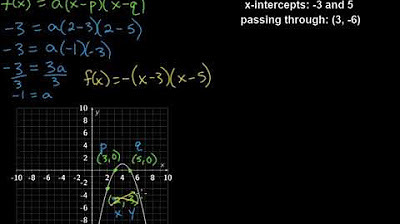
In this video, Mat explains how to find the quadratic function when given two x-intercepts and a point on the curve. He starts by introducing the general form of a quadratic function and demonstrates how to apply a specific formula when the function cuts the x-axis at two points. Mat guides viewers step-by-step, showing how to substitute coordinates into the formula and solve for unknowns. Using an example with x-intercepts at (3, 0) and (-2, 0), and a point at (0, 3), he simplifies the process and finds the quadratic function, offering clear explanations throughout.

Takeaways

• 😀 Quadratic functions are generally expressed in the form of FX = ax² + bx + c.
• 😀 When a quadratic function intersects the x-axis at two points, the function can be factored as FX = a(x - x1)(x - x2).
• 😀 The x-intercepts are the points where the quadratic function crosses the x-axis, often represented as (x1, 0) and (x2, 0).
• 😀 The given problem specifies that the quadratic intersects the x-axis at (3, 0) and (-2, 0), which provides the x1 and x2 values.
• 😀 After identifying the x-intercepts, we substitute these values into the factored form of the quadratic equation.
• 😀 The next step involves using another point on the curve, for example, (0, 3), to solve for the constant 'a' in the equation.
• 😀 The equation is then solved by substituting x = 0 and y = 3 into the factored form of the quadratic function.
• 😀 Once the value of 'a' is determined, it is substituted back into the factored quadratic equation to complete the function.
• 😀 The final quadratic function is then expanded and simplified to obtain the standard form.
• 😀 In this case, after finding 'a' as -1/2, the final quadratic function is FX = -1/2(x - 3)(x + 2).
• 😀 The function can be left in the expanded form or further simplified, but it is not necessary to multiply through if it's not required.

Q & A

What is the general form of a quadratic function?

The general form of a quadratic function is f(x) = ax² + bx + c, where a, b, and c are constants.

How do you write the equation of a quadratic function when two x-intercepts are given?

When two x-intercepts are given, the equation of the quadratic function can be written as f(x) = a(x - x1)(x - x2), where x1 and x2 are the x-intercepts.

What do the terms 'x1' and 'x2' represent in the factored form of a quadratic function?

'x1' and 'x2' represent the x-intercepts or the points where the quadratic function crosses the x-axis.

How do you determine the coefficient 'a' in the quadratic function?

To determine the coefficient 'a', you substitute the coordinates of a known point on the graph (other than the x-intercepts) into the equation and solve for 'a'.

In the given example, what are the x-intercepts of the quadratic function?

The x-intercepts in the example are (3, 0) and (-2, 0). These are the points where the quadratic function crosses the x-axis.

Why is the factor (x - 3) used in the quadratic function equation?

The factor (x - 3) is used because 3 is one of the x-intercepts, meaning the quadratic function crosses the x-axis at x = 3.

What does the point (0, 3) represent in this problem?

The point (0, 3) represents a point on the quadratic curve where the value of y is 3 when x equals 0. This point is used to determine the coefficient 'a'.

How do you handle negative signs in the factored form of the quadratic function?

When you encounter negative signs in the factors, you apply the rule that two negatives multiplied together give a positive result. For example, (x - (-2)) becomes (x + 2).

What is the significance of the factor 'a' in the quadratic function?

The factor 'a' determines the shape and direction of the parabola. If 'a' is positive, the parabola opens upwards, and if 'a' is negative, it opens downwards.

Why is the final quadratic function f(x) = -1/2(x - 3)(x + 2) simplified to f(x) = -1/2x² - x + 3?

The function is simplified by expanding the factored form and then distributing the value of 'a' to each term. The result is the standard form of the quadratic equation, f(x) = -1/2x² - x + 3.