PERBANDINGAN TRIGONOMETRI SUDUT BERELASI

Summary

This educational video explores trigonometric ratios and their relationships across different quadrants. It demonstrates how to calculate sine and cosine values for specific angles (120°, 210°, and 330°) using key trigonometric formulas. The video further explains these values in the context of the unit circle, breaking down each calculation step-by-step. The content also clarifies how certain angles relate to others in different quadrants and emphasizes the importance of understanding positive and negative signs for trigonometric functions in each quadrant. Finally, the video highlights practical examples using right triangles and the Pythagorean theorem to define sine and cosine values.

Takeaways

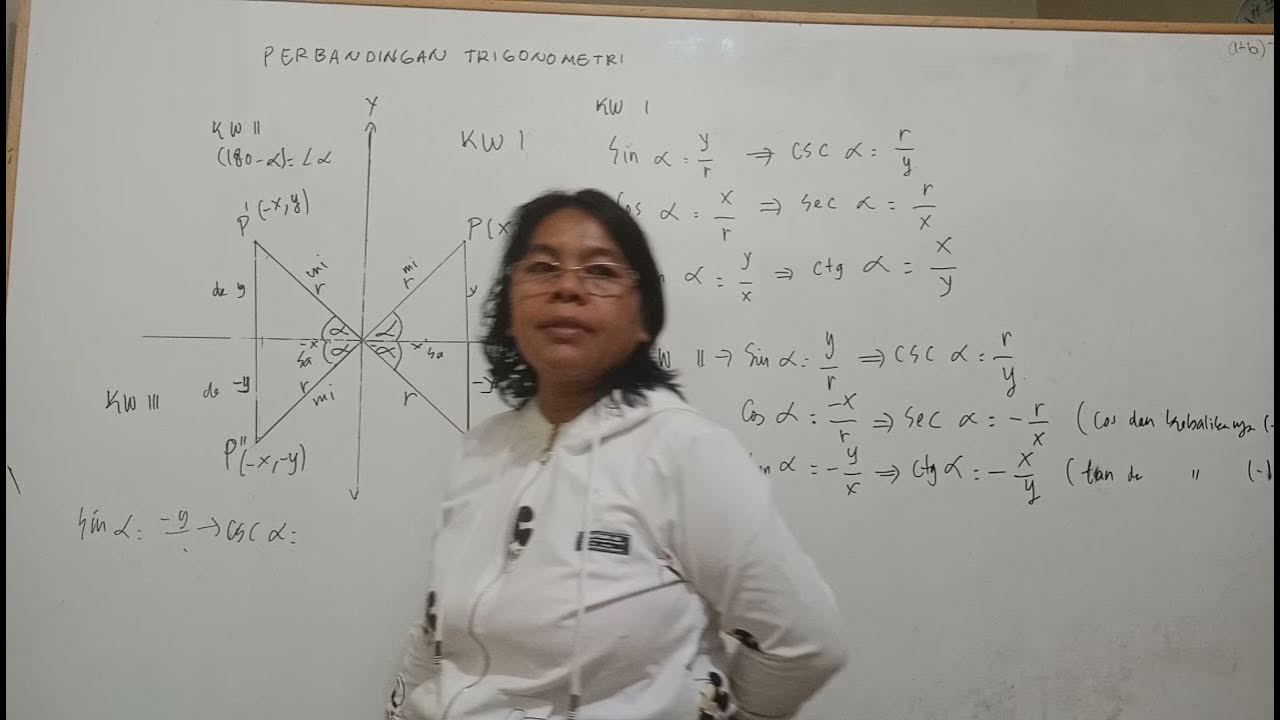
• 😀 There are five special angles in quadrant 1, each having a related trigonometric value.
• 😀 The provided formula helps to easily calculate trigonometric ratios for angles in different quadrants.
• 😀 The formula relies on three key equations in the red boxes, which are crucial for solving trigonometric values.
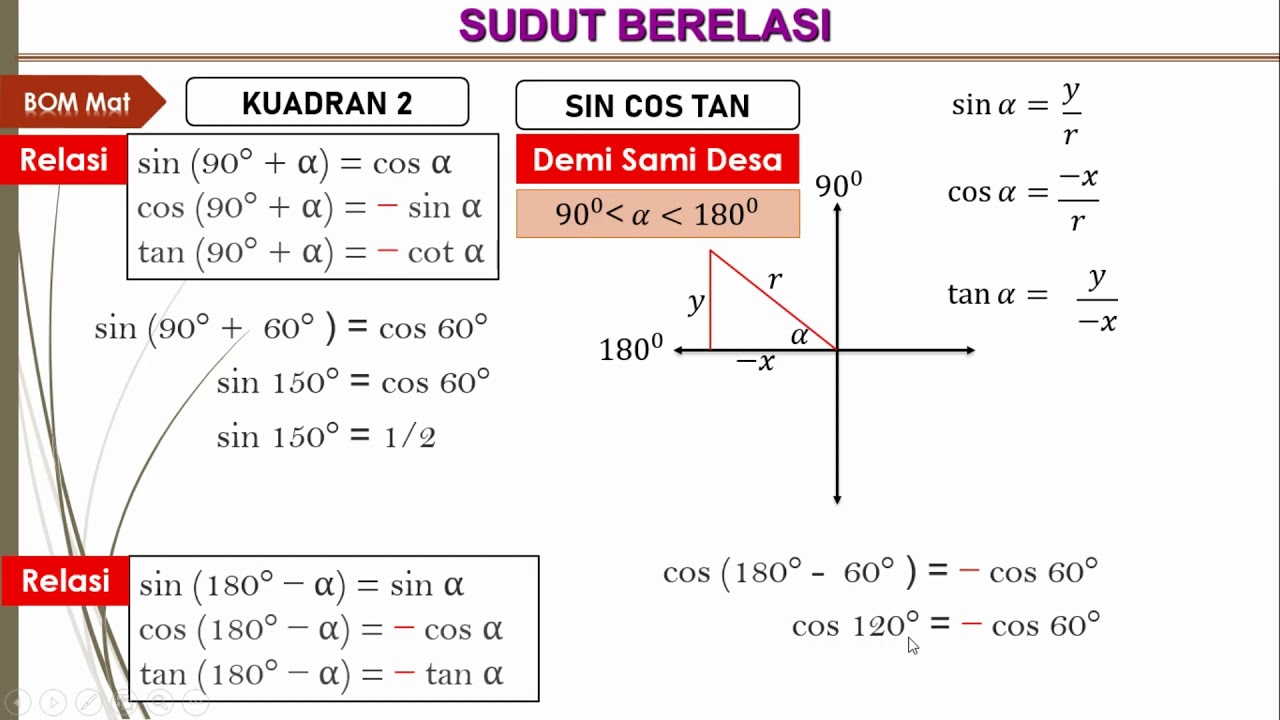
• 😀 To calculate Sin 120°, we use the identity Sin (180° - 60°), which simplifies to Sin 60° with a value of 1/2√3.
• 😀 Cos 210° can be calculated using the identity Cos (180° + 30°), which simplifies to -Cos 30°, with a value of -1/2√3.
• 😀 Sin 330° is derived using the identity Sin (360° - 30°), which simplifies to -Sin 30°, with a value of -1/2.
• 😀 Trigonometric values can be verified using quadrant-based rules: Sin in quadrant 2 is positive, Cos in quadrant 3 is negative, and Sin in quadrant 4 is negative.
• 😀 The definition of Sin 30° is based on the ratio of the opposite side to the hypotenuse in a right-angled triangle, where Sin 30° equals 1/2.
• 😀 A point with coordinates (√3, -1) forms a right triangle with a 30° angle, where the sine of 330° is -1/2, based on the same relation as Sin 30° but with a negative value.
• 😀 The concept of relating angles from different quadrants (like Sin 30° and Sin 330°) is crucial in understanding trigonometric identities and their properties.

Q & A

What are the five special angles in quadrant 1 mentioned in the script?

The five special angles in quadrant 1 are the standard angles used in trigonometric functions, which are typically 30°, 45°, 60°, 90°, and 0°.

What is the relationship between the trigonometric ratios in different quadrants?

Trigonometric ratios change sign depending on the quadrant in which the angle is located. In quadrant 1, all functions are positive, in quadrant 2, sine is positive while cosine and tangent are negative, in quadrant 3, tangent is positive while sine and cosine are negative, and in quadrant 4, cosine is positive while sine and tangent are negative.

How is the value of sin 120° determined in the script?

Sin 120° is determined by using the formula sin(180° - 60°). According to the sine identity, sin(180° - θ) = sin(θ). Thus, sin 120° is equal to sin 60°, which is √3/2.

How is the value of cos 210° calculated in the script?

Cos 210° is calculated using the formula cos(180° + 30°). According to the cosine identity, cos(180° + θ) = -cos(θ). Thus, cos 210° equals -cos 30°, which is -√3/2.

How is the value of sin 330° determined in the script?

Sin 330° is determined by using the formula sin(360° - 30°). According to the sine identity, sin(360° - θ) = -sin(θ). Thus, sin 330° is equal to -sin 30°, which is -1/2.

What is the significance of using identities for calculating trigonometric values in different quadrants?

Using identities helps simplify the process of finding trigonometric values in different quadrants by converting angles into familiar reference angles. The identities for sine and cosine in specific quadrants allow for a more systematic and error-free calculation.

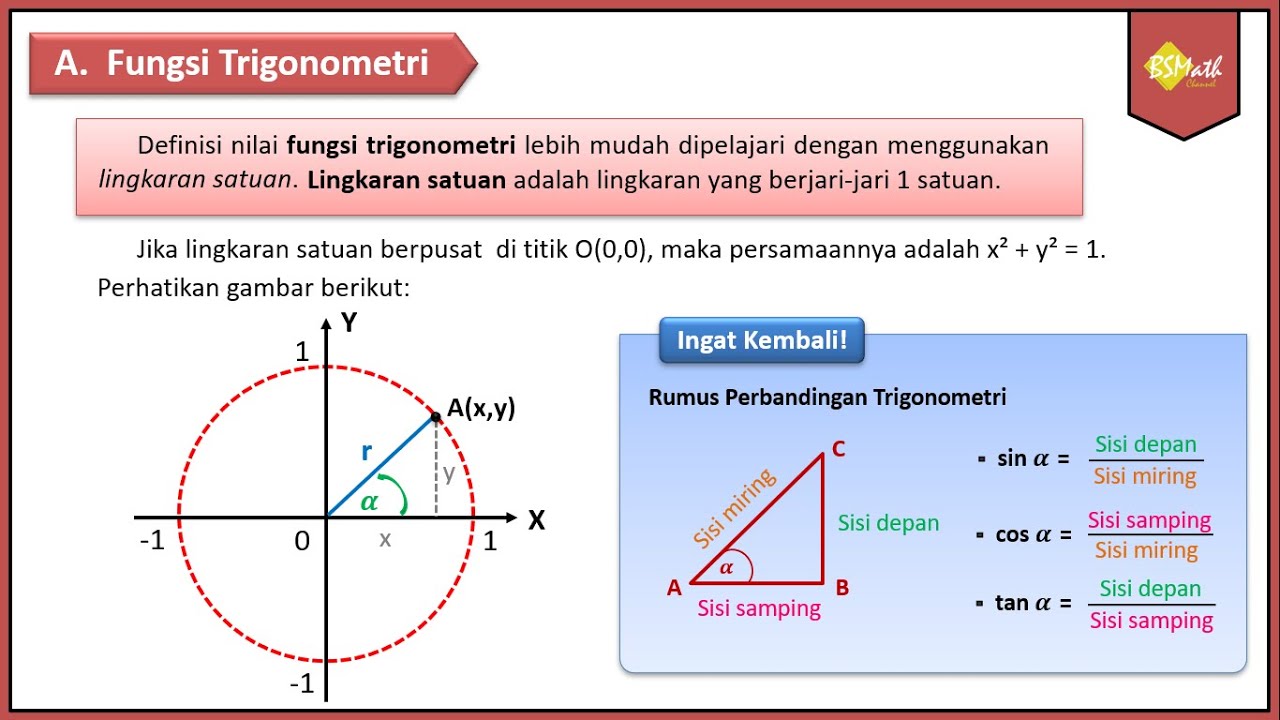
What is the basic definition of sine (sin) in a right triangle, as explained in the script?

In a right triangle, sine (sin) of an angle is defined as the ratio of the length of the side opposite the angle to the length of the hypotenuse.

How does the script use the Pythagorean theorem to calculate the hypotenuse?

The script mentions using the Pythagorean theorem to calculate the hypotenuse, where the hypotenuse is found by applying the formula √(x² + y²), where x and y are the lengths of the other two sides of the triangle.

What is the relationship between sin 30° and sin 330° based on the script?

The script demonstrates that sin 30° and sin 330° are related by having the same numerical value, but with different signs. Sin 30° is positive (1/2), while sin 330° is negative (-1/2).

How does the script confirm the correctness of the trigonometric values for the angles 120°, 210°, and 330°?

The script confirms the correctness of the trigonometric values by referencing the quadrant in which each angle lies and ensuring that the signs of the trigonometric functions are appropriate for that quadrant. For example, sin 120° is positive in quadrant 2, cos 210° is negative in quadrant 3, and sin 330° is negative in quadrant 4.