(TRIK) Perbandingan Trigonometri Sudut Berelasi #fazanugas

Summary

In this video, the host provides an in-depth explanation of trigonometric ratios and their relationships in different quadrants, focusing on special angles and how to memorize their values. The script covers the signs of trigonometric functions in each quadrant, offering practical tips for remembering key values such as sin, cos, and tan. It also introduces the concept of relational angles and demonstrates how to work with angle transformations, giving viewers clear methods to calculate trigonometric values efficiently. The host emphasizes understanding the patterns rather than rote memorization, making the topic more accessible and engaging.

Takeaways

• 😀 Special angles in trigonometry form a pattern: sin(0°) = 0, sin(30°) = 1/2, sin(45°) = √2/2, etc.
• 😀 Understanding the relationship between sin, cos, and tan helps in memorizing trigonometric values for special angles.
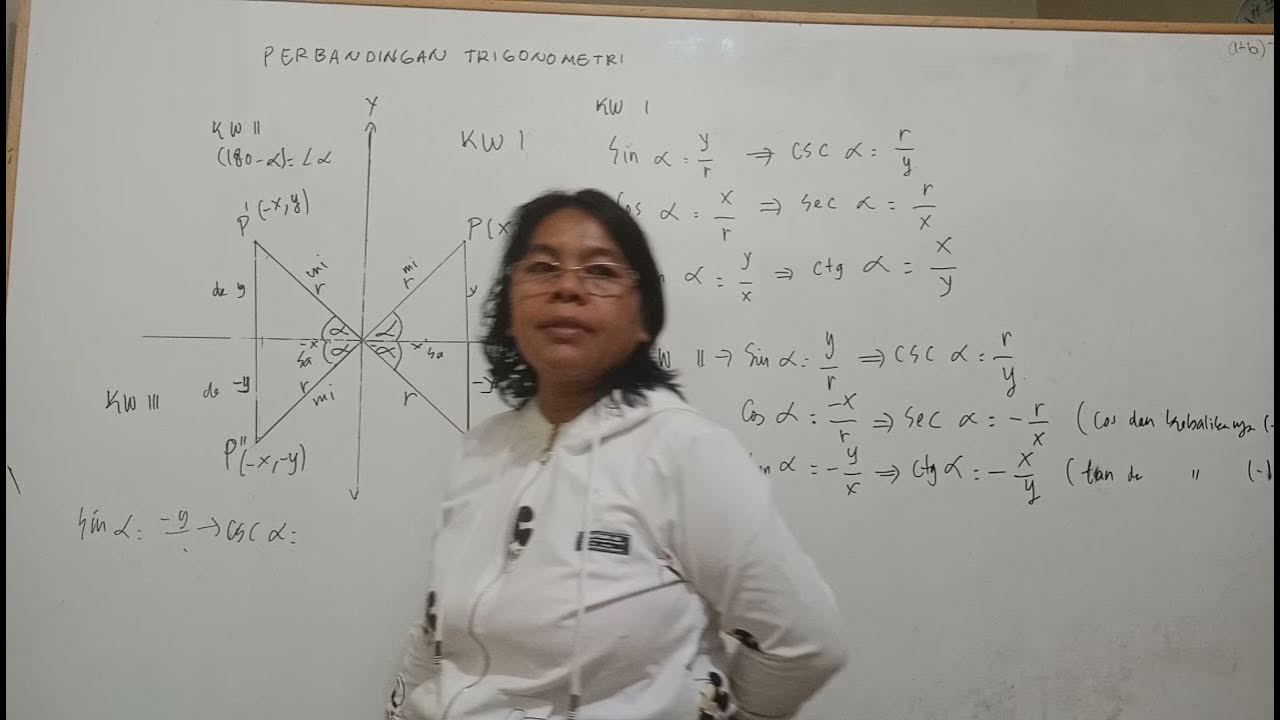
• 😀 The signs of trigonometric functions vary across different quadrants: all positive in Quadrant 1, only sin positive in Quadrant 2, only tan positive in Quadrant 3, and only cos positive in Quadrant 4.
• 😀 A helpful mnemonic for remembering the signs of trigonometric functions in each quadrant is 'All Students Take Calculus' (ASTC).
• 😀 To find trigonometric values for related angles, use the angle sum and difference identities, e.g., sin(120°) = cos(30°).
• 😀 In trigonometric identities, the relation between sin, cos, and tan helps simplify the process of solving problems.
• 😀 Memorizing key values for sin, cos, and tan for specific angles (30°, 45°, 60°, etc.) is crucial for solving trigonometric problems.
• 😀 A key rule in the quadrant system: if the angle is in Quadrant 2, sin is positive, while cos and tan are negative.
• 😀 The tangent function can be calculated as the ratio of sin to cos (tan(θ) = sin(θ) / cos(θ)).
• 😀 Practice with various angle transformations, like 180° - angle or 360° - angle, helps simplify trigonometric evaluations.

Q & A

What is the significance of special angles like 30°, 45°, and 60° in trigonometry?

Special angles like 30°, 45°, and 60° are significant because their trigonometric values (sine, cosine, tangent) follow a specific pattern that makes them easier to memorize and use in calculations. For example, sin 30° = 1/2, cos 30° = √3/2, and tan 45° = 1.

How are the trigonometric values for angles like 30° and 45° derived?

The trigonometric values for angles like 30° and 45° can be derived from geometric principles, particularly using the properties of right-angled triangles, unit circles, or by using known relationships in trigonometry. For instance, sin 30° = 1/2 comes from a 30-60-90 triangle.

What is the role of quadrant signs in determining the value of trigonometric functions?

The signs of trigonometric functions (sine, cosine, tangent) depend on the quadrant in which the angle lies. For example, in Quadrant I, all functions are positive; in Quadrant II, only sine is positive; in Quadrant III, only tangent is positive; and in Quadrant IV, only cosine is positive.

How can the mnemonic 'All Students Take Calculus' help in remembering the signs of trigonometric functions in different quadrants?

The mnemonic 'All Students Take Calculus' helps remember the signs of trigonometric functions in each quadrant. It stands for: 'All' for Quadrant I (all positive), 'Students' for Quadrant II (only sine positive), 'Take' for Quadrant III (only tangent positive), and 'Calculus' for Quadrant IV (only cosine positive).

What does the term 'related angles' or 'sudut berelasi' mean in trigonometry?

Related angles refer to angles that have trigonometric values related to one another. For example, sin(120°) can be expressed in terms of sin(60°), and cos(45°) can be used to find the value of related angles in different quadrants.

What are the two key factors to consider when solving trigonometric problems with related angles?

The two key factors to consider are: 1) The quadrant in which the angle lies (to determine the sign), and 2) The relation being used (whether the angle is a multiple of 90° or 180°, or if it’s an odd/even multiple).

How does changing the sign of trigonometric functions in different quadrants affect calculations?

The sign of trigonometric functions changes based on the quadrant. For example, in Quadrant II, sine is positive while cosine and tangent are negative. These sign changes must be factored in when calculating or simplifying trigonometric expressions.

What are some strategies to memorize the trigonometric values of special angles and their signs?

One strategy is to use a mnemonic for the signs in each quadrant ('All Students Take Calculus'). Additionally, understanding the patterns in special angle values (like sin 30° = 1/2, cos 45° = √2/2) and practicing with unit circles or special triangles can make memorization easier.

What is the formula used to convert sin 120° into a simpler expression?

Sin 120° can be rewritten as sin(90° + 30°). Using trigonometric identities, it can be simplified by recognizing that sin(90° + x) = cos(x). Therefore, sin 120° becomes cos 30°, which equals √3/2.

How can understanding trigonometric identities help simplify complex trigonometric problems?

Understanding trigonometric identities, such as the Pythagorean identities or angle sum/difference formulas, can simplify complex problems by allowing you to convert trigonometric expressions into simpler forms. This makes it easier to compute or compare values across different quadrants.