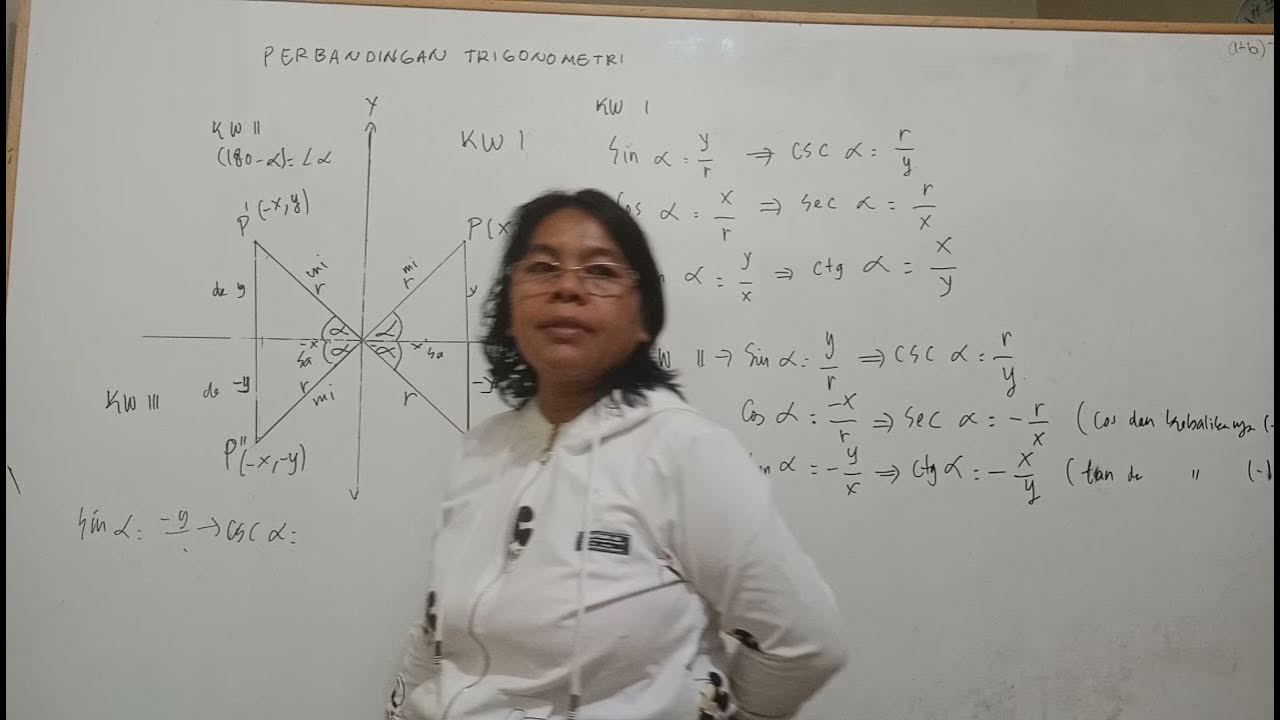
sudut berelasi, sudut di berbagai kuadran

Summary

This educational video from BOM Channel offers a clear and concise explanation of trigonometric relationships in different quadrants. It explores how sine, cosine, and tangent functions behave in the first, second, third, and fourth quadrants, with emphasis on their positivity and negativity in each quadrant. Special angle values and relevant reference angle relationships (like 90°, 180°, 270°, and 360°) are used to demonstrate the process. The video also includes practical exercises to reinforce the learning, helping viewers understand how to calculate trigonometric functions based on the quadrant and angle.

Takeaways

• 😀 Quadrants divide a circle into four parts, each with specific trigonometric function properties.
• 😀 In Quadrant 1 (0° to 90°), sine, cosine, and tangent are all positive.
• 😀 Quadrant 2 (90° to 180°) sees sine as positive, while cosine and tangent are negative.
• 😀 In Quadrant 3 (180° to 270°), tangent is positive, but sine and cosine are negative.
• 😀 Quadrant 4 (270° to 360°) has cosine positive, while sine and tangent are negative.
• 😀 Special angle identities, such as sin(30°) = cos(60°), help in understanding relations across quadrants.
• 😀 Trigonometric function values change depending on the quadrant, using identities like 90° + α, 180° + α, etc.
• 😀 Using angle relations (e.g., 180° + α or 360° + α) can simplify finding values of sin, cos, and tan.
• 😀 The sign of the trigonometric function (positive or negative) is determined by the quadrant of the angle.
• 😀 Practice with different angles helps in mastering the signs and values of trigonometric functions across all quadrants.

Q & A

What is a quadrant in trigonometry?

A quadrant is one of the four sections of a circle, divided into equal parts. Each quadrant corresponds to a specific range of angles, where Quadrant 1 is between 0° and 90°, Quadrant 2 is between 90° and 180°, Quadrant 3 is between 180° and 270°, and Quadrant 4 is between 270° and 360°.

What are the key trigonometric functions covered in this video?

The key trigonometric functions covered are sine (sin), cosine (cos), and tangent (tan), focusing on their values in different quadrants and how they change based on the angle in each quadrant.

How does the value of sin, cos, and tan behave in Quadrant 1?

In Quadrant 1, the values of sin, cos, and tan are all positive. This is because all the trigonometric functions are based on the angle's relationship with the adjacent, opposite, and hypotenuse sides of the right triangle, and all these sides are positive in this quadrant.

What is the relation of trigonometric functions in Quadrant 2?

In Quadrant 2, sin is positive while cos and tan are negative. The trigonometric functions can be related using the formula: sin(90° + α) = cos(α), cos(90° + α) = -sin(α), and tan(90° + α) = -cot(α).

How does the value of sin, cos, and tan behave in Quadrant 3?

In Quadrant 3, sin and cos are negative, while tan is positive. The trigonometric functions in this quadrant can be described using formulas like sin(180° + α) = -sin(α), cos(180° + α) = -cos(α), and tan(180° + α) = tan(α).

What is the rule for trigonometric functions in Quadrant 4?

In Quadrant 4, cos is positive, while sin and tan are negative. Trigonometric functions can be related using sin(360° - α) = -sin(α), cos(360° - α) = cos(α), and tan(360° - α) = -tan(α).

What is the general rule for determining the sign of trigonometric functions in each quadrant?

The signs of the trigonometric functions in each quadrant follow this pattern: Quadrant 1 (All functions positive), Quadrant 2 (Sine positive, others negative), Quadrant 3 (Tangent positive, others negative), and Quadrant 4 (Cosine positive, others negative).

How does using 180° or 360° impact trigonometric functions?

When using 180° or 360°, the trigonometric functions (sin, cos, tan) retain their values, but the sign of the functions may change depending on the quadrant. For example, sin(180° - α) = sin(α), cos(180° - α) = -cos(α), and tan(180° - α) = -tan(α).

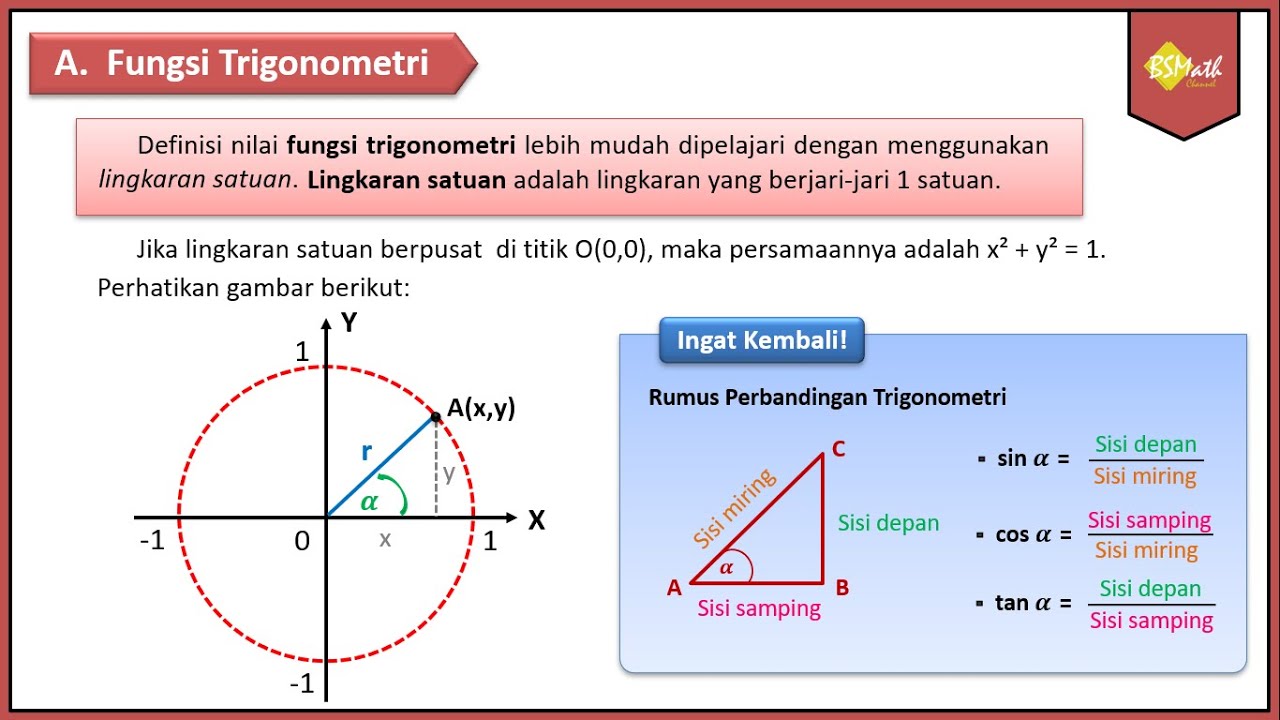
What is the formula for sin, cos, and tan in Quadrant 1 based on a right triangle?

In Quadrant 1, if we consider a right triangle with sides 'x' (adjacent), 'y' (opposite), and 'r' (hypotenuse), then: sin(α) = y/r, cos(α) = x/r, and tan(α) = y/x.

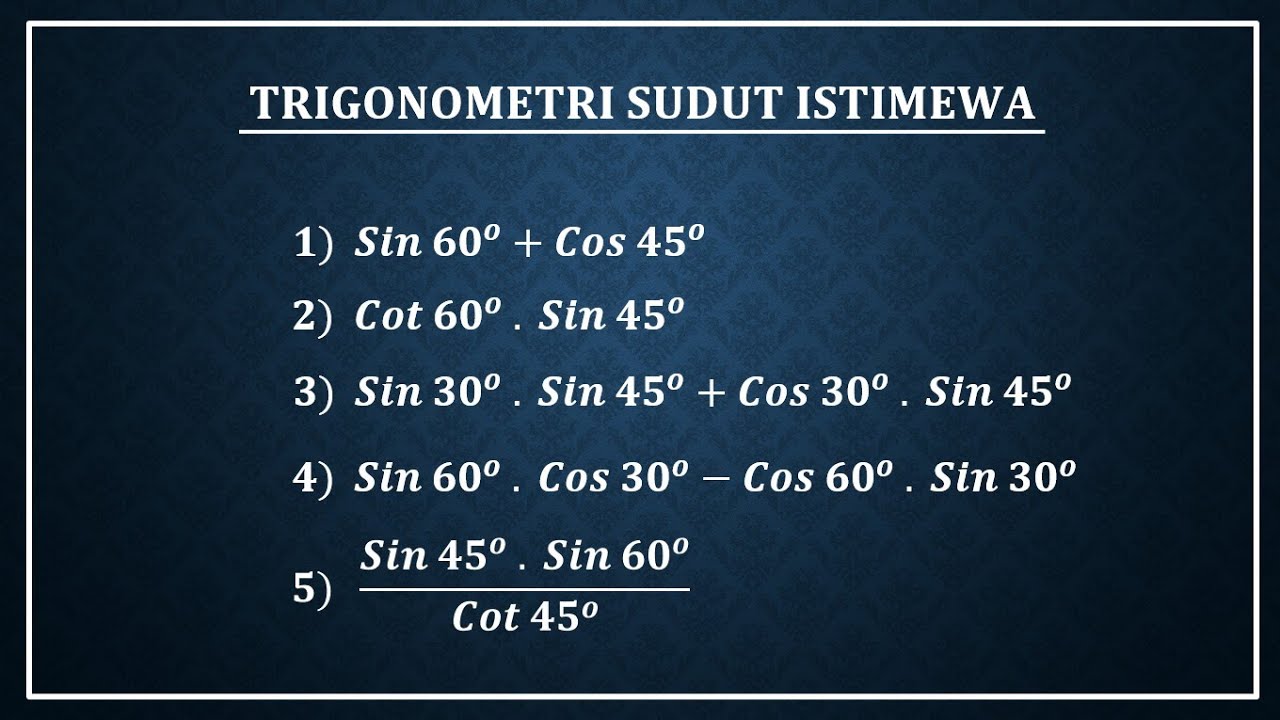
How can you determine the values of sin, cos, and tan for specific angles like 30°, 60°, or 90°?

For specific angles such as 30°, 60°, or 90°, you can use the known values of the sine, cosine, and tangent functions or apply the basic trigonometric ratios to find their values. For example, sin(30°) = 1/2, cos(60°) = 1/2, and tan(45°) = 1.