03. MG3217 Kendali Proses S01: Respons Sistem Orde - 1

Summary

This video discusses the fundamentals of first-order systems in control theory, with a focus on temperature and tank level control. It explains the importance of sensors like thermocouples and infrared thermometers for accurate readings. The lecturer also covers the use of mathematical models, including Laplace transforms, to describe and analyze system responses. Practical applications in automation, system stability, and process control are highlighted, and tools like Excel are mentioned for simulating and solving these systems. The video is an insightful guide to understanding and applying control systems in various industrial contexts.

Takeaways

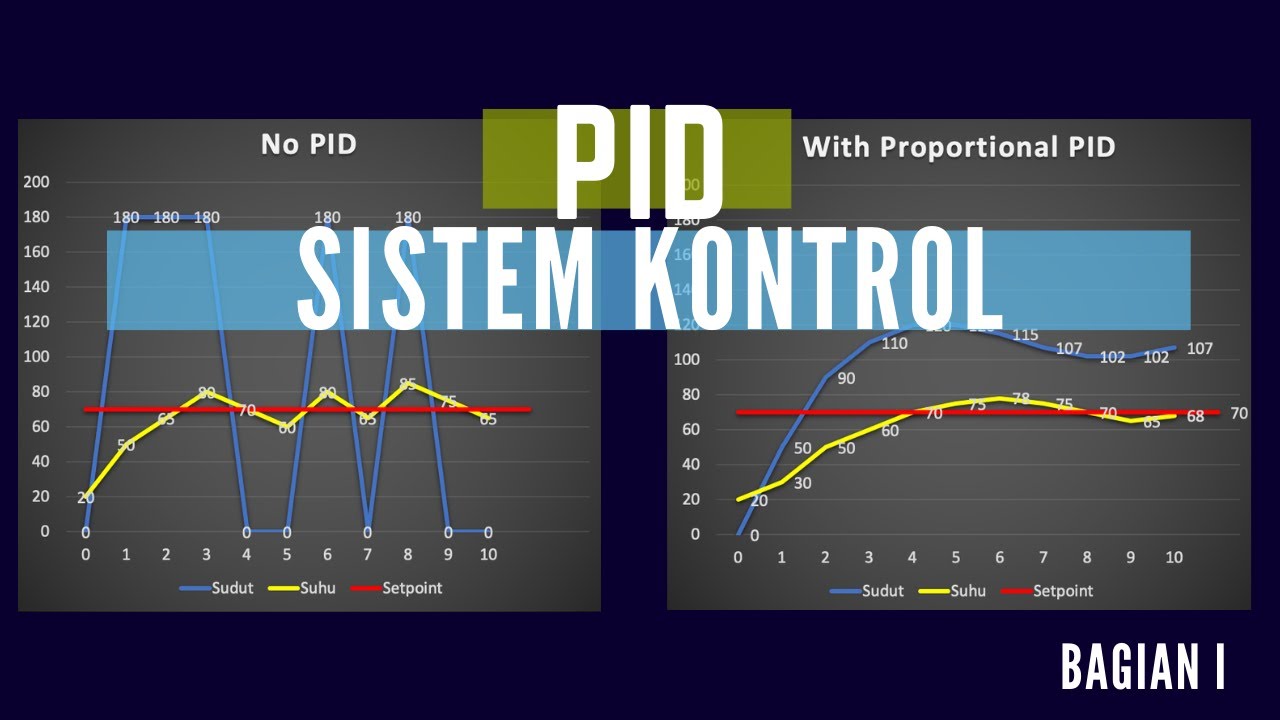
• 😀 First-order system responses play a crucial role in automatic control systems, such as water level control in tanks and temperature regulation.
• 😀 Sensors like thermocouples, resistance thermometers, and infrared thermometers are essential for accurate temperature measurement and system feedback.
• 😀 Water tank level control is a practical example of a first-order system, where the water level is controlled through a feedback loop.
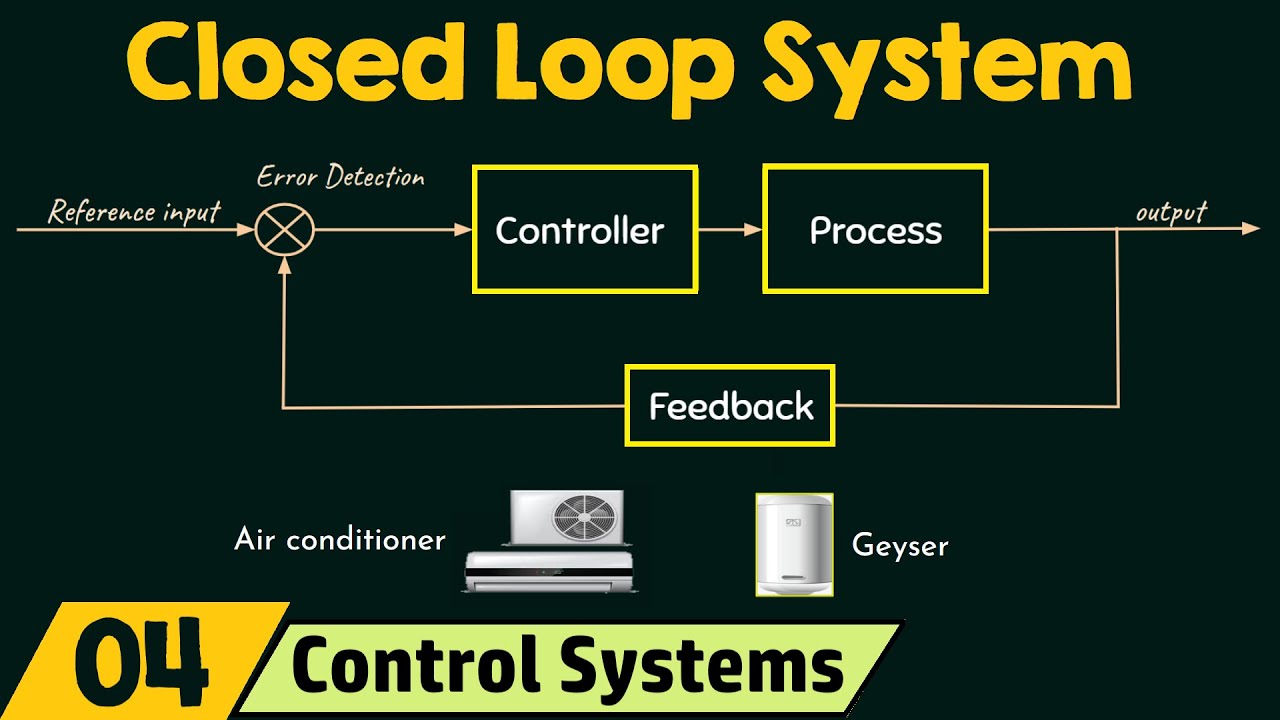
• 😀 Transfer functions represent the dynamic behavior of a system in the frequency domain, allowing for the analysis and design of control systems.
• 😀 The Laplace Transform is used to convert system equations into the frequency domain, simplifying analysis and helping to design controllers.
• 😀 In control engineering, understanding the time and frequency response of systems is essential to ensure stable and accurate performance.
• 😀 The time domain response of a system can be visualized using exponential functions, which are typical in first-order systems.
• 😀 Excel can be used as a tool to simulate and visualize the behavior of control systems, including the responses of first-order systems.
• 😀 The concept of pole-zero analysis helps in determining system stability and behavior in the Laplace domain.
• 😀 Control systems like temperature controllers and water level regulators often require careful tuning of parameters to maintain stability and desired performance.
• 😀 Applying system dynamics and transfer functions in practical applications allows engineers to create efficient and responsive control systems for everyday uses.

Q & A

What is the main topic discussed in the video script?

The main topic of the video script is the concept of Laplace transforms, their reverse process, and how to work with them using Excel for easier calculations and analysis.

What is the significance of understanding the transfer function in this context?

Understanding the transfer function is crucial as it helps in interpreting how a system behaves in the Laplace domain, which is necessary for solving and analyzing differential equations more easily.

What does the speaker mean by 'transforming Laplace to time domain'?

Transforming Laplace to time domain refers to the process of taking a function from the Laplace domain (frequency domain) and converting it back to its original time-domain form, which represents the system's behavior over time.

Why is it important to use Excel for these calculations?

Excel is mentioned as a useful tool for handling the complex calculations involved in Laplace transforms and their inverses, making it easier for users to manage and solve these problems without requiring advanced mathematical software.

What does the speaker hope to achieve with the video content?

The speaker hopes to provide viewers with an understanding of Laplace transforms and how to apply them practically, using tools like Excel to facilitate the process and enhance learning.

What is the importance of the reverse process of Laplace transforms?

The reverse process of Laplace transforms is important because it allows one to convert a function back from the Laplace domain to the time domain, which is necessary for real-world applications like solving differential equations that describe physical systems.

How does the speaker suggest approaching the concept of Laplace transforms?

The speaker suggests understanding the function's behavior in the Laplace domain and then learning how to convert it back into the time domain to solve the problem effectively, making the process more manageable with tools like Excel.

What type of calculations or analysis is the speaker referring to when mentioning Excel?

The speaker refers to the calculations involved in working with Laplace transforms, such as performing inverse transformations and solving equations, which can be simplified and managed with Excel.

What are the benefits of learning about Laplace transforms for practical applications?

Learning about Laplace transforms helps in solving complex engineering and physics problems, particularly those involving systems dynamics, circuit analysis, and control systems, where they simplify the process of solving differential equations.

What tone does the speaker use at the end of the video?

The speaker uses a polite and formal tone, thanking the audience for their attention and wishing them well, conveying a sense of closure and appreciation.