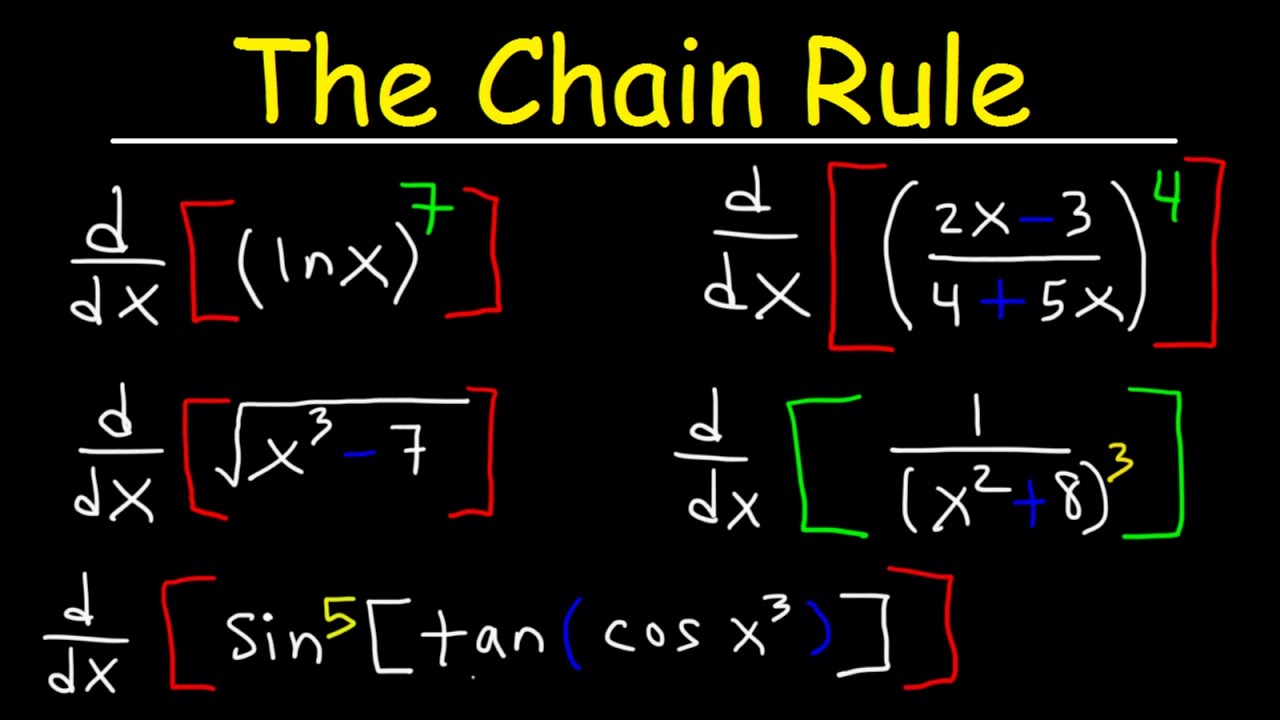
Aturan Rantai (Turunan Fungsi Komposisi)

Summary

In this video, the presenter explains the chain rule for derivatives, focusing on composite functions in calculus. The tutorial covers the chain rule for single-variable, two-variable, and three-variable functions, offering clear graphical illustrations and detailed explanations. Examples are provided to demonstrate the application of the chain rule in various scenarios, including solving for derivatives with respect to multiple variables. The content is tailored to students learning calculus, with practical examples and tips to help understand and apply the chain rule effectively. Viewers are encouraged to try exercises and engage with the material for deeper understanding.

Takeaways

• 😀 The Chain Rule is important for differentiating composite functions, and it is essential for calculus courses.
• 😀 The Chain Rule for a composition of functions, where y = f(x) = t, involves taking the derivative of y with respect to x and then with respect to t.
• 😀 For a function of two variables, dz = f(x, y) with x = x(t) and y = y(t), the derivative of z with respect to t involves applying the chain rule to both x and y.
• 😀 The chain rule for a function of two variables involves calculating the derivatives of z with respect to x and y, then multiplying them by the derivatives of x and y with respect to t.
• 😀 For functions with more than two variables, the chain rule can be applied similarly by considering each variable's derivative with respect to t.
• 😀 In the case of three variables, w = f(x, y, z) with x, y, and z being functions of t, you apply the chain rule for each of the three variables.
• 😀 An example involving z = x^3 y shows how to apply the chain rule, where the derivatives of x and y with respect to t are multiplied accordingly.
• 😀 For the example with z = x^3 y, the derivative dz/dt was computed by differentiating x and y with respect to t, applying the chain rule to get the final expression.
• 😀 The chain rule involves differentiating each term with respect to its respective variable and then combining the results to form the final derivative expression.
• 😀 The video also encourages practice with additional exercises to solidify understanding of the chain rule for composite functions in calculus.

Q & A

What is the main topic of the video?

The main topic of the video is the chain rule for derivatives in calculus, particularly focusing on the chain rule for composite functions with one, two, and three variables.

What is the chain rule in calculus?

The chain rule in calculus is a formula used to compute the derivative of a composite function. It states that the derivative of a composition of functions is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function.

How is the chain rule applied to a single-variable composite function?

For a composite function y = f(x(t)), where both f and x are differentiable, the chain rule states that dy/dt = (dy/dx) * (dx/dt), where dy/dx is the derivative of f with respect to x, and dx/dt is the derivative of x with respect to t.

How does the chain rule apply to functions with two variables?

For a composite function with two variables, such as z = f(x, y), where x and y are functions of t, the derivative dz/dt is given by (dz/dx)*(dx/dt) + (dz/dy)*(dy/dt), where dz/dx and dz/dy are partial derivatives of z with respect to x and y, respectively.

What happens when applying the chain rule to a function with three variables?

For a function with three variables, such as w = f(x, y, z), where x, y, and z are functions of t, the derivative dw/dt is calculated using the chain rule as (dw/dx)*(dx/dt) + (dw/dy)*(dy/dt) + (dw/dz)*(dz/dt), where each term is the partial derivative of w with respect to the respective variable.

Can you provide an example of applying the chain rule with two variables?

Yes, for example, if z = 3x^2 - y^2, x = 2t - 7, and y = 5t, then to find dz/dt, you differentiate z with respect to x and y, then multiply each by dx/dt and dy/dt, respectively, resulting in dz/dt = (6x)*(2) + (-2y)*(5).

What is the difference between the chain rule for a single variable and multiple variables?

The chain rule for a single variable involves a single derivative, whereas for multiple variables, it requires partial derivatives with respect to each variable involved in the function and then applying the chain rule to each derivative.

Why is it important to understand the chain rule in calculus?

Understanding the chain rule is crucial in calculus as it allows for the differentiation of composite functions, which are common in real-world applications like physics, engineering, and economics, where functions depend on other functions.

What is the process for differentiating a function involving multiple variables?

The process involves differentiating the function with respect to each variable separately, then applying the chain rule by multiplying the partial derivatives by the respective rates of change of each variable.

What is the significance of using graphical illustrations in explaining the chain rule?

Graphical illustrations help in visualizing how the chain rule works in the context of composite functions, making it easier to understand the relationships between the variables and their derivatives.