Teorema Pythagoras [Part 4] - Menentukan Perbandingan Sisi Segitiga Siku-siku
Summary
TLDRIn this educational video, Pak Beni teaches viewers how to determine the side ratios of special right-angled triangles. He covers the 45°-45°-90° and 30°-60°-90° triangles, explaining their consistent side ratios: 1:1:√2 and 1:√3:2, respectively. Pak Beni walks through various examples, demonstrating how to apply these ratios in practical problems, such as finding missing sides, perimeters, and areas of geometric shapes. The video encourages viewers to practice by solving problems independently and checking their answers, offering both basic and more complex challenges. This content is aimed at middle school students, reinforcing fundamental geometry skills.
Takeaways
- 😀 The video discusses how to determine the ratio of sides in right-angled triangles with special angles (45°-45°-90° and 30°-60°-90°).
- 😀 The teacher (Pak Beni) emphasizes the importance of understanding basic geometric concepts, like the sum of angles in a triangle being 180°.
- 😀 For a 45°-45°-90° triangle, the ratio of the sides is always 1:1:√2 (for the sides opposite the 45° angle and the hypotenuse).
- 😀 Pak Beni shows that in a 45°-45°-90° triangle, the sides opposite the 45° angles are equal in length.
- 😀 For a 30°-60°-90° triangle, the side ratios are 1:√3:2 (for the sides opposite the 30°, 60°, and 90° angles respectively).
- 😀 The video provides practical examples, such as using given side lengths to calculate missing sides using the ratios.
- 😀 A demonstration was given where the side opposite the 45° angle was compared to a known side (72) using the ratio √2.
- 😀 In another example, the teacher used the known side of √32 to calculate the other side using the ratio 1:√2.
- 😀 The video also covers real-world applications such as calculating the perimeter and area of geometric shapes like squares and rectangles based on right-angled triangles.
- 😀 The teacher encourages students to practice by providing problems for them to solve, and answers are provided in the video description for self-checking.
Q & A
What is the main purpose of this video?
-The main purpose of the video is to teach viewers how to determine the side ratios of right-angled triangles with specific angles, namely 45°-45°-90° and 30°-60°-90°.
How does Pak Beni begin the lesson in this video?
-Pak Beni starts by reviewing basic concepts of triangle angles, emphasizing that the sum of all angles in a triangle is always 180°.
What is the key property of a 45°-45°-90° triangle?
-In a 45°-45°-90° triangle, the two legs are of equal length, and the hypotenuse is √2 times the length of either leg. The side ratio is 1:1:√2.
What is the side ratio of a 30°-60°-90° triangle?
-The side ratio of a 30°-60°-90° triangle is 1:√3:2, where 1 is the length of the leg opposite the 30° angle, √3 is the leg opposite the 60° angle, and 2 is the hypotenuse.
How can you determine the length of the hypotenuse in a 45°-45°-90° triangle if one leg is known?
-If one leg is known in a 45°-45°-90° triangle, multiply the length of the leg by √2 to determine the hypotenuse.
What practical example does Pak Beni use to demonstrate the 45°-45°-90° triangle's properties?
-Pak Beni uses a triangle where both legs are equal, and one leg is given as 72 units. By applying the 1:1:√2 ratio, the hypotenuse is calculated as 72√2 units.
What happens when the side lengths of a 30°-60°-90° triangle are known?
-If the side lengths of a 30°-60°-90° triangle are known, you can use the side ratio 1:√3:2 to find the missing side lengths, as demonstrated when one leg is given as 5 units.
How does Pak Beni use real-world examples to apply the concepts?
-Pak Beni demonstrates applying the side ratios to find the perimeter of a square and the area of a rectangle, using the triangle's side ratios to solve for unknown sides.
How does the video encourage student participation?
-The video encourages student participation by offering practice problems and inviting viewers to post their answers in the comment section for feedback.
What will be covered in the next video?
-The next video will cover solving problems related to the Pythagorean Theorem, continuing the discussion from this video.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

SEGITIGA ISTIMEWA SUDUT 30,60,90 DAN SUDUT 45,45,90

Teorema Pythagoras [Part 1] - Menentukan Panjang Salah Satu Sisi Pada Segitiga Siku-siku

Video Pembelajaran Matematika Materi Trigonometri Kelas 10 - Nilai Trigonometri pada Sudut Istimewa

Matematika Kelas 9 | Kesebangunan pada Segitiga Siku-siku

KESEBANGUNAN PADA SEGITIGA SIKU-SIKU
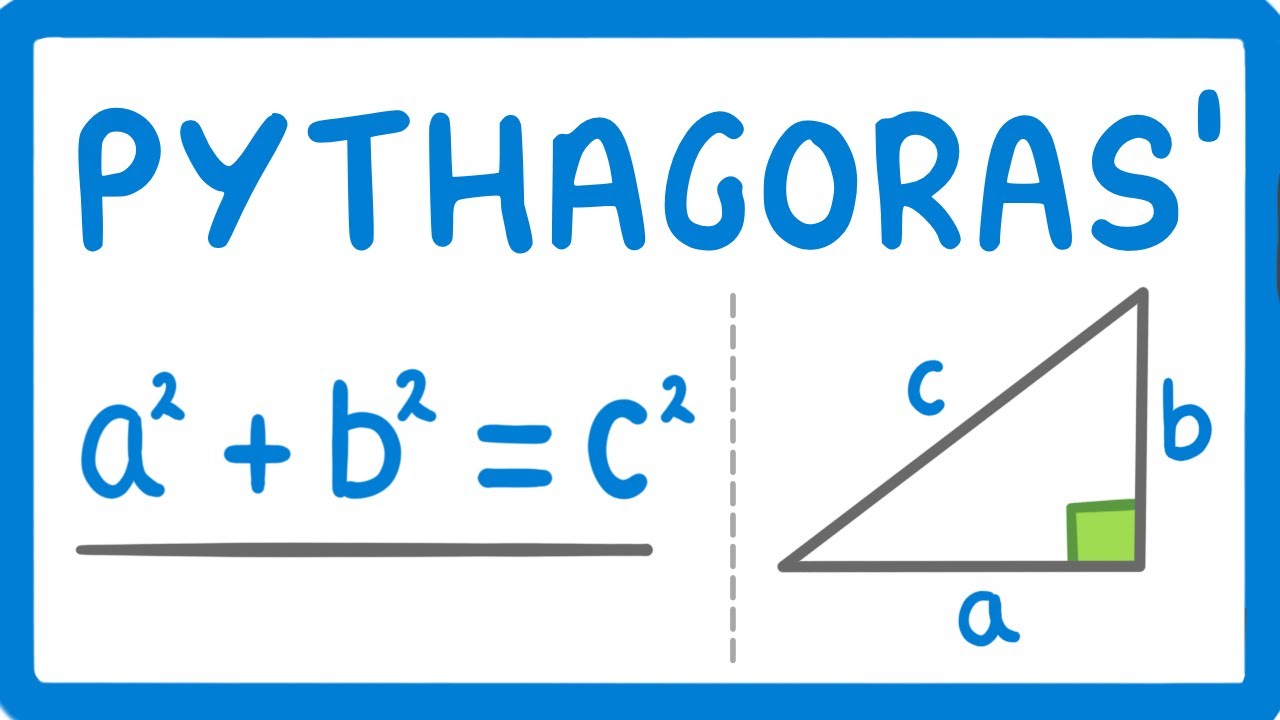
GCSE Maths - Pythagoras' Theorem And How To Use It #120
5.0 / 5 (0 votes)