Matematika Kelas 9 | Kesebangunan pada Segitiga Siku-siku
Summary
TLDRIn this educational video, the presenter explains how to determine the side lengths of right-angled triangles using the Pythagorean theorem and properties of similar triangles. The problem involves triangle ABC, where viewers learn to find the lengths of BD, AB, and BC by applying mathematical principles such as proportionality and Pythagoras' theorem. The video walks through step-by-step solutions for each side, demonstrating the process clearly. Viewers are encouraged to subscribe and share the content, with the goal of making learning more accessible and interactive.
Takeaways
- 😀 The video is about learning similarity in right-angled triangles.
- 😀 The lesson focuses on triangle ABC, which is a right triangle with a right angle at B.
- 😀 The first question is to find the length of side BD in triangle ABC.
- 😀 The second question is to find the length of side AB.
- 😀 The third question is to find the length of side BC.
- 😀 The script explains the process of comparing triangles to find corresponding sides.
- 😀 The length of CD is given as 18 cm, and the length of DE is 32 cm.
- 😀 The sum of CD and DE is calculated as 50 cm.
- 😀 To find BD, the script uses the similarity of the triangles and solves using cross-multiplication, resulting in BD = 24 cm.
- 😀 The length of AB is found using the Pythagorean theorem, resulting in AB = 40 cm.
- 😀 The length of BC is also determined using the Pythagorean theorem, with BC calculated as 30 cm.
Q & A
What is the main topic of the video?
-The main topic of the video is learning about the similarity of right-angled triangles.
What is the specific triangle discussed in the video?
-The specific triangle discussed is triangle ABC, which is a right-angled triangle with the right angle at vertex B.
How many triangles are discussed in the video?
-Three triangles are discussed: triangle ABC, triangle ABD, and triangle BDC.
What method is used to determine the side lengths of the triangles?
-The method used involves triangle similarity and proportionality, along with the Pythagorean theorem.
How is the length of BD calculated?
-The length of BD is calculated by using the proportionality between the similar triangles, resulting in BD = 24 cm.
What formula is used to calculate the length of AB?
-The length of AB is calculated using the Pythagorean theorem: AB = √(AD² + BD²), which results in AB = 40 cm.
How is the length of BC determined?
-The length of BC is determined using the Pythagorean theorem: BC = √(AC² - AB²), which results in BC = 30 cm.
What is the length of segment CD?
-The length of segment CD is 18 cm, as mentioned in the video.
Why is the Pythagorean theorem used in this video?
-The Pythagorean theorem is used to calculate the lengths of the sides of the triangles, especially for the sides AB and BC, which are part of right-angled triangles.
What is the purpose of comparing the triangles in the video?
-The purpose of comparing the triangles is to demonstrate their similarity and use proportionality to calculate the unknown side lengths.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

segitiga-segitiga yang kongruen 2

SEGITIGA ISTIMEWA SUDUT 30,60,90 DAN SUDUT 45,45,90

tripel pythagoras kelas 8 part 2 (mudah) dan menentukan jenis segitiga - Abi Muis Math
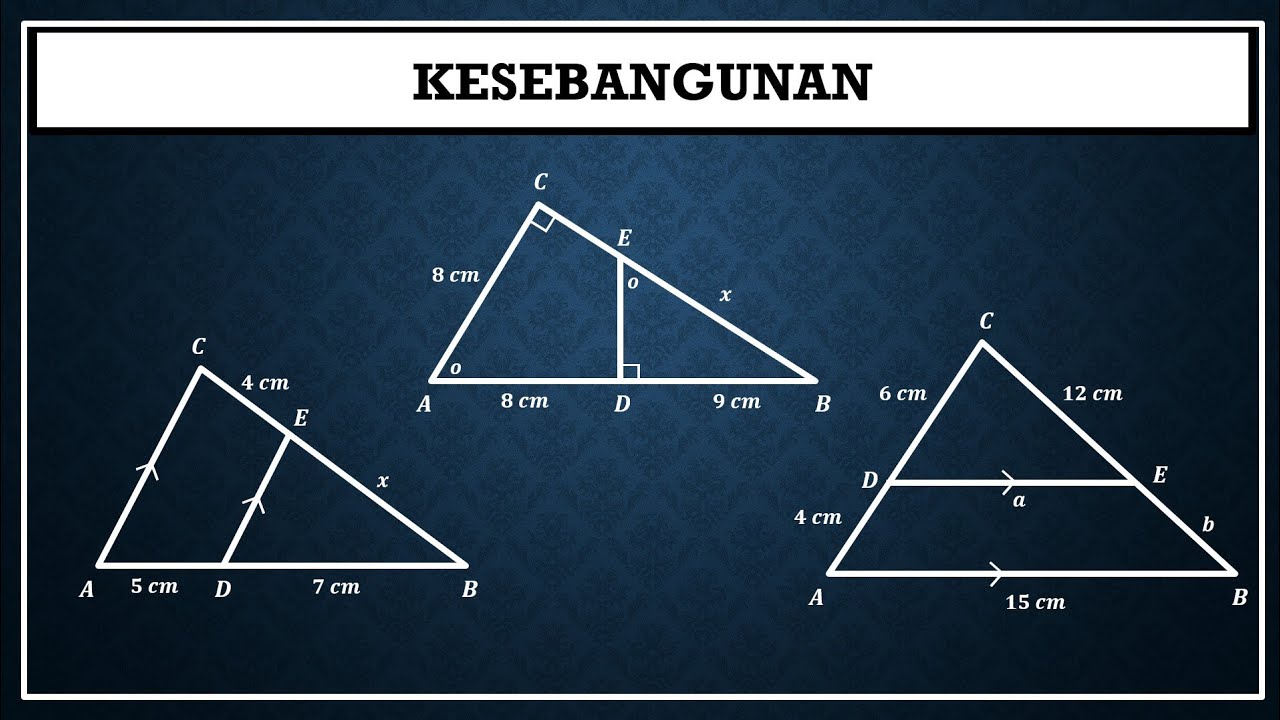
Kesebangunan pada segitiga
Triunghiul dreptunghic, teorema 30 60 90, teorema medianei, teorema lui Pitagora si reciproca, arie

Contoh Soal Perbandingan Trigonometri pada segitiga siku siku
5.0 / 5 (0 votes)