Integral Lipat | Integral Lipat (Part 1) | Kalkulus

Summary

In this video, the host introduces the concept of double integrals (integral lipat), building upon single-variable integrals. The explanation focuses on calculating volumes under surfaces defined by functions of multiple variables, using partitioned regions and summing small volumes. Key properties of multiple integrals, including linearity, additivity, and comparison, are discussed. The video emphasizes that double integrals are useful for solving real-world problems involving areas and volumes, as long as the function is bounded and well-defined. Overall, it's a beginner-friendly explanation of the foundational concepts of integral calculus.

Takeaways

• 😀 Integral calculus involves the concept of calculating areas under curves and volumes under surfaces.
• 😀 A single-variable integral calculates the area under a curve on a 2D plane, achieved by partitioning the area into small rectangles and summing their areas.
• 😀 Multiple integrals extend the concept of integration to functions with two or more variables (e.g., x, y, z), allowing for volume calculations in higher dimensions.
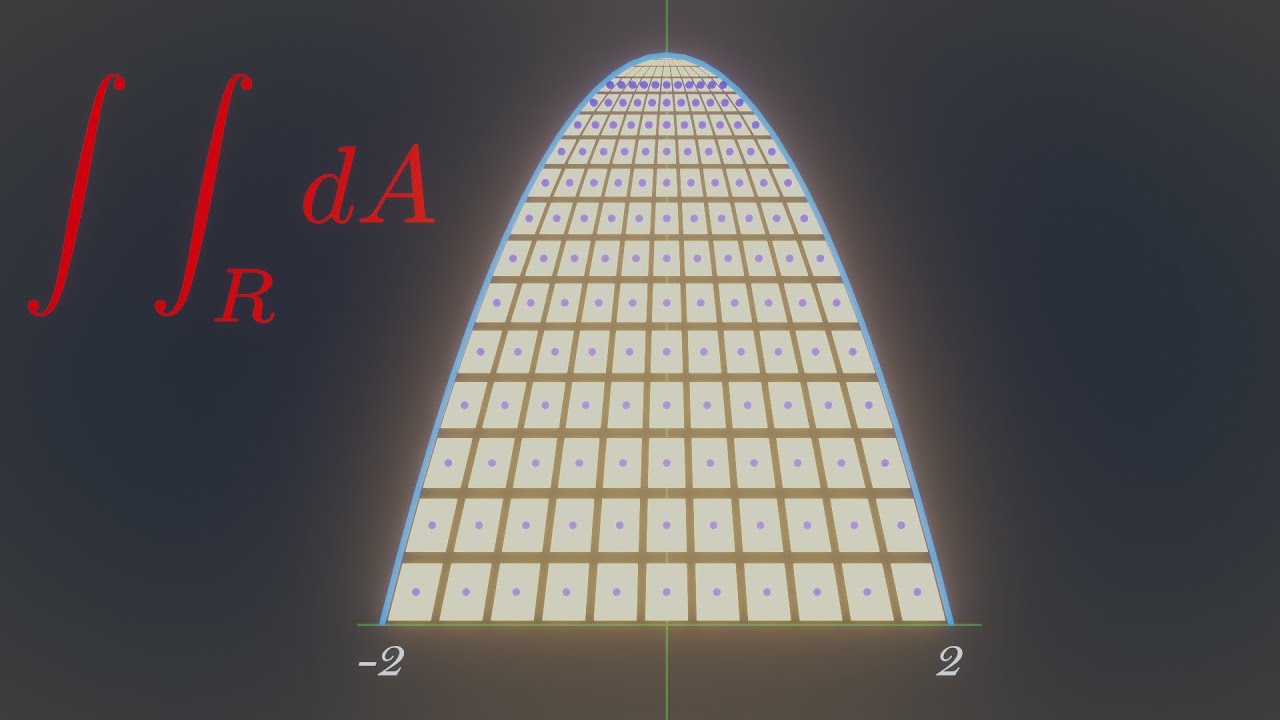
• 😀 The region of integration is partitioned into smaller areas or volumes, and the function's value at each partition is multiplied by the partition's area or volume.
• 😀 The integral represents the volume under the curve defined by the function over the specified region.
• 😀 Multiple integrals can be evaluated by summing the areas or volumes of smaller partitions, which is akin to approximating the total volume using small prisms.
• 😀 For multiple integrals to be well-defined, the function being integrated must be bounded over the region, meaning it does not approach infinity.
• 😀 Key properties of multiple integrals include linearity: integrals of sums can be split, and constants can be factored out.
• 😀 If a region can be divided into subregions, the total integral can be split into the sum of integrals over each subregion.
• 😀 If one function is smaller than another over a region, the integral of the smaller function will be less than or equal to the integral of the larger function over the same region.

Q & A

What is the basic concept of a multivariable integral?

A multivariable integral extends the concept of integration to functions of more than one variable. In the script, the example starts with the concept of a double integral, where the function is defined over a two-dimensional region and involves summing the products of the function values and the area of small regions (e.g., rectangles or boxes).

How is a single-variable integral different from a multivariable integral?

A single-variable integral calculates the area under a curve defined by a function of one variable. In contrast, a multivariable integral involves summing the contributions of a function over a region defined by multiple variables (e.g., two or three dimensions), resulting in a volume or area under a surface or across multiple dimensions.

What does a double integral represent in the context of the script?

A double integral, as discussed in the script, represents the volume under a surface defined by a function of two variables. The integration is performed over a two-dimensional region, where the function is evaluated at small partitions (e.g., rectangles) and then summed to approximate the total volume.

What is the significance of the partitions in the multivariable integral?

The partitions divide the region of integration into smaller, manageable sections (e.g., small rectangles or boxes). By evaluating the function at each partition and summing the results, we approximate the total volume or area under the surface described by the function. The smaller the partitions, the more accurate the result.

What is the meaning of 'bounded' in relation to multivariable integrals?

In the context of multivariable integrals, 'bounded' means that the function does not approach infinity within the region of integration. For the integral to exist and be finite, the function must have finite values across the entire region. If the function becomes infinite, the integral is undefined.

Can you describe the linear properties of multivariable integrals?

The linear properties of multivariable integrals allow operations like scaling and addition to be simplified. For instance, if you multiply the integrand by a constant, you can factor it out of the integral. Similarly, the integral of a sum of two functions can be split into the sum of the integrals of the individual functions.

How does the script explain the relationship between integrals over separate regions?

The script explains that if the integration region can be split into two non-overlapping subregions (e.g., R1 and R2), the total integral over the entire region is equal to the sum of the integrals over each subregion. This property is due to the additive nature of integration.

What happens when one function is less than another in the context of multivariable integrals?

When one function, say f(x, y), is less than another function g(x, y), the integral of f over a given region will be less than the integral of g over the same region. This is because the volume under the surface of f will be smaller than the volume under the surface of g, which is higher.

Why are small partitions important in evaluating a multivariable integral?

Small partitions lead to a more accurate approximation of the integral. As the size of the partitions decreases, the approximation of the volume or area under the surface becomes more precise, ultimately converging to the exact value of the integral.

What does the concept of 'volume under a surface' mean in the context of multivariable integrals?

The 'volume under a surface' refers to the total volume enclosed between the surface defined by the function and the plane beneath it, within the specified region. For a double integral, this volume is computed by summing the contributions from each small partition of the region, as described in the script.