Volumen por Método de Cascarones Cilíndricos (alrededor del eje Y) | Ejemplo 2

Summary

In this video, the host demonstrates how to calculate the volume of a region formed by rotating a graph around the y-axis using the cylindrical shells method. The explanation emphasizes the advantages of this method, such as avoiding the need to solve for x, which can be complex. The script covers graphing the region, setting up the integral, and simplifying the calculations. The video also invites viewers to try solving a similar problem and provides additional resources for those interested in further exploration. Overall, it’s a helpful tutorial for understanding volume calculations in calculus.

Takeaways

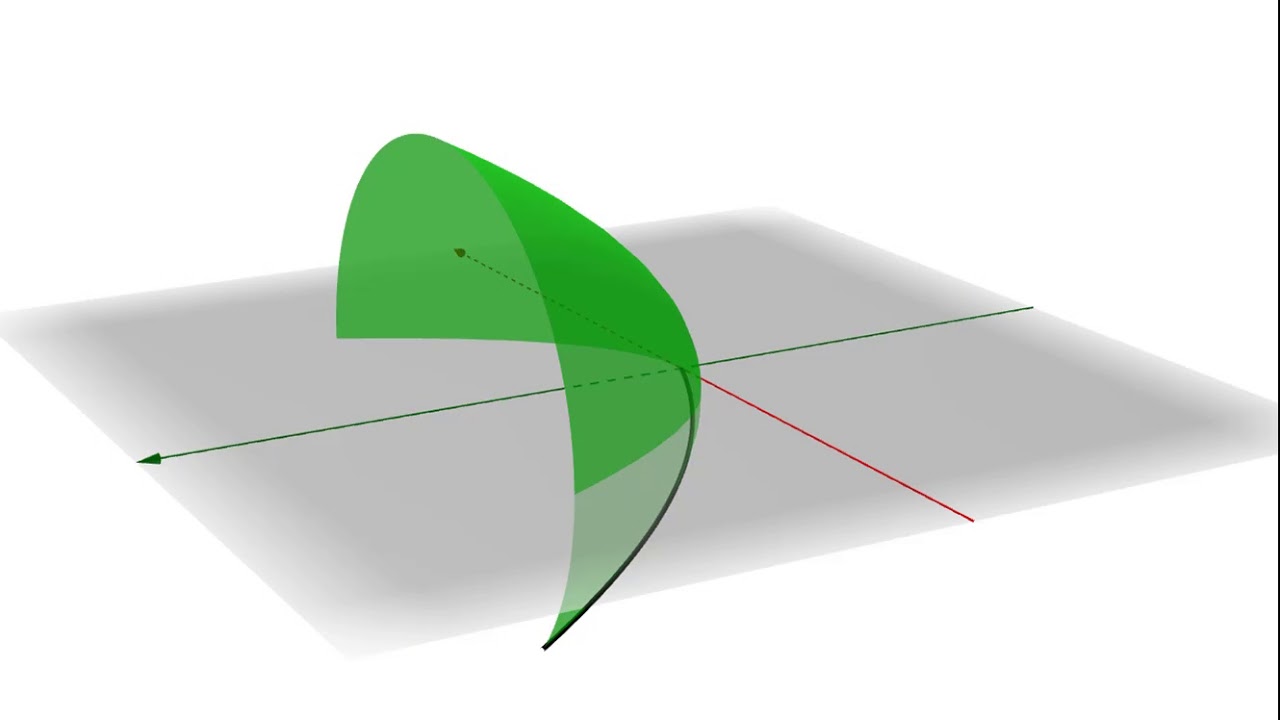
• 😀 The video focuses on calculating the volume obtained by rotating a region around the y-axis using the cylindrical shells method.
• 😀 The cylindrical shells method is preferred in this case because it does not require solving for x, simplifying the process.
• 😀 If the disk method were used, it would require solving for x and dealing with complex logarithmic integrals, making it more difficult.
• 😀 The region being rotated is defined by the equation y = -x^2 and the line x = 1, with x ranging from 0 to 1.
• 😀 The volume is calculated using the formula for cylindrical shells: ∫ 2πx * (function of x) dx, where the function is y = -x^2.
• 😀 The integral for the volume is evaluated from x = 0 to x = 1, where the limits are determined by the region's boundaries.
• 😀 The integral involves simplifying the function by factoring out constant terms and performing standard derivative calculations.
• 😀 After evaluating the integral, the volume is found to be π, a result that is quickly obtained with the cylindrical shells method.
• 😀 The method of cylindrical shells is more efficient than the disk method, which would involve splitting the volume into two regions and adding them.
• 😀 The video encourages viewers to try solving a similar problem on their own using the cylindrical shells method, then verifying the result with another method for comparison.
• 😀 The video concludes by thanking members who support the channel on YouTube and Patreon, and inviting viewers to access exclusive content.

The video is abnormal, and we are working hard to fix it.
Please replace the link and try again.