CILINDRO DE REVOLUCIÓN
Summary
TLDRThis video tutorial explains the concept of a cylinder of revolution, formed by rotating a rectangle around an axis. Key concepts such as the cylinder’s elements, including its bases, axis, height, radius, and generatrix, are thoroughly covered. The tutorial demonstrates how to calculate the lateral surface area, total surface area, and volume of the cylinder with mathematical formulas. It also includes a detailed example, applying the theory to a real-life problem involving a rectangle with a given length and diagonal, helping viewers understand the calculations behind a cylinder's properties.
Takeaways
- 😀 A cylinder of revolution is formed by rotating a rectangular region around an axis that contains one of its sides.
- 😀 The side of the rectangle that moves during the rotation is called the generatrix, and it forms the cylindrical surface.
- 😀 The top and bottom bases of the cylinder are circles, and their radii are equal because the bases are congruent and parallel.
- 😀 The axis of the cylinder is represented by the line along which the rectangle rotates.
- 😀 The height of the cylinder is the distance between the top and bottom circular bases.
- 😀 The lateral surface area of the cylinder can be represented by a rectangle when unfolded, where the base of the rectangle corresponds to the circumference of the cylinder's base.
- 😀 The formula for calculating the lateral surface area of the cylinder is 2πr × h, where 'r' is the radius of the base and 'h' is the height.
- 😀 To calculate the total surface area of a cylinder, we add the lateral area and the areas of the two circular bases: 2πr × h + 2πr².
- 😀 The formula for the volume of a cylinder is πr² × h, where 'r' is the radius of the base and 'h' is the height.
- 😀 An example problem involves a cylinder formed from a rectangle with a length of 8 meters and a diagonal of 10 meters. The lateral area, total area, and volume are calculated using the formulas provided.
- 😀 In the example, the radius of the base is calculated using the Pythagorean theorem, and then the area and volume are computed by substituting the values into the respective formulas.
Q & A
What is a cylinder of revolution?
-A cylinder of revolution is a solid formed by rotating a rectangular region around an axis that contains one of its sides. The rotation generates the cylindrical shape.
What are the key elements of a cylinder of revolution?
-The key elements of a cylinder of revolution are the upper and lower circular bases (which are congruent and parallel), the axis of the cylinder, the height (the distance between the bases), the radius (the distance from the center to the circumference), and the generatrix (the side of the rectangle that forms the surface).
What is the generatrix of a cylinder of revolution?
-The generatrix of a cylinder of revolution is the side of the rectangle that, when rotated around the axis, forms the cylindrical surface.
How do you calculate the lateral surface area of a cylinder of revolution?
-The lateral surface area of a cylinder of revolution is calculated by multiplying the perimeter of the base (2πr) by the height (H). The formula is: Area = 2πrH.
What formula is used to calculate the total surface area of a cylinder of revolution?
-The total surface area of a cylinder of revolution is the sum of the lateral surface area and the areas of the two circular bases. The formula is: Total Area = 2πrH + 2πr², which can be factored as: Total Area = 2πr(H + r).
What is the formula for calculating the volume of a cylinder of revolution?
-The volume of a cylinder of revolution is calculated by multiplying the area of the base (πr²) by the height (H). The formula is: Volume = πr²H.
How do you find the height and radius of a cylinder formed from a rectangle with a given diagonal?
-To find the height and radius, you can use the Pythagorean theorem if the diagonal and one side of the rectangle are known. The missing side will be the radius, and the known side can be the height.
In the example problem, how did the Pythagorean theorem help calculate the missing side of the rectangle?
-In the example problem, the Pythagorean theorem was used to find the missing side of the rectangle. Given that the diagonal was 10 meters and one side was 8 meters, the missing side was calculated as the square root of (10² - 8²), resulting in 6 meters.
What are the correct units for the area and volume of a cylinder?
-The units for the lateral area and total area of a cylinder are square units (e.g., square meters), while the units for the volume are cubic units (e.g., cubic meters).
How does the unwrapping of the cylinder visually help explain the lateral surface area?
-When the cylinder is unwrapped, the lateral surface becomes a rectangle. This visualization helps understand that the lateral surface is a rectangle whose width is the perimeter of the base (2πr) and whose height is the same as the height of the cylinder (H).
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Menentukan Volume Benda Putar dengan Menggunakan Integral (Integral Part 5) M4THLAB
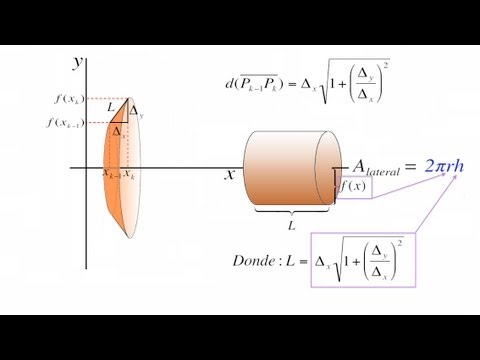
Superficie de Revolución (introducción) - Deducción de la Formula

CILINDRO (AULA 11/16)

Volumen por Método de Cascarones Cilíndricos (alrededor del eje Y) | Ejemplo 2
Volumes of Revolution (Disk Method)
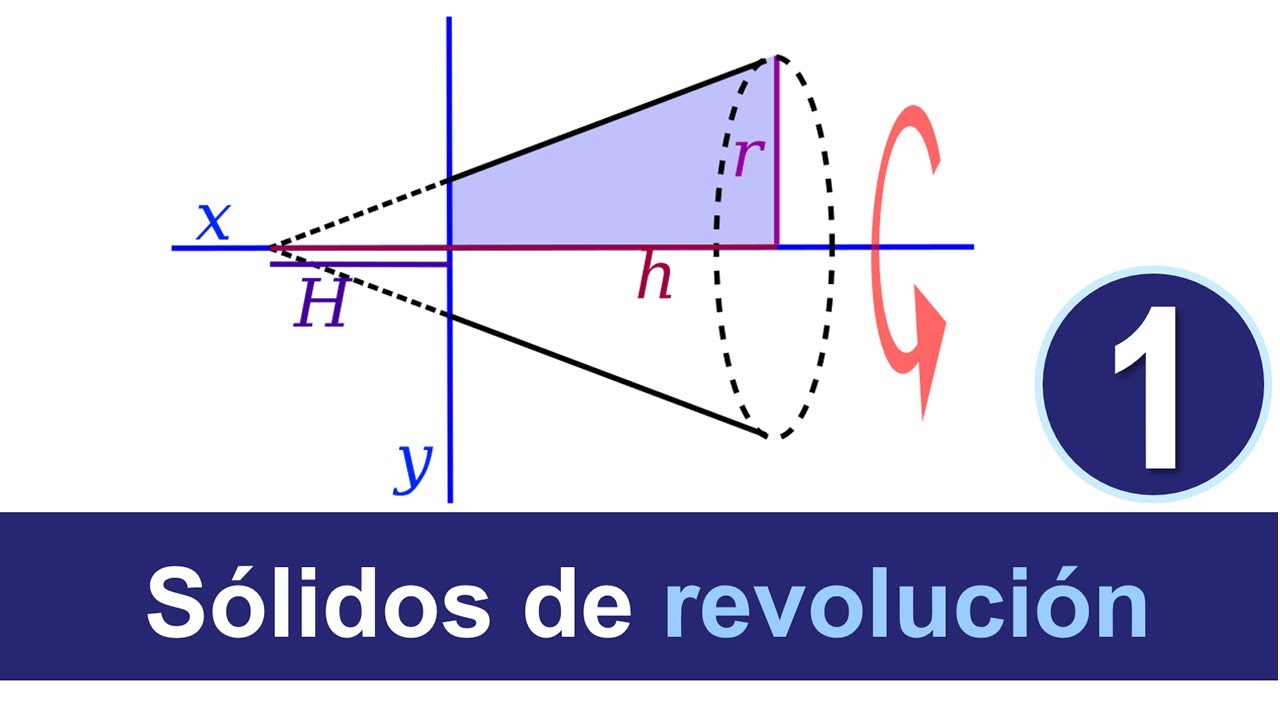
Cálculo Integral - Volumen de sólidos de revolución #1
5.0 / 5 (0 votes)