FOURIER SERIES LECTURE 1 | STUDY OF DEFINITION AND ALL BASIC POINTS @TIKLESACADEMY
Summary
TLDRThis video introduces the concept of Fourier Series, an essential tool for solving periodic functions in mathematics and engineering. The Fourier Series is an infinite sum expressed in terms of trigonometric sine and cosine functions. The video covers its definition, basic properties, and applications, such as solving ordinary and partial differential equations. It emphasizes the importance of mastering this method, particularly for problems involving periodic conditions and multiple independent variables. The next lessons will delve deeper into periodic functions and standard Fourier formulas, guiding viewers toward practical problem-solving skills in engineering mathematics.
Takeaways
- 😀 Fourier Series is an infinite series used to express periodic functions in terms of sine and cosine functions.
- 😀 It is a powerful tool to solve ordinary and partial differential equations, especially when periodic functions are involved.
- 😀 Fourier Series is constructed over one period and is only valid within that period for the corresponding values.
- 😀 The method of Fourier Series allows the decomposition of a periodic function into sines and cosines, facilitating complex problem-solving.
- 😀 Understanding the periodic nature of Fourier Series is essential, as it directly impacts its construction and application.
- 😀 Fourier Series plays a key role in solving modern engineering problems involving multiple independent variables.
- 😀 By using Fourier Series, complex partial differential equations can be simplified and solved more efficiently.
- 😀 Most problems in engineering mathematics today involve solving linear non-homogeneous partial differential equations using Fourier Series.
- 😀 Fourier Series is especially useful for handling boundary value problems in mathematical physics and engineering.
- 😀 The next lessons will focus on periodic functions and their standard formulas in relation to Fourier Series, with more problem-solving techniques.
Q & A
What is the definition of Fourier Series?
-Fourier Series is an infinite series used to represent periodic functions as a sum of sine and cosine terms. It is an expansion of a periodic function in terms of trigonometric functions, which allows us to express complex periodic behaviors in a simplified mathematical form.
Why is Fourier Series considered a powerful method?
-Fourier Series is considered powerful because it provides a method to solve ordinary and partial differential equations, especially those involving periodic functions. It helps to break down complex problems into simpler trigonometric components, making them easier to analyze and solve.
What are the main components involved in studying Fourier Series?
-The main components involved in studying Fourier Series include understanding periodic functions, even and odd functions, changes of interval, and half-range expansions (Fourier Cosine Series and Fourier Sine Series). These topics are essential for building a solid foundation in Fourier analysis.
How does the periodic nature of a function affect the construction of Fourier Series?
-The periodic nature of a function ensures that the Fourier Series is constructed for one period of the function. This makes the series valid for all values within that period, which is crucial for analyzing periodic behaviors in various systems.
What types of differential equations can Fourier Series be used to solve?
-Fourier Series can be used to solve ordinary and partial differential equations, especially those that involve periodic functions. It is particularly useful for solving non-homogeneous equations that appear in many engineering applications, such as heat transfer and wave equations.
What is the significance of the Half-Range Fourier Series?
-The Half-Range Fourier Series is a special case of Fourier Series used when the function is defined only over a half interval. This method allows us to represent a function with only half of the Fourier terms, simplifying the calculation process for certain problems.
How does Fourier Series help in solving real-world engineering problems?
-Fourier Series plays a crucial role in engineering by enabling the solution of problems involving periodic phenomena. It is particularly useful in solving partial differential equations (PDEs) with periodic boundary conditions, such as those found in heat conduction, vibration analysis, and signal processing.
What is the importance of practicing Fourier Series problems?
-Practicing Fourier Series problems is vital because it helps in understanding the application of the theory to real-life situations. The more problems you solve, the better you become at applying Fourier Series to complex differential equations and periodic systems, which is crucial in engineering mathematics.
What types of equations do students typically encounter when solving problems involving Fourier Series?
-Students typically encounter first and second-order linear non-homogeneous partial differential equations when solving problems involving Fourier Series. These equations arise from the presence of multiple independent variables in real-world engineering problems.
Why is it important to understand the periodic function in the context of Fourier Series?
-Understanding the periodic function is critical because Fourier Series is built on the assumption that the function is periodic. This understanding helps in applying the series correctly and ensures that the function is expressed accurately in terms of its trigonometric components for solving differential equations.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

TuMT 221 Tutorial Matematika Teknik - Deret Fourier Trigonometri
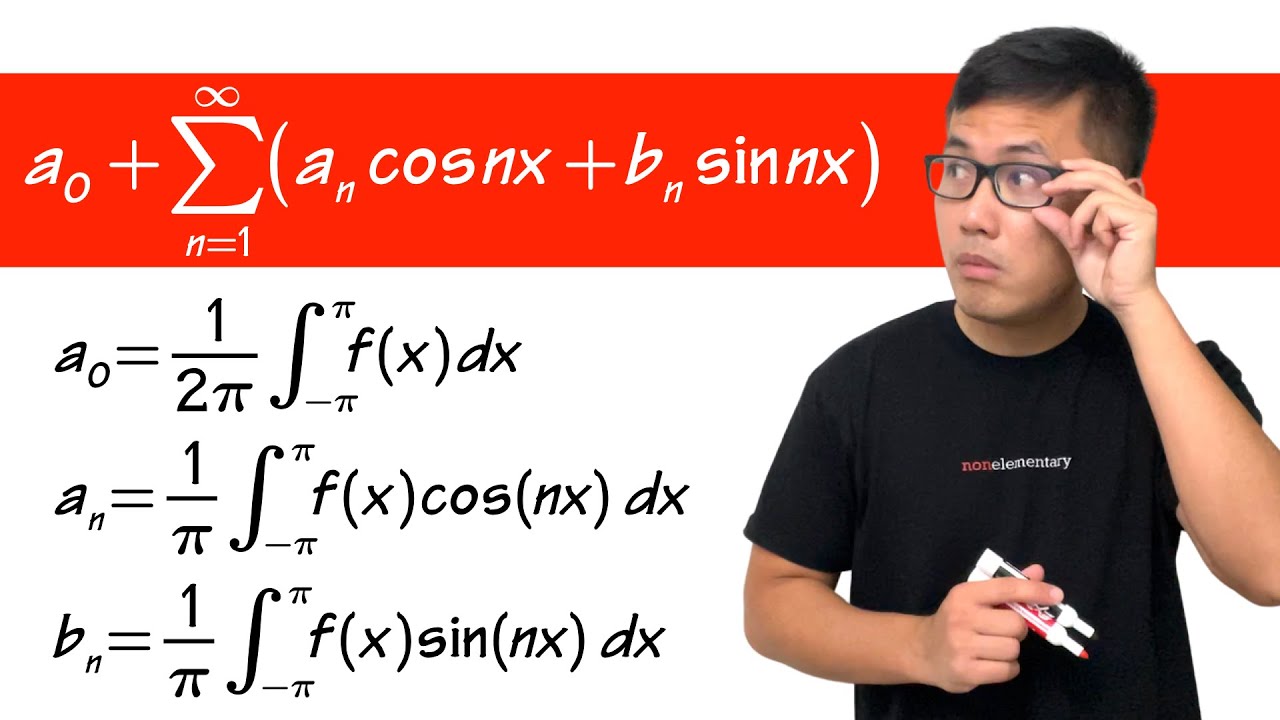
how to get the Fourier series coefficients (fourier series engineering mathematics)

I Prodigi della TRASFORMATA di FOURIER
Transform Calculus and its applications in Differential Equations
Intro to FOURIER SERIES: The Big Idea
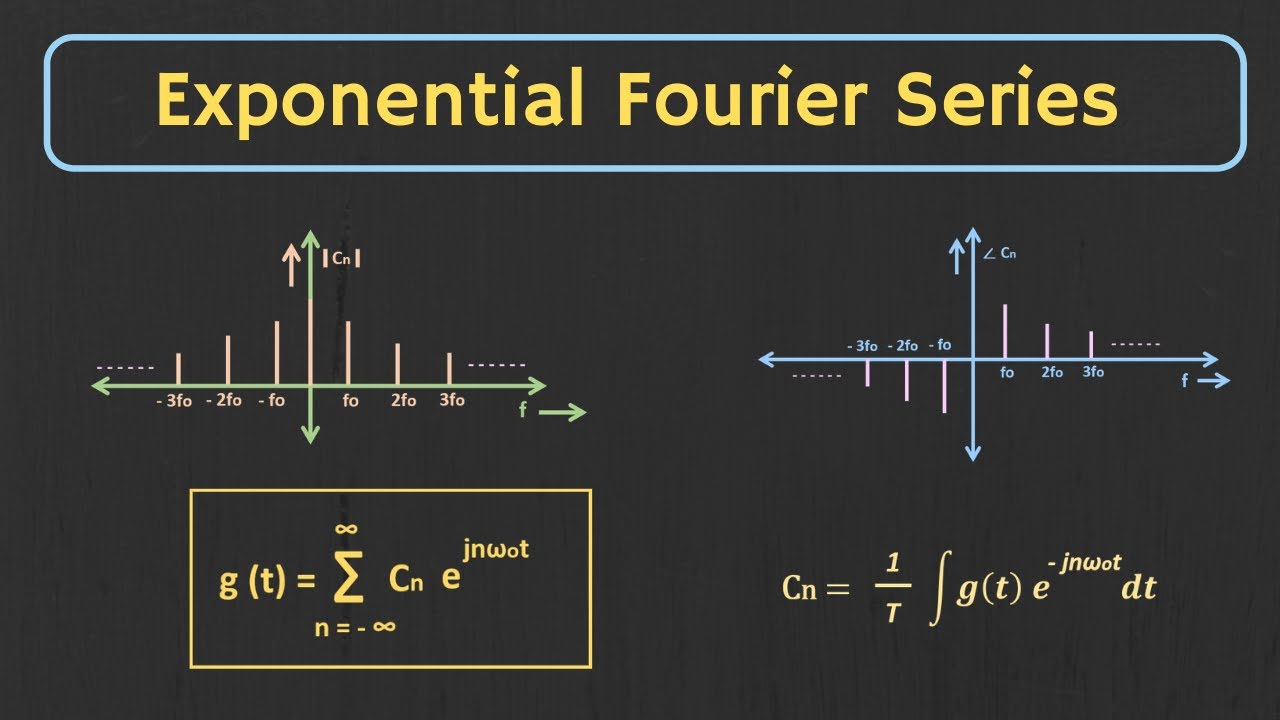
Exponential Fourier Series Explained | Concept of Negative Frequency Explained
5.0 / 5 (0 votes)