Superficie de Revolución (introducción) - Deducción de la Formula

Summary

This video explores the concept of calculating the surface area of a solid formed by rotating a continuously differentiable function around the x-axis. The process begins by approximating the curve's length using partitioned segments and progressively refining the estimate with increasing partitions. The focus shifts to calculating the surface area of the revolution by considering the formation of infinitesimal rings, each approximating the surface. With the use of Pythagoras' theorem, the area of each ring is derived, and the total surface area is obtained through integration. The video provides a clear understanding of the formula used for this calculation.

Takeaways

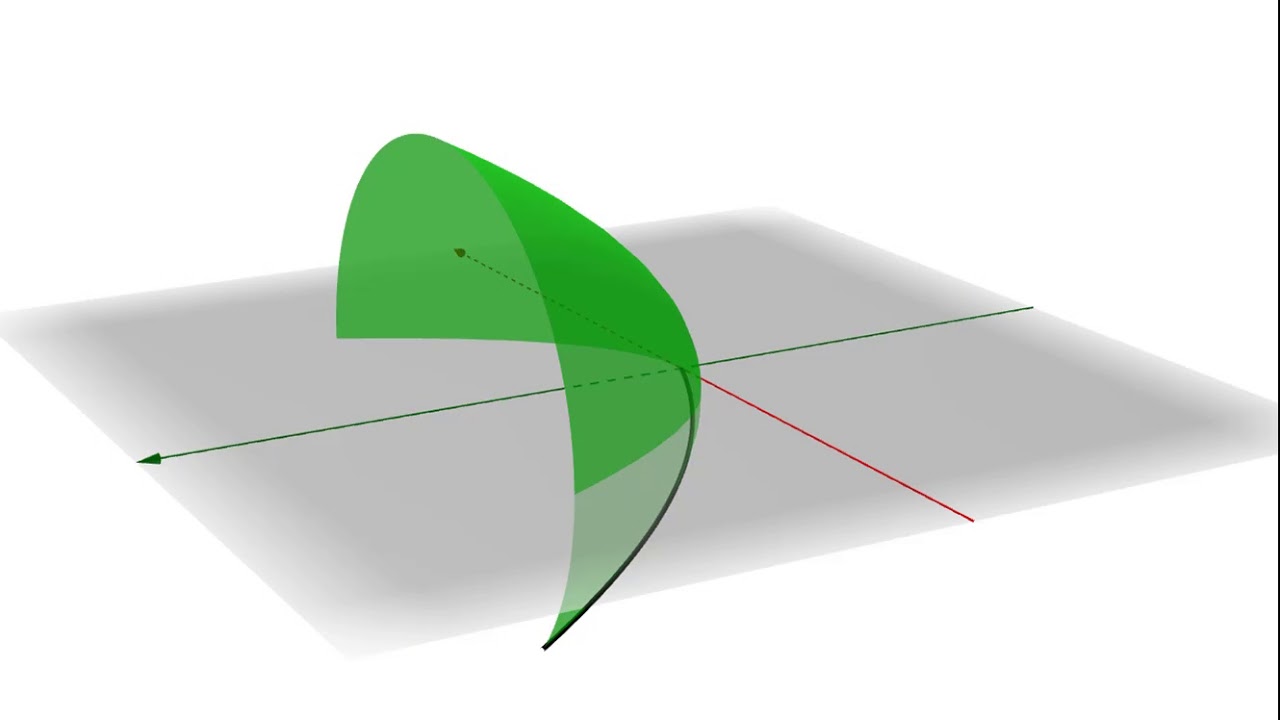
• 😀 The script explains how to calculate the surface area of a solid of revolution generated by rotating a continuous, differentiable function f(x) around the x-axis.
• 😀 To approximate the surface area, the curve is divided into N partitions, each generating a segment (or rope) that approximates the actual curve's length.
• 😀 As the number of partitions increases, the approximation of the surface becomes smoother and more accurate, with the defect (delta) between the curve and the approximating rope shrinking.
• 😀 The surface defect (delta) arises from the fact that a straight line (the rope) cannot perfectly match the shape of the curve unless the number of partitions is infinite.
• 😀 The approach involves dividing the curve into increasingly smaller partitions, and the defect (delta) tends toward zero as the partition size gets infinitesimally small.
• 😀 Each partition forms a ring, and the surface area can be approximated by summing the areas of these infinitesimal rings.
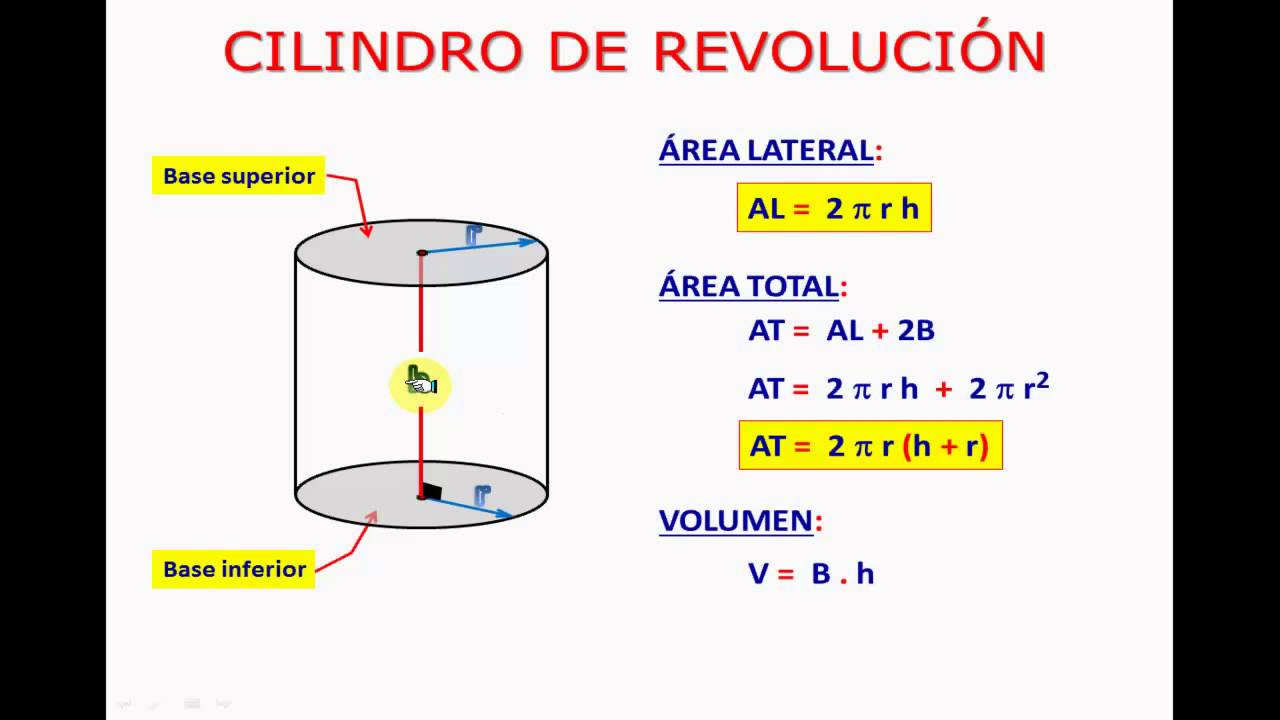
• 😀 The formula for the lateral surface area of a cylinder (2π * radius * height) is applied to calculate the surface of each infinitesimal ring.
• 😀 In the context of the surface of revolution, the radius of each ring is determined by the function f(x), and the height is the distance between adjacent partition points.
• 😀 The final surface area is obtained by integrating the areas of all these infinitesimal rings, ultimately leading to the formula for the surface area of the solid of revolution.
• 😀 The provided formula is used for calculating the surface area in square units, and the entire process demonstrates the transition from an approximation to the exact surface area as the partition size approaches zero.

The video is abnormal, and we are working hard to fix it.
Please replace the link and try again.