SOLVING LOGARITHMIC INEQUALITIES | GRADE 11 GENERAL MATHEMATICS Q1

Summary

This video lesson focuses on solving logarithmic inequalities, explaining essential concepts and providing clear examples. Viewers learn how to determine the relationship between logarithmic expressions based on their bases, whether greater than one or between zero and one. The process of converting logarithmic inequalities to exponential form is demonstrated, along with methods for combining and simplifying expressions. Solutions are expressed in interval notation to clarify the range of valid values for x. The tutorial aims to equip students with the skills needed to tackle logarithmic inequalities confidently.

The video is abnormal, and we are working hard to fix it.
Please replace the link and try again.

Q & A

What is a logarithmic inequality?

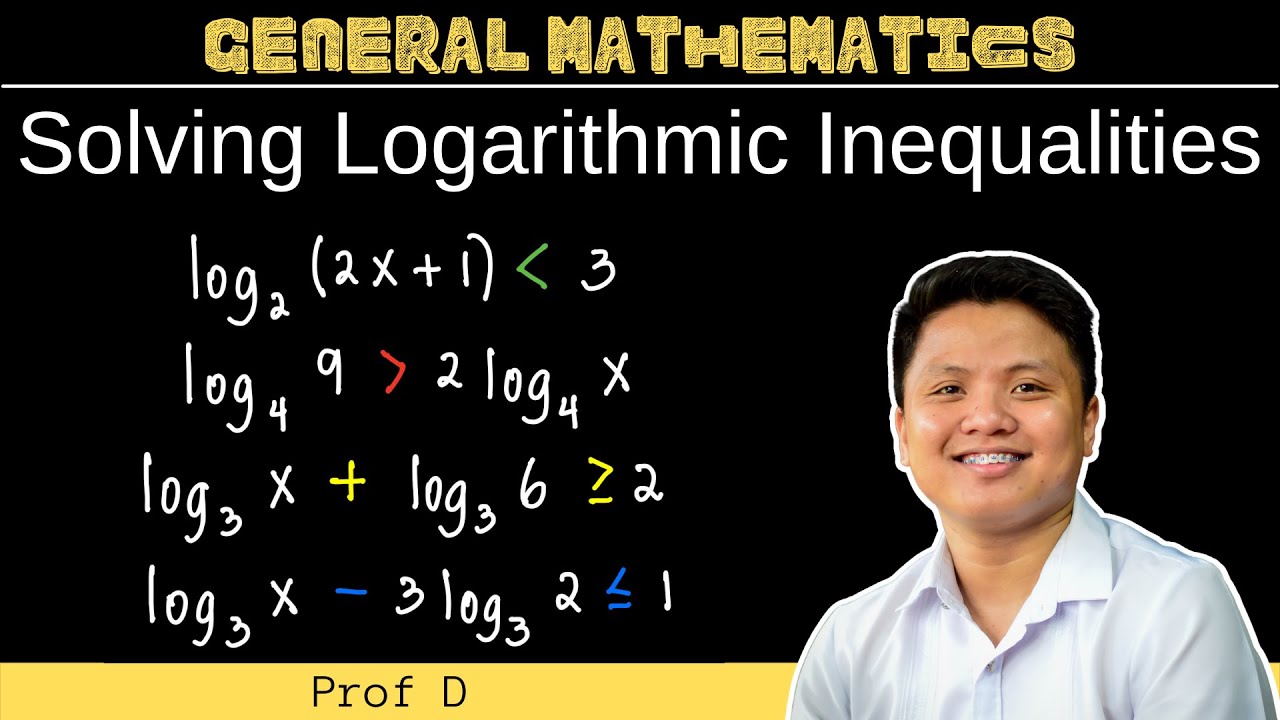
A logarithmic inequality is an inequality that involves logarithms, such as log expressions with different bases and comparison symbols like less than, greater than, or equal to.

How do you solve logarithmic inequalities when the base is greater than 1?

When the base is greater than 1, the inequality symbol retains its direction; if log x1 with base b is less than log x2 with base b, then x1 is less than x2.

What changes when the base of the logarithm is between 0 and 1?

When the base is between 0 and 1, the inequality symbol reverses direction. For example, if log x1 with base b is greater than log x2 with base b, then x1 is less than x2.

In the example log x with base 3 being less than or equal to 5, how is the solution expressed?

The solution involves converting the logarithmic expression to exponential form, yielding x less than or equal to 3 raised to the power of 5, which evaluates to x less than or equal to 243.

What is the importance of ensuring that the logarithmic expressions are defined?

It is crucial that the arguments of logarithmic functions are positive, as logarithms are undefined for non-positive values. This requirement leads to additional conditions in solving inequalities.

How do you express the solution of the inequality in interval notation?

The solution can be expressed in interval notation by indicating the range of valid values, such as (0, 243] for the previous example, meaning x is greater than 0 and less than or equal to 243.

What does it mean when log x is less than log 125 with base 1/5?

Since the base is less than 1, the inequality symbol reverses, leading to the conclusion that x must be greater than 0 and less than 125.

What role does converting logarithmic inequalities to exponential form play in solving them?

Converting to exponential form allows for easier manipulation and simplification of the inequality, making it more straightforward to identify the solutions.

Can logarithmic inequalities include both strict and non-strict inequalities?

Yes, logarithmic inequalities can include both strict inequalities (such as < or >) and non-strict inequalities (such as ≤ or ≥), which affects how solutions are expressed.

Why is it necessary to check for additional conditions when solving logarithmic inequalities?

Additional conditions are necessary to ensure that the arguments of logarithmic expressions remain positive, which impacts the validity of the solutions derived from the inequalities.