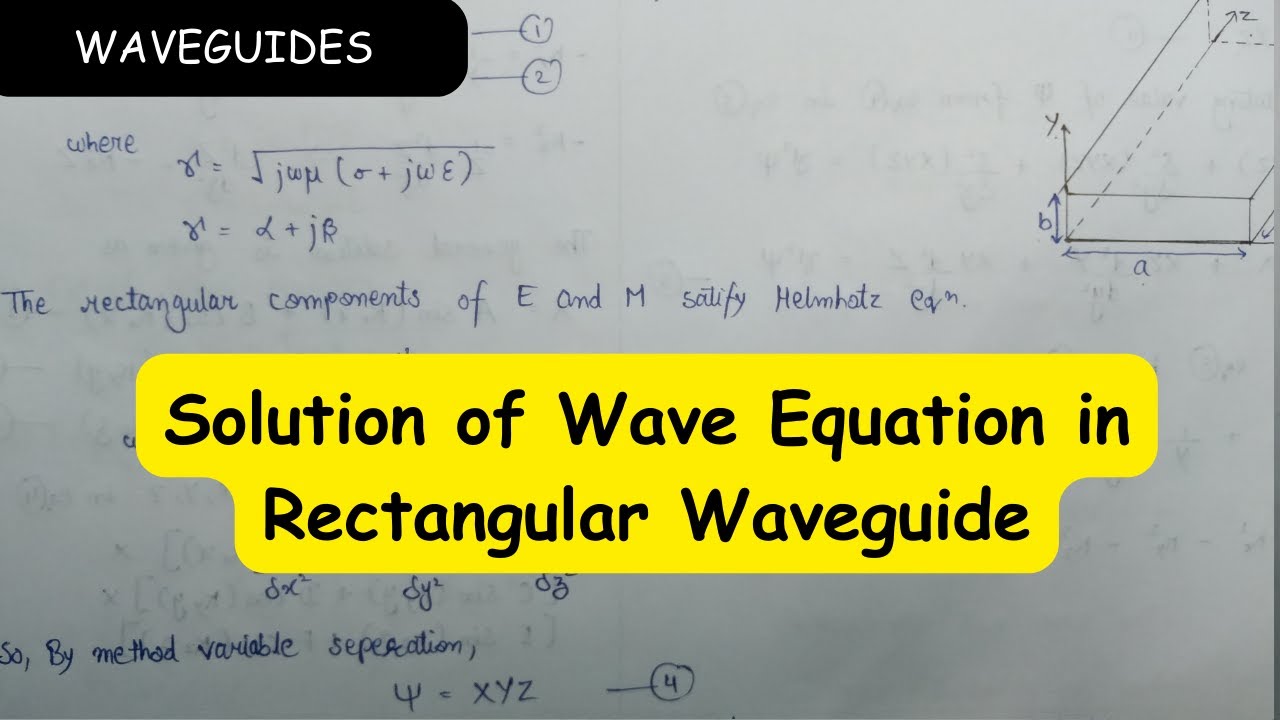
Wave equation and its solutions

Summary

In this lecture, the discussion revolves around the oscillation of particles and their interactions, leading to wave propagation. The lecturer explores how disturbing one particle affects others, creating a continuum and analyzing oscillation patterns in strings. Key concepts include linear density, tension, and wave speed, as well as the mathematical representation of these phenomena. The session emphasizes the relationship between potential and kinetic energy, presenting sine and cosine functions as solutions for oscillating systems. The lecturer concludes by indicating that future discussions will incorporate boundary conditions to solve specific problems.

Takeaways

• 😀 The importance of effective communication skills in both personal and professional settings.
• 😀 Active listening is a crucial component of successful communication.
• 😀 Non-verbal communication, such as body language, significantly impacts how messages are perceived.
• 😀 Clarity and conciseness in speech help in delivering messages more effectively.
• 😀 The use of open-ended questions fosters deeper conversations and understanding.
• 😀 Empathy in communication helps build stronger relationships and trust.
• 😀 Feedback is essential for improving communication skills and addressing misunderstandings.
• 😀 Cultural awareness enhances communication in diverse environments.
• 😀 Conflict resolution techniques are vital for maintaining healthy interpersonal dynamics.
• 😀 Continuous practice and self-reflection can significantly improve communication abilities.

Q & A

What is the significance of oscillations in the context of particles?

Oscillations are significant because they indicate that when one particle is disturbed, other particles coupled with it will also experience disturbances. This behavior leads to the propagation of waves.

How is the propagation of disturbances related to waves?

The propagation of disturbances through a medium, such as sound waves through air, is characterized by oscillations that spread out, creating a wave. The movement of particles leads to the spread of disturbances, which we refer to as waves.

What role does tension play in the behavior of a string under oscillation?

Tension in a string is essential as it influences the oscillation patterns. A uniform tension allows for consistent oscillatory motion, and the wave speed is dependent on both the tension and the linear density of the string.

What are the components of forces acting on an oscillating string?

The forces acting on an oscillating string include horizontal components of tension that balance each other and vertical components that provide restoring forces. These forces lead to the vertical oscillations of the string segments.

How does the assumption of small amplitude oscillations simplify the analysis?

Assuming small amplitude oscillations allows us to use approximations, such as sin(d) being approximately equal to d when d is small. This simplifies calculations and helps in deriving the wave equation.

What is the derived wave equation for a string in oscillation?

The derived wave equation for a string in oscillation is represented as T * (∂²y/∂x²) = μ * (∂²y/∂t²), where T is the tension, μ is the linear density, and y is the displacement of the string.

What does the wave speed depend on in a string?

The wave speed (c) in a string depends on the tension (T) and the linear density (μ) of the string, expressed as c = √(T/μ).

What kind of functions describe the oscillation of a string?

The oscillation of a string can be described using sine and cosine functions. These functions represent the displacement of the string at different points and times.

What is the general solution for the displacement of a string?

The general solution for the displacement of a string can be written as y(x, t) = A * sin(kx - ωt) or y(x, t) = A * cos(kx - ωt), where A is the amplitude, k is the wave number, and ω is the angular frequency.

How do boundary conditions influence the solutions of wave equations?

Boundary conditions are crucial as they define the specific constraints for a physical problem. They determine the form of the solutions to wave equations, allowing for unique solutions that fit the physical situation.