8.02x - Module 12.01 - EM Plane Waves - Poynting Vector - E-fields - B fields - Wavelength

Summary

The video script discusses the fundamental principles of traveling electromagnetic waves, emphasizing the importance of Maxwell's equations and their consequences. It explains how the electric field vector (E) and magnetic field vector (B) are perpendicular to each other and the direction of propagation, always in phase, and how the amplitude of the magnetic field (B0) is related to the electric constant (ε0) and the speed of light (c). The script provides a detailed example of calculating properties of an electromagnetic wave with a frequency of 4.0 * 10^13 Hz, including its wavelength, wave number, and direction of propagation. It also illustrates how to determine the direction of the electric field vector when given the magnetic field vector's oscillation and propagation direction, highlighting the significance of the signs in the cosine function for wave propagation.

Takeaways

• 🧲 Maxwell's Equations: The lecturer emphasizes the importance of Maxwell's equations in understanding traveling electromagnetic waves.
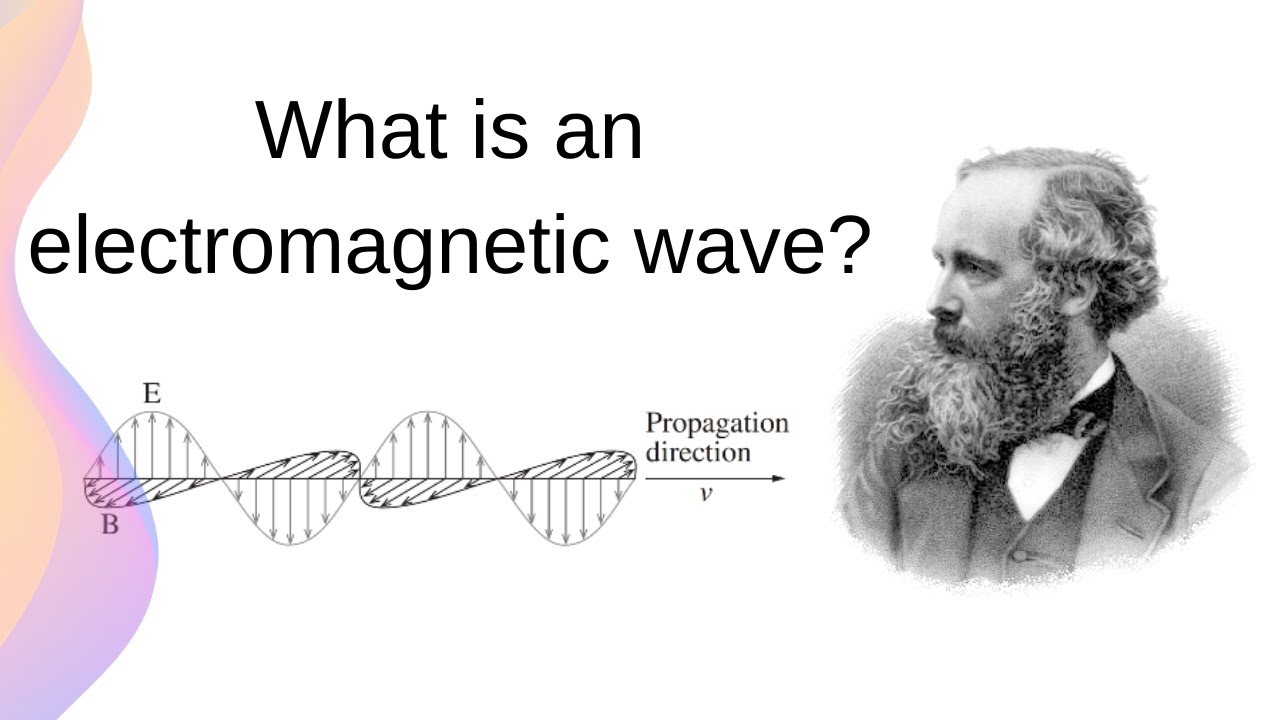
• 🌐 Wave Propagation: The E vector and B vector are always perpendicular to each other and to the direction of wave propagation.
• 🔄 Phase Relationship: E and B are in phase, meaning they reach their maximum and zero values at the same moments in time.
• 🌌 Electromagnetic Wave in Vacuum: The amplitude of the magnetic field (B0) is related to the amplitude of the electric field (E0) through the equation B0 = E0/c.
• 🚀 Energy Flux: The energy per second flowing through each square meter is given by the cross product of E and B vectors divided by the magnetic constant (μ0).
• 🌀 Wave Characteristics: The wavelength (Lambda) is related to the speed of light (c) and the period of oscillation, and the wave number (k) is the reciprocal of the wavelength.
• 🔢 Frequency and Wavelength: Given a frequency of 4.0 * 10^13 Hz, the angular frequency (Omega) and wavelength (Lambda) are calculated, identifying the wave as infrared radiation.
• 📍 Direction of Propagation: The direction of wave propagation is specified as the positive Y direction, with the B vector oscillating in the X direction.
• 📉 Oscillation and Propagation: The B vector's oscillation is described by a cosine function that includes the angular frequency and wave number, crucial for the wave's directionality.
• 🛑 Significance of Signs: The correct signs in the cosine function are essential for the correct direction of wave propagation; changing signs alters the direction.
• 🔄 Changing Propagation Direction: To change the direction of wave propagation, one must adjust the signs in the cosine terms and ensure the direction of E cross B is consistent with the new direction.

Q & A

What are the fundamental properties of electromagnetic waves as described in the script?

The script describes that the electric field vector (E) is always perpendicular to the magnetic field vector (B), and both are perpendicular to the direction of propagation. Additionally, E and B are always in phase, meaning they reach their maximum and zero values at the same moments in time.

What is the relationship between the amplitude of the magnetic field (B0) and the electric field (E0) in a vacuum?

In a vacuum, the amplitude of the magnetic field (B0) is related to the electric field (E0) by the equation B0 = E0/c, where c is the speed of light.

What does the pointing vector represent and how is it calculated?

The pointing vector represents the amount of energy per second flowing through each square meter of a particular surface. It is calculated as the cross product of the electric field vector (E) and the magnetic field vector (B), divided by the permeability of free space (μ0), or E x B / μ0.

What is the significance of the wave number (k) and how is it related to the wavelength (λ)?

The wave number (k) is a measure of the number of wavelengths per unit length and has units of 1/meter. It is related to the wavelength (λ) by the equation k = 2π/λ.

How is the frequency of electromagnetic radiation related to its wavelength?

The frequency (f) of electromagnetic radiation is inversely related to its wavelength (λ). This relationship is given by the equation λ = c/f, where c is the speed of light.

What is the significance of the angular frequency (ω) in the context of the script?

The angular frequency (ω) is related to the frequency of the electromagnetic wave and is used to describe the rate of oscillation. It is calculated as ω = 2πf, where f is the frequency of the wave.

What is the frequency given in the script, and how does it relate to the angular frequency and wavelength?

The frequency given in the script is 4.0 * 10^13 Hz. The angular frequency (ω) is then 2.5 * 10^14 rad/s, and the wavelength (λ) is 7.5 * 10^-6 m, which corresponds to infrared radiation.

What is the direction of propagation (V) and the oscillation direction of the magnetic field (B) vector as described in the script?

The direction of propagation (V) is in the positive Y direction. The magnetic field (B) vector oscillates in the X direction, as indicated by the cosine function with a time-dependent term and a spatial term with a wave number k.

Why is the sign in front of the wave number (k) in the expression for the magnetic field (B) vector crucial?

The sign in front of the wave number (k) is crucial because it determines the direction of wave propagation. A positive sign indicates propagation in the positive Y direction, while a negative sign would indicate propagation in the negative Y direction.

How does the direction of the electric field (E) vector relate to the magnetic field (B) vector and the direction of propagation (V)?

The electric field (E) vector must be in a direction such that the cross product of E and B is in the direction of propagation (V). In the script's example, E is in the positive Z direction, ensuring that E x B is in the positive Y direction, consistent with the wave propagating in the positive Y direction.

What changes would be necessary to change the direction of propagation from positive Y to negative Y?

To change the direction of propagation from positive Y to negative Y, the signs within the cosine terms for both E and B would need to be changed to be the same (both positive or both negative), and the direction of the electric field (E) vector would need to be adjusted so that E x B is in the negative Y direction.

Outlines

00:00

🌌 Electromagnetic Waves and Maxwell's Equations

The first paragraph introduces the concept of traveling electromagnetic waves, emphasizing the importance of Maxwell's equations in understanding their behavior. The speaker admits to knowing few equations but being able to derive many from those known. Key points include the perpendicularity of the electric (E) and magnetic (B) vectors to the direction of propagation, their in-phase nature, and the formula for the amplitude of the magnetic field in vacuum (B0 = E0 * c). The energy flux, given by the cross product of E and B divided by the magnetic constant (μ0), is also discussed, along with the relationship between wavelength (λ), speed of light (c), and frequency (f). The paragraph concludes with an example problem involving an infrared wave with a given frequency, direction of propagation, and magnetic field oscillation.

05:03

📏 Directionality and Propagation of Electromagnetic Waves

The second paragraph delves into the specifics of wave propagation direction, highlighting the crucial role of the sign in the wave equation. It explains how a change in sign affects the direction of wave propagation, using the cosine function to illustrate the relationship between time (t), angular frequency (ω), and wave number (k). The paragraph also discusses the importance of maintaining the right-handed coordinate system and the perpendicular relationship between the electric and magnetic fields. It concludes with a practical example of how to determine the direction of the electric field vector (E) based on the given magnetic field vector (B) and propagation direction, ensuring that E x B aligns with the direction of propagation.

10:05

🔄 Changing the Propagation Direction of Electromagnetic Waves

The third paragraph focuses on altering the direction of wave propagation. It explains that changing the signs within the cosine terms is not sufficient; one must also ensure that the cross product of the electric and magnetic fields (E x B) changes direction accordingly. The paragraph provides a step-by-step guide on how to adjust the signs to achieve the desired propagation direction, emphasizing the need to consider the overall orientation of E x B. It concludes with a hypothetical scenario where the wave is made to propagate in the opposite direction, illustrating the necessary sign changes.

Keywords

💡Electromagnetic Wave

An electromagnetic wave is a wave that carries energy through space, composed of alternating electric and magnetic fields that are perpendicular to each other and to the direction of travel. In the video, the concept is central to the discussion of traveling waves, with the script explaining how these waves propagate in a vacuum and how their properties are derived from Maxwell's equations.

💡Maxwell's Equations

Maxwell's equations are a set of four fundamental equations in electromagnetism that describe how electric and magnetic fields are generated and altered by each other and by charges and currents. The script mentions them as the basis for deriving properties of traveling electromagnetic waves, emphasizing their importance in physics.

💡Perpendicularity

In the context of the script, perpendicularity refers to the relationship between the electric field vector (E) and the magnetic field vector (B) in an electromagnetic wave, which are always perpendicular to each other. This concept is crucial for understanding the orientation of these fields in the propagation of the wave.

💡Direction of Propagation

The direction of propagation is the direction in which the electromagnetic wave travels. The script specifies that both the electric and magnetic fields are perpendicular to this direction, and it uses the plus Y direction as an example to illustrate how the wave's properties are described in relation to its propagation.

💡Amplitude

Amplitude in the context of waves refers to the maximum extent of a vibration or oscillation, measured from the position of equilibrium. In the script, the amplitudes of the electric and magnetic fields are discussed, particularly how they relate to the energy carried by the wave and how they oscillate in phase with each other.

💡Energy Flux

Energy flux is the rate at which energy is transferred through a given area, measured in watts per square meter. The script describes how the energy flux of an electromagnetic wave is calculated using the cross product of the electric and magnetic field vectors divided by the magnetic permeability of free space (mu0).

💡Wavelength

Wavelength is the distance between two consecutive points in a wave that are in the same phase. The script introduces the concept of wavelength (Lambda) and explains how it is related to the speed of light (c) and the period of oscillation, emphasizing its importance in characterizing the wave.

💡Wave Number

The wave number (k) is a measure of the spatial frequency of a wave, expressed in units of inverse meters. In the script, it is introduced as 2π/Lambda, which relates to the wavelength of the electromagnetic wave and is used to describe its spatial characteristics.

💡Angular Frequency

Angular frequency (Omega) is a measure of how fast an object undergoes rotational motion, expressed in radians per second. The script calculates it from the frequency of the wave and uses it to describe the oscillation of the electric and magnetic fields in the wave.

💡Phase

Phase in the context of waves refers to the position of a point in time within a wave cycle. The script explains that the electric and magnetic fields of an electromagnetic wave are always in phase, meaning they reach their maximum and minimum values at the same moments in time.

💡Poynting Vector

The Poynting vector is a quantity that represents the directional energy flux density of an electromagnetic field. The script mentions it in the context of describing how much energy per second flows per square meter out of a particular surface, which is crucial for understanding the propagation of electromagnetic waves.

Highlights

Maxwell's equations are fundamental to deriving properties of traveling electromagnetic waves.

Electromagnetic waves are characterized by the E vector being perpendicular to the B vector.

Both E and B vectors are perpendicular to the direction of wave propagation.

E and B vectors are in phase, reaching maximum and zero values simultaneously.

The amplitude of the magnetic field (B0) is related to the speed of light in a vacuum.

The energy flux is given by the cross product of E and B vectors divided by the permeability of free space (μ0).

The wavelength (λ) is related to the speed of light (c) and the period of oscillation.

Introduction of wave number (k) as a unit of 1/meter, derived from the wavelength.

The angular frequency (ω) is directly proportional to the wave number (k) and the speed of light.

A frequency of 4.0 * 10^13 Hz is given, leading to specific calculations for ω and λ.

The direction of wave propagation is specified as the positive Y direction.

The B vector oscillates in the X direction with a given angular velocity (ω).

The importance of the correct sign in the wave equation for proper propagation direction.

The relationship between the E and B vectors and their phase synchronization.

The E vector is determined to be in the positive Z direction based on the right-hand rule.

A step-by-step explanation of how to determine the direction of the E vector in relation to B and propagation direction.

Changing the direction of wave propagation requires adjusting both the signs in the wave equation and the direction of E cross B.

Transcripts

problem one is

335 it deals with a

traveling electromagnetic

wave now I told you earlier and I wasn't

joking that I know very few equations in

physics I can derive many from the few

that I know I know Maxwell's equation s

yes I do certainly after this

course I also know some of the

consequences of Maxwell's equations

related to traveling waves and I will

share those with you and if you remember

those because it would be rather silly

to derive them every time then it's very

easy to write down traveling

electromagnetic waves with the vectors

in the right direction and the

amplitudes all correct and the plus and

minus signs correct the first thing that

I happen to remember is that the E

Vector is always perpendicular to the B

Vector the second is that e as well as

B are both perpendicular to the

direction of

propagation the third is that e and B

both are in face at all moments in time

what does that mean it means if one

reaches a maximum value the other

reaches a maximum value at the same

moment in time of course at right angles

and if one goes through zero the other

goes through zero that's what it means

always in

phase and then B 0 which is the

amplitude of the magnetic field Factor

equals e0 ided c when the electr

magnetic radiation is in

vacuum

now independent of electromagnetic waves

what always holds is that the pointing

Vector which tells you how much energy

per second flows per square

meter out of a particular surface equals

e cross B divided by mu0 so this is

energy per second flowing through each

square meter and I will call that flux

it's an energy

flux what also so always

holds Lambda equals c * the period of

one oscillation don't confuse this T's

Tesla so this is also C divided by the

frequency of the radiation

F what you will often see introduced is

a wave number K which has nothing to do

with the unit Vector in the Z Direction

nothing that k equals 2 Pi / Lambda and

that has as a unit 1 over meter now

since Omega equals 2 pi * the frequency

f it follows immediately that Omega

divided by K also is the speed of light

the speed with which electromagnetic

radiation propagates itself in vacuum

now if you can remember all these things

that would help I happen to remember

them we have here in problem one we have

a frequency which is

4.0 * 10 13

Hertz so it follows immediately that

Omega equals

2.5 * 10

14th radians per

second and it follows that Lambda equals

7.5 * 10- 6 M 75,000 angstroms if you

like that unit this is infrared

radiation we're also being told that the

direction of V is in plus

Y and we're being told that the B Vector

oscillates in the direction of X plusus

plusus plus minus with that angular

velocity

Omega and you know b0 whatever that is

so the B vector

if we write that down in terms of X Y

and Z and all moments in time would be

the amplitude of the B Vector times

cosine Omega T which indicates the

oscillatory character Min - k

y in the X

Direction and K then here mean simply 2

Pi / Lambda if you want to write for

this k 2 Pi / by Lambda be my guest now

let's look at these

things this minus sign is absolutely

crucial If This Were A plus sign the

wave would not be propagating in the

plus y direction and you can immediately

check that for yourself increase T by a

teeny weeny little bit if you want the

cosine function to be exactly the

same you would have to increase y by a

teeny weeny little bit so that Omega T

minus KY Remains the

Same so for this wave to propagate in

the plus y direction it is important

that this sign here and this sign here

have an opposite sign if they both have

the same sign minus minus or plus plus

then the wave would run in the minus y

direction and this X tells you simply

that the B Vector oscillates in the X

Direction sometimes plus sometimes

minus well Omega you already know if you

are interested in K by any chance then I

believe that is

8.38 * 10 5 m minus

1 now since you know b0 you also know e0

because e0 equals B 0 *

C so if we write down now the E Vector

as a function of XYZ and T then we would

get e z here which you

know and then I get exactly this

same argument Omega T minus

KY why because I mentioned earlier that

e and B are always at any moment in time

exact in PH and that's only possible if

this is identical to

that now comes the question what is the

direction that I should put here what is

the unit Vector that I should put here

and for that I'm going to make a

drawing and I'm going to make a drawing

of a right-handed coordinate system

always make a drawing of a right-handed

coordinate system and a right-handed

coordinate

system is a coordinate system whereby X

cross y equals z don't even think of

ever doing it in another way because you

get into deep

trouble so let this be

X and let this be

y then

this would be Z convince yourself that X

cross y would then be

Z now pick any moment in time and let us

assume that the B Vector is in this

direction of course it's not only along

the X Direction in this direction but

since it's a Plaine wave it's everywhere

here the same magnitude and the same

direction and this wave propagates to

the

right and a little later in time half a

period in time if assume that this is

the maximum value B possible in this

direction

half a period in time it will be

pointing down and a quarter period in

time it will go through zero and they

will all go through zero at exactly the

same

time in what direction at this moment in

time should I now Point

e so that and this is one of

my statements that I made earlier so

that e cross B is in the direction of V

which means in this case in the

direction of positive y

well that should not give you any

problems the only way that you can do

that is when e is in the

plus Z Direction convince yourself that

only then is e cross B in the plus y

direction so that means that if we now

have to finish this Vector notation for

E we have to write down here a z roof

and it's all done I canot asked the

question suppose we wanted to move that

wave in the other direction not in plus

y but in minus y what would we have to

change well we could change this minus

sign to a plus sign and this minus sign

to a plus sign what count is actually is

not so much that this becomes a plus but

that these two signs here and here are

the

same but that's not enough if you want e

cross B to be in the opposite direction

you also must either put a minus sign

here so you get a minus X and leave the

plus here or you put a minus sign here

in the minus Z Direction and leave the

plus there so whenever you have to

change the direction of propagation keep

in mind that it's not enough to only

change the signs inside the brackets of

the cosine terms but you also have to

take into account that e cross B must

change direction