FISIKA VEKTOR KELAS XI [FASE F] PART 1 - KURIKULUM MERDEKA
Summary
TLDRThis educational script introduces the fundamental concepts of vectors and scalars, emphasizing the importance of understanding their differences. It explains that scalars have magnitude without direction, exemplified by mass, while vectors have both magnitude and direction, illustrated with potential energy. The script delves into vector description, showing how to represent them with arrows and points, and discusses methods for vector addition and subtraction, including the triangle and parallelogram methods. It also covers the cosine formula for determining the magnitude of the resultant vector, providing an example problem to solidify the concepts.
Takeaways
- 📚 The first objective of the lesson is to understand vector calculation operations using various methods.
- 🔍 The second objective is to identify vector components and describe vectors in detail.
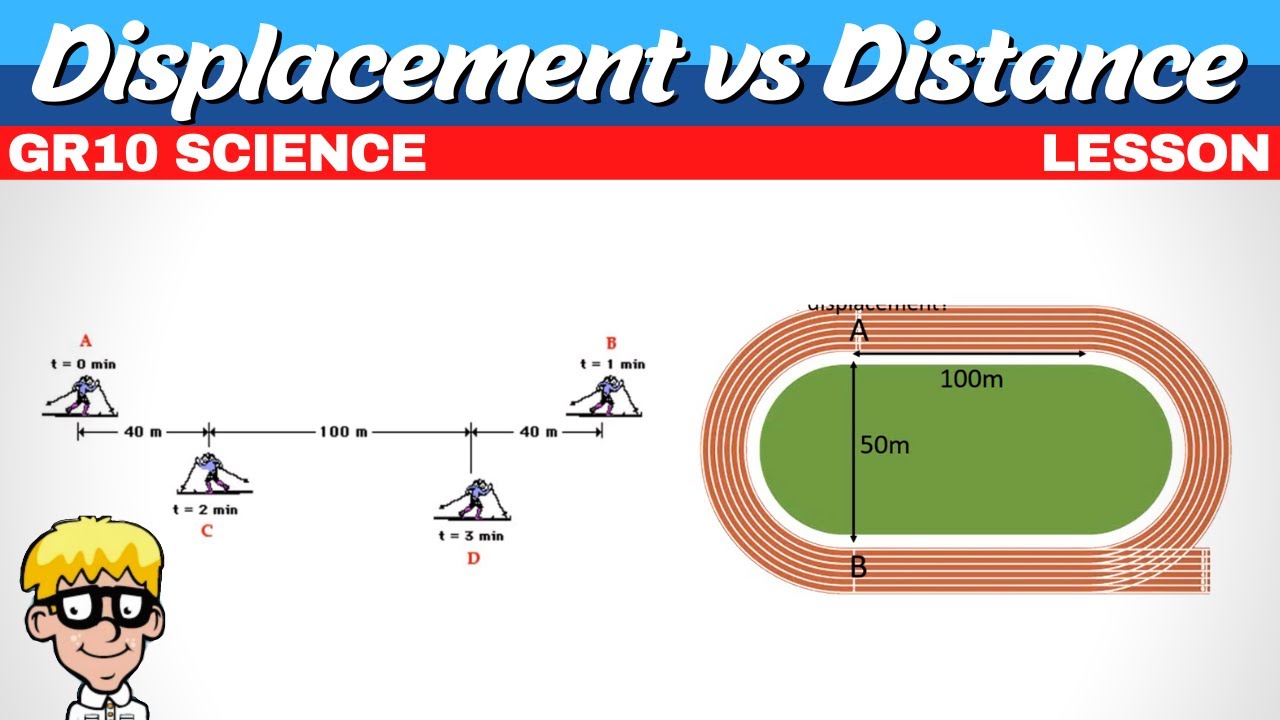
- 📏 Scalars are quantities with magnitude but no direction, such as mass, which is exemplified by 10 kg of rice.
- 🧭 Vectors are quantities with both magnitude and direction, differing from scalars by having directional properties.
- 🦏 An example of a vector is potential energy, demonstrated by an elephant moving from top to bottom, indicating a directional movement.
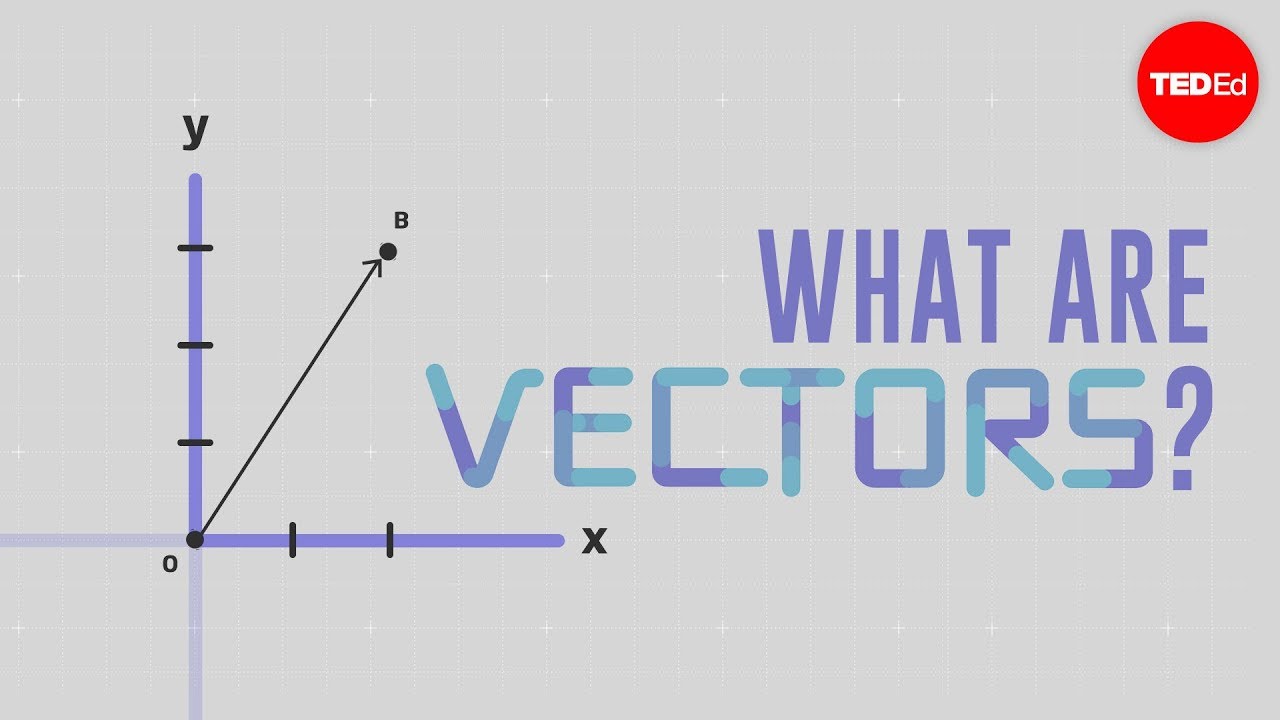
- 📐 Vectors are described by their starting point (anchor point), ending point (tip), magnitude, and direction, often represented with an arrow.
- 📉 The magnitude of a vector is its length, and the direction is usually given in degrees, like a vector AB with a magnitude of 50 meters and a direction of 30°.
- ✍️ Vectors are written with a symbol and a size, where the symbol is marked with an arrow, such as acceleration or force vectors.
- 🔄 Vectors can be added or subtracted using methods like the triangle method, parallelogram method, and polygon method to find the resultant vector.
- ➡️ The triangle method involves arranging vectors head-to-tail to form a triangle and finding the resultant as the diagonal.
- 🟪 The parallelogram method involves placing vectors tail-to-head to form a parallelogram, with the resultant being the diagonal.
- 🔢 The cosine formula is used to determine the magnitude of the resultant vector, expressed as \( r = \sqrt{a^2 + b^2 + 2ab \cdot \cos(\alpha)} \), where \( a \) and \( b \) are vector magnitudes and \( \alpha \) is the angle between them.
- 📌 Understanding the cosine formula and special angle trigonometric values is essential for solving vector problems.
Q & A
What is the fundamental difference between scalar and vector quantities?
-Scalar quantities have only magnitude without direction, such as mass. Vector quantities have both magnitude and direction, such as velocity.
Can you provide an example of a scalar quantity mentioned in the script?
-An example of a scalar quantity given in the script is the mass of rice weighing 10 kg, which has magnitude but no direction.
How is a vector quantity described in the script?
-A vector quantity is described by its magnitude and direction. For instance, the script describes the movement of an elephant from top to bottom as a vector quantity because it has a direction.
What are the two main components of a vector mentioned in the script?
-The two main components of a vector are its magnitude and direction. The script uses the example of vector OB where OB represents both the magnitude and the direction of the vector.
How can the magnitude and direction of a vector be represented?
-The magnitude of a vector can be represented by the length of the vector, and the direction can be represented by an angle, as shown in the script with vector AB having a magnitude of 50 meters and a direction of 30°.
What is the significance of the anchor point and the tip of the vector in describing vectors?
-The anchor point is the starting point of the vector, and the tip is the endpoint, usually marked with an arrow to indicate direction. Knowing these helps in the correct depiction and understanding of vector directionality.
According to the script, how can two vectors be considered the same?
-Two vectors are considered the same if they have the same magnitude and direction, as explained in the script with the example of two vectors both having a magnitude of 50 and a direction of 30°.
What is the triangle method for adding vectors as described in the script?
-The triangle method for adding vectors involves placing the vectors head-to-tail to form a triangle, with the resultant vector being the third side of the triangle, as demonstrated with vectors A and B in the script.
What is the parallelogram method for adding vectors, and how does it differ from the triangle method?
-The parallelogram method for adding vectors involves placing the vectors tail-to-tail to form a parallelogram, with the resultant vector being the diagonal of the parallelogram. It differs from the triangle method in the arrangement of the vectors and the shape formed.
How does the script explain the concept of vector subtraction?
-The script explains vector subtraction by considering one vector as having a negative value, effectively reversing its direction, and then adding it to the other vector using the parallelogram method to find the resultant vector.
What is the cosine formula used for in the context of vector addition, and how is it applied?
-The cosine formula is used to determine the magnitude of the resultant vector when adding two vectors. It is applied using the formula R = √(A² + B² + 2ABcos(α)), where A and B are the magnitudes of the vectors and α is the angle between them, as demonstrated in the script with an example problem.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тариф5.0 / 5 (0 votes)