How To Solve Any Projectile Motion Problem (The Toolbox Method)

Summary

In this video, Jesse Mason tackles a classic projectile motion problem, focusing on calculating range, peak height, and velocity for a projectile launched from uneven ground. Using kinematic equations, Mason walks viewers through determining time, horizontal and vertical displacement, velocity components, and the trajectory's peak. He highlights the importance of breaking down motion into horizontal and vertical components and uses trigonometry and the quadratic formula to solve the problem. This tutorial emphasizes the need for a 'toolbox' of equations when approaching projectile motion challenges.

Takeaways

• 📐 Projectile motion problem involves determining the range, peak height, and velocity over time.
• 🖼️ The initial setup includes drawing a diagram of a projectile launched from a cliff at a 20° angle with an initial speed of 30 m/s.
• 📏 The projectile starts at an initial height of 100 meters, and gravity is set at -9.8 m/s².
• 🔄 The key unknowns are the horizontal range (x), the maximum vertical height (y-max), and the velocity at 2 seconds post-launch.
• 🔧 To solve, use kinematic equations separately for x and y directions, assuming no air resistance.
• ⏲️ The time of impact is solved using the quadratic formula, yielding a positive value of 5.68 seconds.
• 🏁 The horizontal range of the projectile upon impact is calculated to be 160.1 meters.
• 📊 The projectile reaches its peak height of 105.4 meters at 1.05 seconds after launch.
• 📉 At 2 seconds, the projectile’s velocity is 29.7 m/s with a downward angle of 18.3 degrees.
• 🔨 Using a toolbox of equations simplifies projectile motion analysis, making the process more systematic and organized.

Q & A

What is the main focus of this Teach Me installment?

The main focus is solving a classic projectile motion problem involving an angled launch from uneven ground.

What are the key parameters given in the problem?

The key parameters include the initial vertical displacement (height of the cliff: 100 meters), the magnitude of the launch velocity (30 meters per second), and the launch angle (20 degrees).

What is the first step in solving the projectile motion problem?

The first step is to draw a diagram illustrating the cliff, the level ground below, the cannon's initial position, and the projectile's trajectory.

How is the acceleration due to gravity represented in the problem?

The acceleration due to gravity is represented by the value -9.8 meters per second squared, since the positive y-axis is aligned upwards and gravity acts downward.

Which equations are used to solve for the projectile’s range and peak height?

The kinematic equations for displacement and velocity in both the x- and y-directions are used. Specifically, equation (1) is used to calculate the horizontal displacement (range), and equation (3) is used to calculate the vertical displacement (peak height).

How is time calculated for when the projectile hits the ground?

Time is calculated by solving equation (3) for vertical displacement (y = 0) using the quadratic formula. The positive time value of 5.68 seconds is used.

How is the range of the projectile determined?

The range is determined by plugging the calculated time of 5.68 seconds into equation (1), yielding a horizontal displacement of 160.1 meters.

How is the peak height of the projectile determined?

The peak height is determined by first calculating the time at which the vertical velocity is zero (t = 1.05 seconds) using equation (4), and then inserting this time into equation (3), which gives a peak height of 105.4 meters.

How is the velocity 2 seconds after launch calculated?

The velocity 2 seconds after launch is calculated by using equation (2) for the horizontal component (v-sub-x = 28.19 m/s) and equation (4) for the vertical component (v-sub-y = -9.34 m/s).

How is the magnitude and direction of the velocity 2 seconds after launch determined?

The magnitude of the velocity is determined using Pythagoras' Theorem, yielding 29.70 meters per second. The direction is calculated using the inverse tangent of the velocity components, giving an angle of 18.3 degrees below the horizontal.

Outlines

00:00

🎯 Introduction to Projectile Motion Problem

Jesse Mason introduces a classic projectile motion problem involving an angled launch from uneven ground. The goal is to determine the projectile's range, peak height, and velocity at a specific time. Jesse begins by setting up the problem visually, describing the initial position, velocity, and drawing a cannon firing from a cliff, marking key components like the angle of launch and coordinate system.

05:08

📐 Known and Unknown Variables

Jesse outlines the known variables, including the height of the cliff (100 meters), initial speed (30 m/s), and launch angle (20 degrees). He discusses the need to explicitly define the coordinate system, highlighting the initial vertical displacement and setting up the diagram. The unknowns include the horizontal displacement (range), peak height, and the velocity at 2 seconds after launch. He breaks the initial velocity into x and y components, labeling these and emphasizing the importance of using the correct trigonometric relationships.

10:13

🔧 Choosing the Right Equations for Projectile Motion

The next step involves selecting the appropriate kinematic equations to solve the problem. Jesse explains the need to break the motion into x and y components, applying Galileo’s method. He simplifies the equations for the x-direction, noting that horizontal acceleration is zero, allowing him to derive simplified equations for both horizontal displacement and velocity. These will be used to find the projectile’s range.

🧮 Applying Equations to the Y-Direction

Moving to the y-direction, Jesse applies the kinematic equations with gravitational acceleration (g = -9.8 m/s²). He rewrites the displacement and velocity equations, incorporating the projectile’s vertical velocity and the effects of gravity. He outlines the steps to determine time using the quadratic formula and how to use this time to find the horizontal displacement (range). After simplifying, Jesse calculates the projectile’s range as 160.1 meters.

⏱ Calculating Peak Height and Time

Jesse now focuses on determining the peak height of the projectile’s trajectory. He explains how to calculate the time at which the projectile reaches its maximum height by setting the vertical velocity to zero. Using this time (1.05 seconds), Jesse inserts the values into the y-direction displacement equation and calculates the peak height to be 105.4 meters.

📏 Calculating Velocity at a Specific Time

Jesse concludes by calculating the projectile’s velocity 2 seconds after launch. He calculates the horizontal (v_x = 28.19 m/s) and vertical components (v_y = -9.34 m/s), where the negative sign indicates downward motion. Using Pythagoras’ theorem, Jesse combines the components to find the overall velocity magnitude (29.70 m/s) and the angle of the velocity vector (18.3 degrees below the horizontal).

🎉 Final Thoughts and Summary

Jesse wraps up by emphasizing the importance of building a toolbox of equations for solving projectile motion problems. He encourages viewers to practice and apply the steps outlined, and invites suggestions for future videos. The episode concludes with a lighthearted sign-off, encouraging engagement in the comments section.

Keywords

💡Projectile motion

Projectile motion refers to the movement of an object that is launched into the air and is subject to gravitational force. In the video, the instructor uses a cannonball as the projectile, showing how it moves through space after being launched from a cliff. The main task is to determine its range, peak height, and velocity at different points in its trajectory.

💡Range

Range is the horizontal distance a projectile travels before hitting the ground. In this video, determining the projectile's range is a key objective. The instructor calculates the range by first solving for the time of impact and then using it to find how far the projectile moves horizontally.

💡Peak height

Peak height is the maximum vertical displacement reached by the projectile. The instructor calculates this by solving for the time when the projectile’s vertical velocity is zero, which corresponds to the peak of its trajectory. This is a critical part of understanding how high the projectile goes during its flight.

💡Velocity

Velocity refers to the speed of the projectile in a given direction. The video examines the initial launch velocity, its horizontal and vertical components, and the velocity at different times during the projectile's flight. The instructor uses both the horizontal and vertical components to calculate the overall velocity two seconds after launch.

💡Kinematic equations

The kinematic equations are a set of equations that describe the motion of objects. In the video, these equations are used to analyze both the horizontal and vertical components of the projectile's motion. The instructor simplifies these equations to apply them to the specific conditions of projectile motion, such as neglecting air resistance.

💡Gravity (g)

Gravity (denoted as 'g') is the force that accelerates the projectile downward at a rate of approximately 9.8 m/s². In the video, gravity is treated as a constant negative value in the equations for vertical motion, as it acts against the upward motion of the projectile. The negative sign indicates that gravity pulls the projectile downward.

💡Launch angle (theta)

The launch angle, denoted by the Greek letter theta (θ), is the angle at which the projectile is fired relative to the horizontal. In the video, the cannonball is launched at an angle of 20 degrees. The instructor breaks down the projectile's velocity into horizontal and vertical components based on this angle using trigonometry.

💡Horizontal displacement (x)

Horizontal displacement refers to the distance the projectile moves along the horizontal axis. The video focuses on calculating this displacement to determine the range of the projectile. The instructor simplifies the horizontal displacement equation by assuming no acceleration in the x-direction, making it dependent on the initial horizontal velocity and time.

💡Quadratic formula

The quadratic formula is used to solve equations of the form ax² + bx + c = 0. In the video, the instructor uses this formula to solve for the time of impact by setting the vertical displacement equation equal to zero. This helps find the total time the projectile stays in the air, which is crucial for calculating its range.

💡Components of velocity

The components of velocity refer to the horizontal (v_x) and vertical (v_y) parts of the overall velocity of the projectile. The video emphasizes breaking down the initial velocity into these components using trigonometry, which allows for separate analysis of the projectile's motion in the x- and y-directions. This approach simplifies the calculations for the projectile's trajectory.

Highlights

Introduction to solving a classic projectile motion problem involving an angled launch from uneven ground.

Clarifying known variables: height of the cliff (100 meters), initial velocity (30 meters per second), and launch angle (20 degrees).

Establishing the coordinate system and recognizing the direction of acceleration due to gravity as negative 9.8 m/s².

Deriving the equations for horizontal and vertical displacement and velocity using kinematic equations.

Simplifying the x-direction displacement equation to: x = v₀ cos(θ) t, given that horizontal acceleration is zero.

Solving for time of flight using the vertical displacement equation, recognizing that the vertical displacement at impact is zero.

Using the quadratic formula to solve for time, discarding the negative result, and finding the time of flight to be 5.68 seconds.

Calculating the horizontal range of the projectile as 160.1 meters after substituting values into the displacement equation.

Determining the peak height of the trajectory by solving for the time when the vertical component of velocity equals zero.

Finding the maximum vertical displacement (peak height) to be 105.4 meters at 1.05 seconds.

Calculating the velocity 2 seconds after launch, separating into horizontal (vₓ = 28.19 m/s) and vertical (vᵧ = -9.34 m/s) components.

Using Pythagoras' Theorem to determine the overall velocity magnitude (29.70 m/s) and direction (18.3° below horizontal).

Presenting the final velocity in vector form with both x and y components.

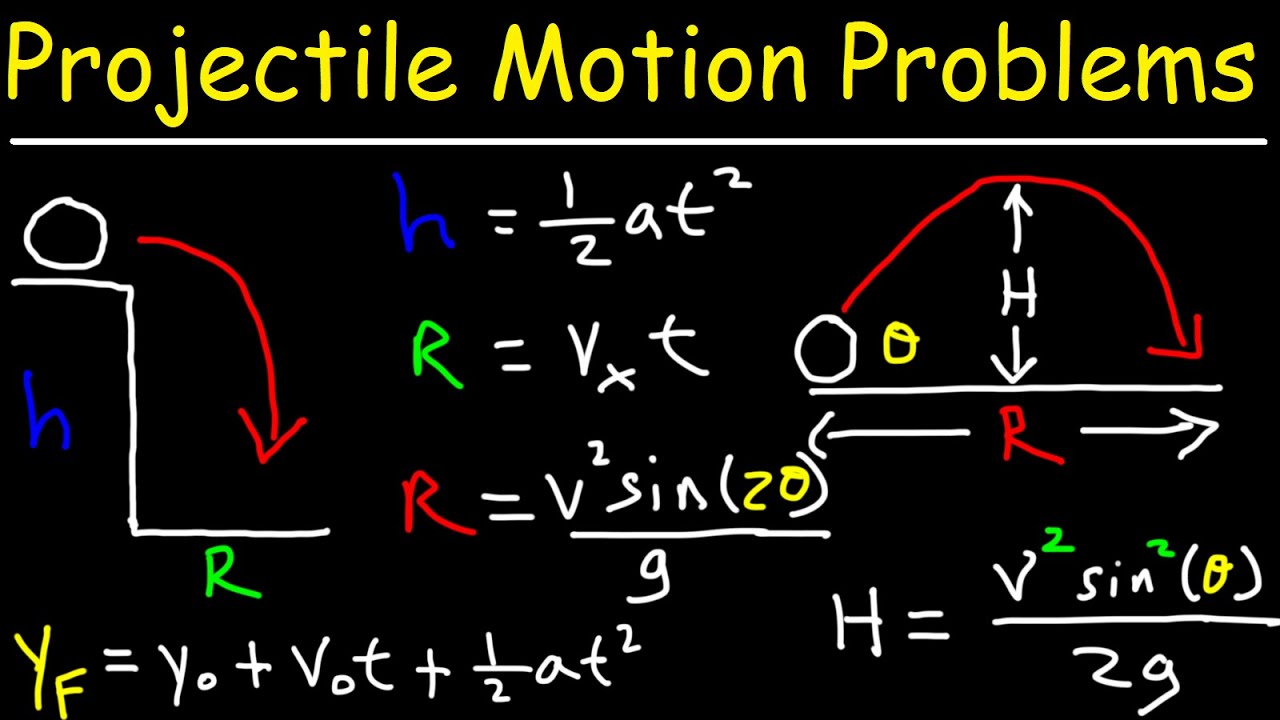
Emphasizing the importance of creating a toolbox to solve projectile motion problems efficiently.

Encouraging viewer interaction by inviting suggestions for future videos and general feedback.

Transcripts

Hello everyone. I'm Jesse Mason and in this installment of Teach Me, we're going to take

a look at a classic projectile motion problem involving an angled launch from uneven ground.

For this problem we're given the initial position and velocity of the projectile and our task

is to determine the projectile's range, peak height and velocity at some point in time

along its trajectory. The first step, as always, is to draw a picture.

So here's the cliff from which our projectile is launched; and here's the level ground below.

We'll draw a cannon to fire the projectile and also to mark's its initial position. (Loud

boom!) And there's our trajectory. "Take that, Pachelbel!"

And we're done with the picture. Not much to this one... yet.

So what are our knowns-n-unknowns? To start, we're given the height of the cliff, which

is to say the initial vertical displacement. That's one hundred meters. Of course, we've

already implied our coordinate system but we need to be explicit and include it on our

drawing. Next, we're given the magnitude of the launch velocity -- the initial speed -- of

our projectile: thirty meters per second. We're also given the direction of the launch

velocity, so we'll label the angle between the velocity vector and the horizontal as

theta. Theta is equal to twenty degrees.

So there are some of our knowns. There are a few more that are implied and we'll address

them shortly.

The first unknown we're tasked with determining is the projectile's horizontal displacement

upon impact: its range. We'll label this x. And by doing so, we've implied the location

of the origin so let's indicate that on our picture. NOW it should be clear to see that

our projectile's initial position along the x-axis is zero meters. We'll label the maximum

vertical displacement -- that is to say, our peak height - as y-max.

Lastly, we're looking for the projectile's velocity two seconds after launch. We're gonna

guess that the projectile -- a cannonball in this case - would be somewhere around...

here... at that time. Accuracy with our guess is unimportant because the equations will

tell us... the rest of the story. So at this point in the trajectory the instantaneous

velocity looks something like this. We're after the magnitude and the direction so we'll

label the angle between the velocity and the horizontal as phi. (Fee? Fi? Fee fi fo fum,

whatever. It's all Greek to me.) Annnnd let's not forget to jot down the given time for

this part. Since we'll be looking for each component of the projectile's velocity separately,

we'll break v into v-sub-x and v-sub-y. And while we're at it, let's resolve our initial

velocity into its constituent x- and y-components as well. So here's our given launch velocity,

v-sub-oh. In the vertical direction we have v-sub-oh-sub-y. And in the horizontal we have

v-sub-oh-sub-x and here's the given launch angle theta. Since we'll eventually be writing

these components in terms of v-sub-oh and theta, we'll write their trigonometric relationship

right here. Let's see... so the y-component of v-sub-oh is opposite theta, so it's equal

to v-sub-oh sine theta. And the x-component of v-sub-oh is adjacent to theta so it's equal

to v-sub-oh cosine theta.

By the way, we've got an implied known that should be listed: the acceleration due to

gravity. What value should we use for g? Or more to the point, is the magnitude of g positive

or negative? Well, it all depends on our coordinate system so we need to compare the acceleration

vector to our chosen coordinate system. What's the direction of the acceleration vector?

Downward, of course. Since we aligned the positive y-axis with the upward direction,

and the acceleration vector is downward, that means that the magnitude of g for this problem

will be negative 9.8 meters per second squared.

Okay! We're finished with identifying the knowns and unknowns. Now we're ready to move

on to the next step: selecting the appropriate equations for our problem.

Since we're dealing with projectiles and ignoring the effects of air resistance, the kinematic

equations -- namely, the displacement equation and the velocity equation -- are entirely

sufficient to analyze the motion of our projectile. Of course, our projectile is moving through

two dimensions so -- with a nod of appreciation to Galileo -- we'll apply these equations

to each direction separately.

So in the x-direction, starting with the displacement equation: Instead of s, we have x equals one

half a-sub-x (that's the acceleration in the x-direction) times t squared, plus v-sub-oh-sub-x

(the x-component of the initial velocity) times t, plus x-sub-oh (the initial horizontal

position). Before moving on, we're going to simplify this equation. A projectile's horizontal

motion is unaccelerated so we can set a-sub-x to zero. We've defined v-sub-oh-sub-x as v-sub-oh

cosine theta. And x-sub-oh is zero. So the displacement equation in the x-direction simplifies

to: x equals v-sub-oh cosine theta t. We'll label this equation (1) and set it aside for

now.

Now we apply the velocity equation to the x-direction and we get: v-sub-x equals v-sub-oh-sub-x,

plus a-sub-x times t. We'll set v-sub-oh-sub-x to v-sub-oh cosine theta. And again, the horizontal

acceleration of a projectile is zero. So the velocity equation in the x-direction simplifies

to v-sub-x equals v-sub-oh cosine theta. We'll label this equation (2) and set it aside.

Okay, so now we'll apply the kinematic equations to the y-direction. The displacement equation

becomes: y equals one half a-sub-y t squared, plus v-sub-oh-sub-y t, plus y-sub-oh. First,

a-sub-y, the acceleration in the y-direction, is the gravitational acceleration of our projectile

so we can set that to g. And we've defined v-sub-oh-sub-y (the y-component of our projectile's

initial velocity) as v-sub-oh sine theta. So the displacement equation in the y-direction

simplifies to become: y equals one half g t squared, plus v-sub-oh sine theta t, plus

y-sub-oh. Label that equation (3) and we're almost done here.

The velocity equation in the y-direction is v-sub-y equals v-sub-oh-sub-y, plus a-sub-y

times t. Again, v-sub-oh-sub-y was defined as v-sub-oh sine theta and a-sub-y is just

g. So our fourth and final equation is v-sub-y equals v-sub-oh sine theta plus g t.

These four equations now constitute what we call our toolbox. Every handyman knows you've

gotta have the right tools for the right job. The same goes for projectile motion: simplifying

the displacement and velocity equations for each direction is selecting the right tools.

And with these tools we can determine everything we want to know about our projectile's motion

and trajectory.

First up: determining the projectile's horizontal displacement upon impact, i.e., its range.

Where do we start? Well, equation (1) would give us horizontal displacement but we don't

have t, the time of impact. To get t, we're going to need to use equation (3) along with

a key implication: at the moment of impact, the projectile's vertical displacement is...

zero! Now we can solve equation (3) for time.

So we'll start by writing equation (3). We'll set y to zero and rewrite for clarity's sake.

Does this equation ring any algebraic bells? Perhaps if we recall it's more general form

and solution: If zero equals A t squared plus B t plus C (where A, B, and C are all constants),

then t equals negative B plus-or-minus the square root of B squared minus four A C all

over two A. Yep, that's how I remember the quadratic formula. So our one half g here

will play the role of A, our v-sub-oh sine theta will be B, and our y-sub-oh is C. So...

t equals (long inhale) negative v-sub-oh sine theta plus-or-minus the square root of quantity

v-sub-oh sine theta end quantity squared minus four times one half g y-sub-oh all divided

by 2 times one half g. (Applause) Thank ya, thank ya very much. Simplifying just a few

terms and then inserting our values we find that t equals negative 3.59 seconds or positive

5.68 seconds. The negative value is not useful for this problem -- negative time? what does

it mean? -- so we'll lovingly discard it.

Now we proceed with equation (1) -- write it out here -- and insert the appropriate

values: 30 meters per second, cosine twenty degrees and for time we use our 5.68 seconds

from equation (3). And we get a horizontal displacement of 160.1 meters. All things considered,

not an unreasonable range.

Okay, now let's determine the maximum vertical displacement of our projectile, that is, the

peak height of its trajectory. We'll use equation (3) to determine this value but we first need

to obtain the time which corresponds to this moment. To obtain the time we need to recognize

that the moment our projectile crests its trajectory, the vertical component of its

velocity is zero. Using this value, along with equation (4) will give us the time at

which our projectile reaches its peak height.

Okay, solving equation (4) for time, we get t equals negative v-sub-oh sine theta divided

by g. Inserting our values, we find that at 1.05 seconds our projectile levels out and

begins its descent, so to speak. Now we'll insert this time into equation (3) to determine

the maximum vertical displacement of our projectile. And when we assign our values to the variables

-- using 1.05 seconds, the time coincident with y-max, for t - we find that our projectile

reaches a peak height of 105.4 meters. Not very impressive, but very reasonable given

our shallow launch angle.

Lastly, we'll determine the projectile's velocity 2 seconds after launch. We'll need to determine

the x- and y-components separately so starting with v-sub-x, we'll use equation (2). V-sub-x

equals v-sub-oh cosine theta. Using the aforementioned known values we get 28.19 meters per second

for the horizontal component. We'll use equation (4) to determine the vertical component. Inserting

our known values, including the time in question -- two seconds - we get a value of negative

9.34 meters per second for v-sub-y. What's up with the negative sign? The negative sign

implies motion in the negative y-direction, that is, downward.

Now at this point we could write our solution in vector notation, where the velocity vector

is equal to the magnitude of the velocity's x-component times the unit vector i-hat, plus

the magnitude of the velocity's y-component times the unit vector j-hat. That would give

us the solution v equals 28.19 meters per second i-hat, plus negative 9.34 meters per

second j-hat... which is fine and dandy, but let's go ahead and determine the magnitude

and direction of this velocity vector.

So we've got 28.19 meters per second in the x-direction and 9.34 meters per second in

the negative y-direction. We'll recombine these components to determine the magnitude

of v using Euclid's 47th Proposition, better known as Pythagoras' Theorem: the square of

the hypotenuse is equal to the sum of the squares of the legs. Taking the square root

of both sides and inserting our values (and dropping the plus-or-minus sign) we get a

magnitude of 29.70 meters per second. Physically reasonable? Check. Are we done? Not yet, we

still need the direction of its motion, that is to say the angle between the velocity vector

and the horizontal. To get phi, we use trigonometry. So the tangent of phi equals opposite (9.34

meters per second) over adjacent (28.19 meters per second). Take an inverse tangent and we

get a value of 18.3 degrees for phi.

So two seconds after launch our projectile is moving at 29.70 meters per second at 18.3

degrees below the horizontal.

I'm Jesse Mason. I hope you found this video helpful and that I've convinced of the utility

-- nay, the necessity! -- of creating a toolbox to solve projectile motion problems. If you

have any suggestions for future Teach Me videos or just want to say hello from your part of

the world, please do so in the comments section below. And as always, happy learning!