How to Find Displacement in Physics
Summary
TLDRThis lesson delves into the concepts of displacement and distance in physics, emphasizing their differences and importance. It teaches how to calculate displacement without involving velocity, acceleration, or time, using the formula Δx = xf - xi for straight-line motion. The tutorial also covers the resultant displacement formula, applying it to various examples, including a car's journey and a farmer's path around a square field, demonstrating how displacement is a vector quantity with both magnitude and direction.
Takeaways
- 📚 The lesson focuses on understanding displacement formulas and their application in physics.
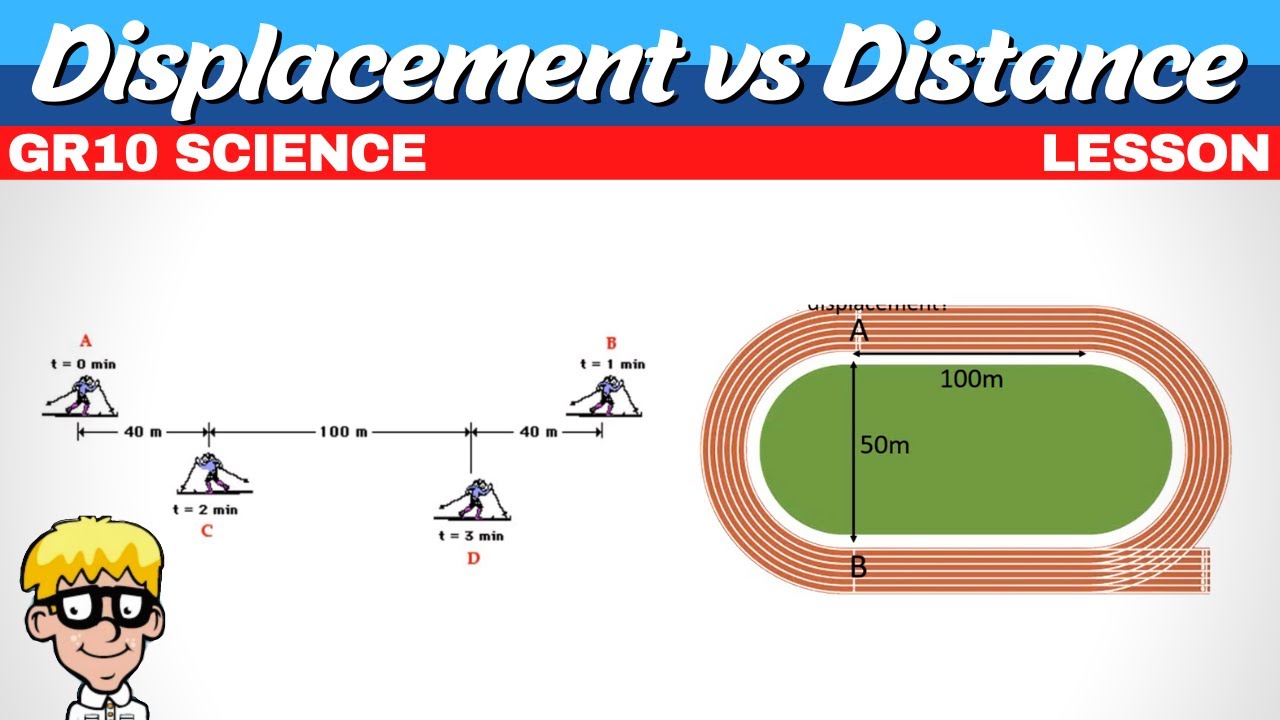
- 🔍 The terms 'distance' and 'displacement' are often confused but are distinct concepts; displacement is a vector quantity with both magnitude and direction.
- 📏 Displacement is defined as the change in position of an object from an initial point to a final point, represented as the shortest path between the two points.
- 📈 The formula for displacement when initial and final positions are known is given by \( \Delta x = x_f - x_i \), where \( x_f \) is the final position and \( x_i \) is the initial position.
- 📐 The resultant displacement formula is used when the initial and final positions are given relative to a reference point and is calculated using \( d = \sqrt{x^2 + y^2} \).
- 🚗 An example problem involves calculating the distance and displacement of a car moving from point A to B to C and back to B, illustrating the difference between distance traveled and displacement.
- 📐 For motion in two directions, the Pythagorean theorem is applied to find the magnitude of the resultant displacement, as shown in an example with eastward and northward movements.
- 🏞 The third example problem involves a farmer moving around a square field, demonstrating how to calculate displacement over multiple laps.
- ⏱ The time taken to move around the field once is used to calculate the number of laps and the resultant displacement after a given time.
- 🔢 The magnitude of displacement for the farmer's path is calculated using the Pythagorean theorem for the right-angled triangle formed by the sides of the square field.
- 🎓 The lesson concludes with a summary of the concepts and formulas discussed, emphasizing the importance of understanding displacement in physics.
Q & A
What is the difference between distance and displacement?
-Distance is the total path length traveled by an object, regardless of direction, while displacement is the change in position of an object, having both magnitude and direction, making it a vector quantity.
Why is displacement considered a vector quantity?
-Displacement is a vector quantity because it has both magnitude (the length of the path traveled from the initial to the final position) and direction (from the initial position to the final position).
How is displacement calculated when an object moves in a straight line?
-Displacement is calculated using the formula Δx = xf - xi, where xf is the final position and xi is the initial position of the object.
What is the resultant displacement formula used for?
-The resultant displacement formula is used when the initial and final positions of an object are given in terms of distance from a reference point, and it helps to find the magnitude and direction of displacement.
How is the magnitude of displacement calculated when an object moves in two different directions?
-The magnitude of displacement is calculated using the formula d = √(x^2 + y^2), where x and y are the distances moved in the respective directions.
What is the significance of the direction in displacement?
-The direction in displacement is significant because it indicates the path taken from the initial to the final position, which is crucial for understanding the object's change in position.
In the example of the car moving from point A to B to C and back to B, what is the total distance covered?
-The total distance covered by the car is 23 kilometers, calculated as the sum of the distances from A to B, B to C, C to B, and B to C again.
How is the magnitude of displacement calculated for the car's movement in the example?
-The magnitude of displacement for the car's movement is 7 kilometers, which is the straight-line distance from the initial point A to the final point C.
In the second example, what is the net distance traveled by the object moving 3 miles east and 4 miles north?
-The net distance traveled by the object is 7 miles, which is the sum of the distances moved east and north.
What is the magnitude of the net displacement for the object moving in two different directions in the second example?
-The magnitude of the net displacement is 5 miles, calculated using the Pythagorean theorem for the right-angled triangle formed by the eastward and northward movements.
For the farmer moving around a square field, what is the magnitude of displacement after 2 minutes and 20 seconds?
-The magnitude of displacement for the farmer after 2 minutes and 20 seconds is approximately 14.14 meters, calculated using the Pythagorean theorem for the right-angled triangle formed by the sides of the square.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
Displacement vs Distance Grade 10 Science

Conceitos Iniciais de CINEMÁTICA - [Cinemática do Zero 01]

Vektor Posisi, Kecepatan, dan Percepatan | Kinematika 2D & 3D | Part 1 | Fisika Dasar

Cinemática 02: Deslocamento e distância percorrida

Linear Motion - Distance, Displacement, Speed, Velocity, Acceleration - SPM & IGSCE Physics

Position/Velocity/Acceleration Part 1: Definitions
5.0 / 5 (0 votes)
