Pythagorean Theorem
Summary
TLDRThis video tutorial covers the Pythagorean theorem, demonstrating its application in solving geometric problems. It explains how to calculate the hypotenuse of a right triangle, find the area of a square given its diagonal, and determine the perimeter of a rhombus and the area of an isosceles trapezoid using the theorem.
Takeaways
- 📐 The Pythagorean theorem is used to solve problems in geometry involving right triangles.
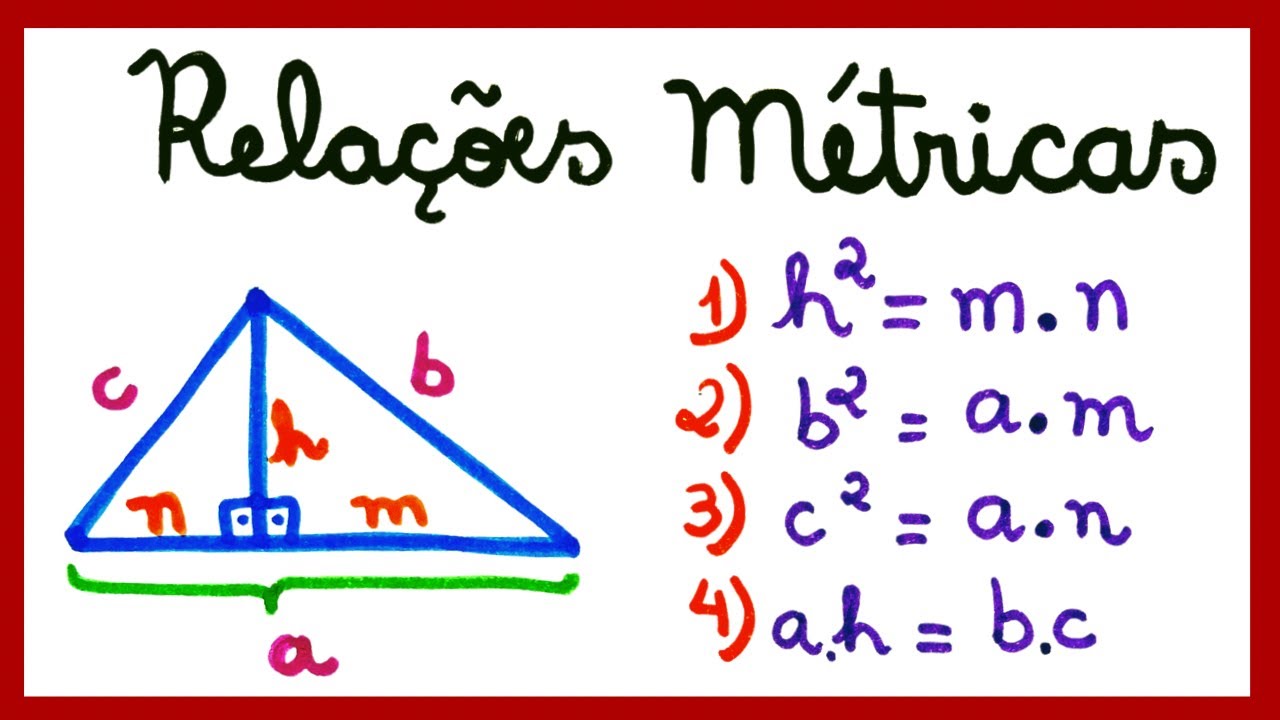
- 📝 The formula for the Pythagorean theorem is \( c^2 = a^2 + b^2 \), where \( c \) is the hypotenuse and \( a \) and \( b \) are the legs.
- 🔍 Example 1: For a right triangle with legs 5 and 12, the hypotenuse \( x \) is calculated as 13.
- 🔢 Example 2: For a right triangle with hypotenuse 10 and one leg 5, the other leg \( y \) is \( 5\sqrt{3} \).
- 🏠 Example 3: To find the area of a square with a diagonal of 12 inches, use the Pythagorean theorem to find the side length and then square it. The area is 72 square inches.
- 🔺 The diagonals of a rhombus bisect each other at right angles.
- 🔄 In a rhombus, all four sides are congruent, and the perimeter can be calculated by determining one side using the Pythagorean theorem and then multiplying by four.
- 🔷 Example 4: For a rhombus with diagonals 14 and 48, each side is 25 units, making the perimeter 100 units.
- 🔻 Example 5: To find the area of an isosceles trapezoid with bases 12 and 20 and legs 5, calculate the height using the Pythagorean theorem and then use the area formula. The area is 48 square units.
- 📏 Important formulas: Area of a square is \( side^2 \), and area of a trapezoid is \( \frac{1}{2}(base1 + base2) \times height \).
Q & A
What is the Pythagorean theorem?
-The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. It is expressed as \( C^2 = a^2 + b^2 \), where \( C \) is the hypotenuse and \( a \) and \( b \) are the other two sides.
How is the Pythagorean theorem used to find the hypotenuse of a right triangle?
-To find the hypotenuse of a right triangle, you can use the formula \( C^2 = a^2 + b^2 \). You substitute the values of the other two sides into the formula, calculate \( C^2 \), and then take the square root of the result to find \( C \).
What is the formula used to calculate the area of a square given its diagonal length?
-The formula to calculate the area of a square given its diagonal length \( d \) is \( \text{Area} = \frac{d^2}{2} \). This is derived from the Pythagorean theorem, where the diagonal forms the hypotenuse of two right triangles within the square.
How can you find the perimeter of a rhombus if you know the lengths of its diagonals?
-To find the perimeter of a rhombus, you first determine the length of one side using the Pythagorean theorem, since the diagonals bisect each other at right angles. If the diagonals are \( d_1 \) and \( d_2 \), the side length \( s \) is \( \sqrt{\frac{d_1^2 + d_2^2}{2}} \). The perimeter is then \( 4s \).
What is the formula for calculating the area of an isosceles trapezoid?
-The formula for calculating the area of an isosceles trapezoid is \( \text{Area} = \frac{1}{2} (b_1 + b_2) \times h \), where \( b_1 \) and \( b_2 \) are the lengths of the two bases and \( h \) is the height of the trapezoid.
How do you calculate the height of an isosceles trapezoid if you know the lengths of the bases and the non-parallel sides?
-To calculate the height of an isosceles trapezoid, you can use the Pythagorean theorem on one of the right triangles formed by drawing a height from one base to the other. If the bases are \( b_1 \) and \( b_2 \), and the non-parallel sides are equal, you can set up the equation \( h^2 + \frac{(b_2 - b_1)^2}{4} = \text{side}^2 \) and solve for \( h \).
Why is the Pythagorean theorem useful in solving geometry problems?
-The Pythagorean theorem is useful in solving geometry problems because it relates the lengths of the sides of a right triangle, allowing you to find unknown side lengths, calculate areas of shapes like squares and rhombuses, and determine perimeters of shapes like rhombuses.
What is the relationship between the diagonals of a rhombus?
-In a rhombus, the diagonals bisect each other at right angles and are perpendicular to each other. This means that each diagonal cuts the other into two equal parts, forming four congruent right triangles within the rhombus.
How can you simplify the square root of 75 when calculating the area of a square with a diagonal of 12 inches?
-To simplify the square root of 75, you can factor 75 into \( 25 \times 3 \). Since the square root of 25 is 5, the square root of 75 simplifies to \( 5\sqrt{3} \).
What is the perimeter of a rhombus with diagonals measuring 7 and 24 units?
-Using the Pythagorean theorem, the side length \( s \) of the rhombus is \( \sqrt{576 + 49} = \sqrt{625} = 25 \). The perimeter is \( 4 \times 25 = 100 \) units.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenant5.0 / 5 (0 votes)