Menentukan Kuartil Q1 Q2 Q3 Median Mean Modus Statistika kelas 12

Summary

In this educational video, the host explains statistical concepts for 12th-grade students, focusing on measures like mean, mode, and quartiles using a sample dataset. The video provides step-by-step guidance on how to calculate these statistical measures, including detailed instructions for finding the mean, mode, and quartiles (Q1, Q2, and Q3) of the given dataset. The explanation is clear, with examples using frequencies and midpoint values, making complex concepts accessible even for those with little prior knowledge of statistics.

Takeaways

• 😀 Understanding statistics for 12th grade, with a focus on mean, mode, and quartiles.
• 😀 The sample data used is from a CPNS (Civil Service Exam), showing only frequency and value columns.
• 😀 The midpoint (X) of each class interval is calculated by averaging the lower and upper bounds.
• 😀 The frequency interval is 10, derived by subtracting the lower bound from the upper bound and adding 1.
• 😀 The mean (Min) is calculated by multiplying frequency (Fi) by midpoint (Xi), summing these values, and dividing by the total frequency (n).
• 😀 The mode (Modus) is found by identifying the class with the highest frequency and using a formula involving the frequency of neighboring classes.
• 😀 The lower boundary of the mode class is adjusted by subtracting 0.5 from the lower bound of the interval.
• 😀 Quartile 1 (Q1) is determined by calculating one-fourth of the total frequency (n) and finding the corresponding class interval.
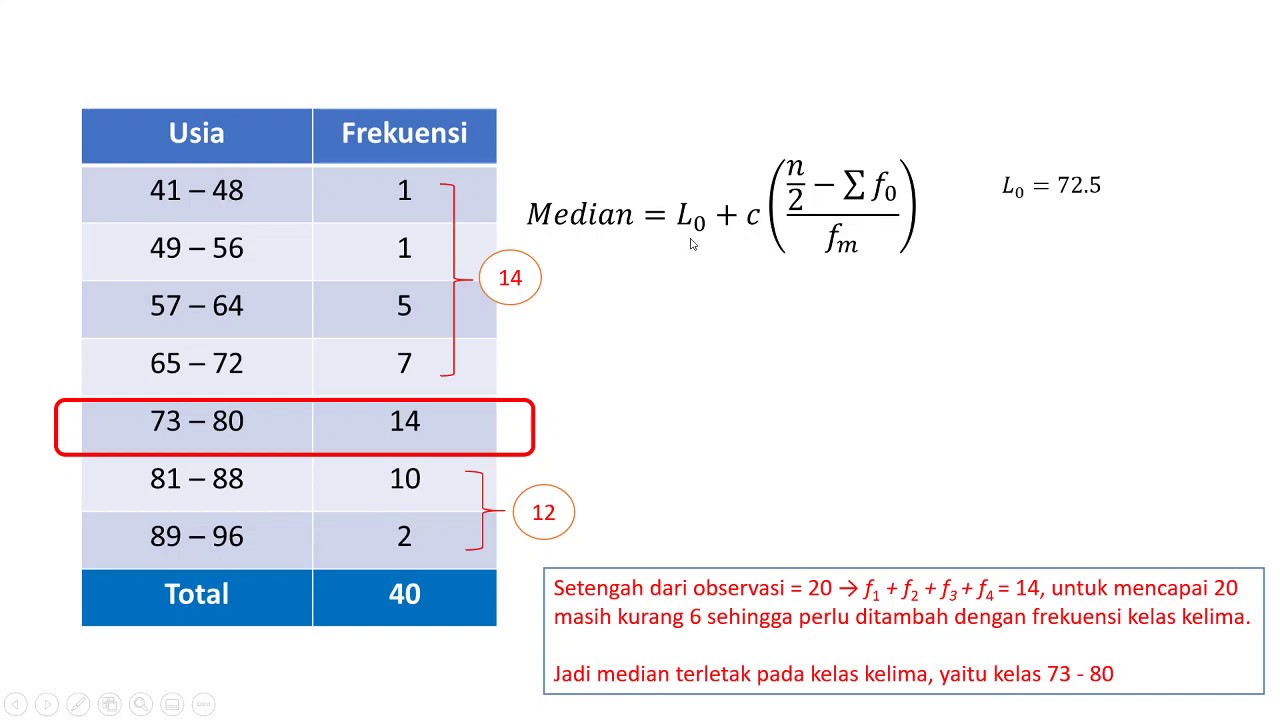
• 😀 Quartile 2 (Q2), also known as the median, corresponds to the middle of the data and is calculated as half of the total frequency (n).
• 😀 Quartile 3 (Q3) is calculated by multiplying three-fourths of the total frequency (n) and determining the corresponding class interval.
• 😀 Each quartile’s lower boundary is adjusted by subtracting 0.5 from the lower bound of its class interval for precise calculation.

Q & A

What is the primary focus of the video?

The video focuses on teaching statistics, particularly for class 12, and covers concepts like calculating mean, mode, quartiles, and other statistical measures using a sample dataset.

How is the midpoint (x) of an interval calculated in this video?

The midpoint (x) of an interval is calculated by adding the lower and upper bounds of the interval and then dividing the sum by 2. For example, for the interval 51-60, the midpoint is (51 + 60) / 2 = 55.5.

Why is the interval size important in this video?

The interval size is important for calculating other statistical measures like mean, mode, and quartiles. The video explains how the interval is derived by subtracting the lower bound from the upper bound and adding 1.

How is the mean (average) calculated in the given example?

To calculate the mean, you multiply each midpoint (x) by its corresponding frequency (Fi), sum these values, and divide by the total frequency (n). In the example, the sum of Fi * x is 3150, and dividing by the total frequency (40) gives a mean of 78.75.

What is the process to determine the mode in this example?

To determine the mode, the class interval with the highest frequency is identified. In this case, the interval with the highest frequency is 71-80, and the mode is calculated using the formula that adjusts the boundaries by subtracting 0.5 from the lower bound and adding the interval size.

What is the significance of Q1 (first quartile) in this context?

Q1 represents the value below which 25% of the data lies. To find Q1, the video explains how to calculate the 10th value based on the frequency distribution and adjust the boundaries similarly to how the mode is calculated.

How is Q2 (second quartile) different from Q1?

Q2, or the median, represents the middle value of the data. The process to find Q2 is similar to finding Q1 but involves calculating the 20th value in the frequency distribution. In this example, Q2 coincidentally overlaps with the mode class interval.

How is Q3 (third quartile) determined?

Q3 represents the value below which 75% of the data lies. To calculate Q3, the video shows how to calculate the 30th value using the cumulative frequency and adjust the boundaries as done for Q1 and Q2.

Why is it necessary to calculate the cumulative frequency for quartiles?

Cumulative frequency is necessary to determine the specific class intervals corresponding to Q1, Q2, and Q3. It allows for the identification of the data points that correspond to these quartiles, helping to calculate their exact values.

What role does the interval size play in quartile calculations?

The interval size is crucial for determining the precise boundaries of each quartile. By adjusting the boundaries based on the interval size, you can accurately find the specific class intervals that represent the quartiles.