PERTIDAKSAMAAN LINEAR DUA VARIABEL
Summary
TLDRThis educational video covers the topic of linear inequalities in two variables. It explains the concept of such inequalities, which involve two variables with a degree of one, connected by inequality signs such as greater than, less than, and equal to. The video presents two methods for solving these inequalities: the point-test method and the sign-analysis method. The tutorial guides viewers through determining solution regions on a graph, with practical examples demonstrating how to solve and graph these inequalities. It concludes with a clear explanation of different solution areas depending on the inequality type.
Takeaways
- 😀 Linear inequalities with two variables are mathematical expressions involving two variables, usually x and y, each with degree 1, and linked by inequality signs (>, <, ≥, ≤).
- 😀 The general form of a linear inequality with two variables can be written as: x + by ≤ c, where x and y are the variables, and A, B, and C are real numbers.
- 😀 The solution to a linear inequality with two variables often represents a region on a graph.
- 😀 There are two primary methods for solving linear inequalities with two variables: the Point Testing Method and the Sign Method.
- 😀 The Point Testing Method involves plotting the inequality as a straight line, choosing a test point (usually (0,0)), and determining which side of the line satisfies the inequality.
- 😀 If the test point satisfies the inequality, the region that includes the test point is the solution region.
- 😀 The Sign Method focuses on the coefficients of x and y in the inequality, adjusting signs as necessary (e.g., multiplying by -1) to simplify the problem.
- 😀 When multiplying or dividing by a negative number, remember that the inequality sign must be reversed.
- 😀 The direction of the solution region depends on the inequality sign: if it is ‘<’ or ‘≤’, the solution lies below or to the left of the line; if it is ‘>’ or ‘≥’, the solution lies above or to the right of the line.
- 😀 Multiple examples are provided in the script, demonstrating the application of both the Point Testing Method and the Sign Method to solve linear inequalities.
- 😀 The script emphasizes the importance of understanding both methods and practicing them to become proficient in solving linear inequalities with two variables.
Q & A
What is a linear inequality in two variables?
-A linear inequality in two variables is a mathematical open sentence that involves two variables, each having a degree of one. The inequality is expressed with a relation such as 'greater than', 'less than', 'greater than or equal to', or 'less than or equal to'.
What are the general forms of a linear inequality in two variables?
-A common general form of a linear inequality in two variables is 'x + by ≤ c', where 'x' and 'y' are the variables, and 'a', 'b', and 'c' are real numbers. The inequality sign can vary, such as '>', '<', '≥', or '≤'.
What are the two methods for solving a linear inequality in two variables?
-The two methods for solving linear inequalities in two variables are: 1) the 'test point method', and 2) the 'sign analysis method'.
How does the test point method work for solving linear inequalities?
-In the test point method, the first step is to graph the inequality as a straight line. Then, a point that does not lie on the line (often (0,0)) is tested by substituting it into the inequality. If the point satisfies the inequality, the region containing the point is the solution region.
How is the sign analysis method different from the test point method?
-In the sign analysis method, the first step is similar to the test point method, where you find the intercepts with the axes. Afterward, you consider the sign of the inequality. If the coefficient of 'x' is positive, the solution lies to the right of the line. If the coefficient is negative, the solution lies to the left. The inequality sign also determines whether the solution is above or below the line.
What happens if the inequality is multiplied or divided by a negative number?
-If an inequality is multiplied or divided by a negative number, the direction of the inequality sign must be reversed. For example, 'x > 5' becomes 'x < 5' when multiplied by -1.
What does it mean if the inequality symbol is '≤' or '≥'?
-If the inequality symbol is '≤' or '≥', the solution region includes the line, and the line itself is part of the solution set. If the symbol is '<' or '>', the line is not included in the solution.
What is the importance of the coefficient sign when solving linear inequalities?
-The sign of the coefficient of 'x' plays a crucial role in determining which side of the line contains the solution. A positive coefficient indicates the solution is to the right or above the line, while a negative coefficient indicates the solution is to the left or below the line.
What happens if a point like (0,0) does not satisfy the inequality in the test point method?
-If the point (0,0) does not satisfy the inequality, then the solution region is the area that does not contain (0,0). In this case, the region opposite to where the point lies is the solution.
Can you provide an example of a linear inequality problem and its solution?
-For example, consider the inequality 'x + y ≤ 5'. First, we change it to the equation 'x + y = 5' to find the intercepts. The x-intercept is (5,0), and the y-intercept is (0,5). Then, by testing the point (0,0), we find that it satisfies the inequality, so the solution region is the area below the line.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

SPtLDV (Sistem Pertidaksamaan Linear Dua Variabel) Kelas 10 Kurikulum Merdeka

MENENTUKAN MODEL MATEMATIKA DARI SOAL CERITA SPtLDV
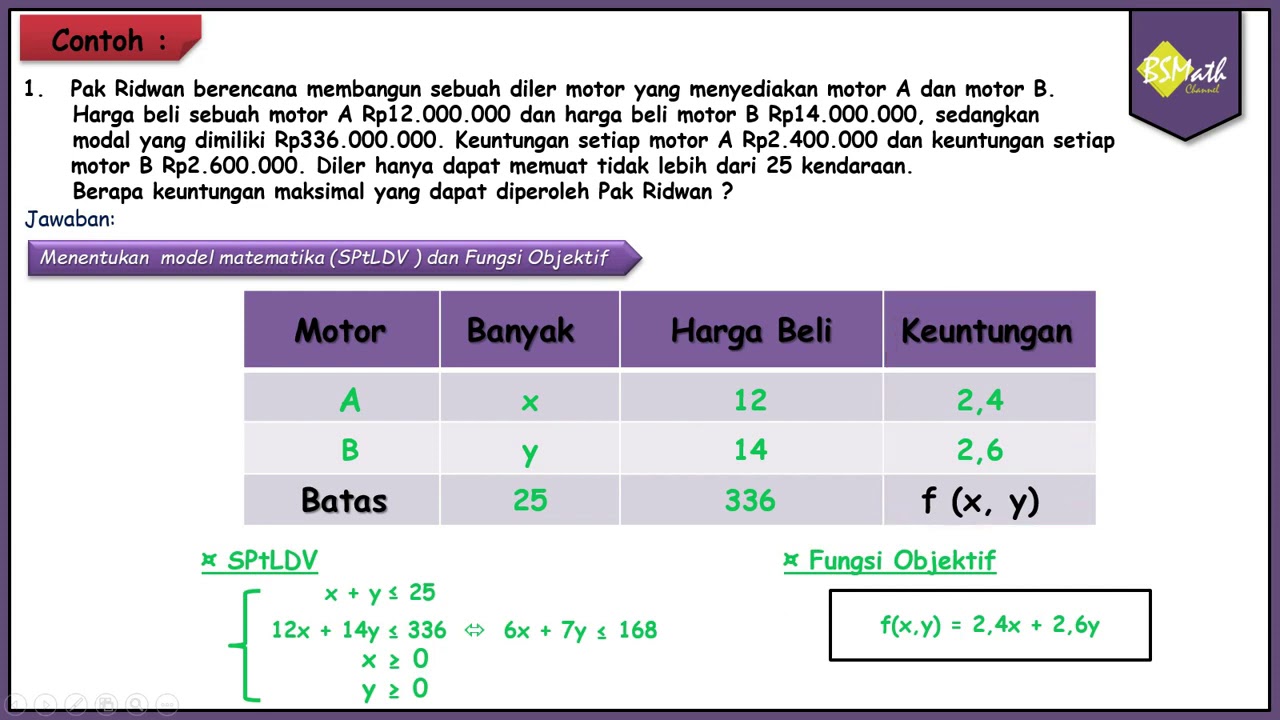
Menyelesaikan Permasalahan Program Linear Menentukan Nilai Optimum dengan Metode Uji Titik Pojok

ILLUSTRATING LINEAR INEQUALITIES IN TWO VARIABLES || GRADE 8 MATHEMATICS Q2

SPtDV Matematika Kelas 10 • Part 6: Contoh Soal Sistem Pertidaksamaan Linear Dua Variabel / SPtLDV

memahami konsep persamaan linear satu variabel
5.0 / 5 (0 votes)
