Cara Menghitung Panjang Busur dan Luas Juring Lingkaran
Summary
TLDRIn this educational video, the speaker explains how to calculate the length of an arc and the area of a sector in a circle. Using an example with a circle's radius of 35 cm and a central angle of 72 degrees, the speaker demonstrates how to find the arc length (44 cm) and the sector area (770 cm²). The process involves straightforward formulas: arc length = (central angle/360) × circumference and sector area = (central angle/360) × area of the circle. The tutorial is simple and provides clear steps to understand these geometric concepts.
Takeaways
- 😀 The script discusses the calculation of arc length and sector area in a circle.
- 😀 The radius (OA) of the circle is given as 35 cm, and the central angle (AOB) is 72°.
- 😀 To find the arc length, we use the formula: Arc Length = (Central Angle / 360°) × Circumference of the circle.
- 😀 The circumference of a circle is calculated using the formula: Circumference = 2πr, where r is the radius.
- 😀 The value of π used in the calculations is 22/7 because the radius is a multiple of 7.
- 😀 For arc length, the central angle (72°) is divided by 360° and multiplied by the circle's circumference.
- 😀 After simplifying the fractions and calculations, the arc length AB is found to be 44 cm.
- 😀 To calculate the area of the sector, the formula used is: Area of Sector = (Central Angle / 360°) × Area of the circle.
- 😀 The area of the circle is found using the formula: Area = πr², where r is the radius.
- 😀 After simplifying the calculations, the area of sector AOB is determined to be 770 cm².
- 😀 The script emphasizes understanding the difference between formulas for arc length and sector area in a circle.
Q & A
What is the main topic discussed in the video?
-The main topic is about calculating the arc length and sector area of a circle, with a focus on applying formulas to specific problems.
What are the two key concepts explained in the video?
-The two key concepts are the calculation of the arc length (panjang busur) and the sector area (luas juring) of a circle.
What formula is used to calculate the arc length of a circle?
-The formula to calculate the arc length is: Arc Length = (Central Angle / 360°) × Circumference of Circle, where the Circumference is 2πr.
What is the formula to calculate the area of a sector (juring) of a circle?
-The formula to calculate the area of a sector is: Sector Area = (Central Angle / 360°) × Area of Circle, where the Area of Circle is πr².
How is the central angle used in the formulas for arc length and sector area?
-The central angle is a fraction of 360° that is multiplied by the circumference (for arc length) or the area (for sector area) of the circle to calculate the desired measurements.
In the example problem, what is the radius of the circle?
-The radius of the circle in the example is given as 35 cm.
How was the arc length AB calculated in the example?
-The arc length AB was calculated by using the formula: Arc Length = (72 / 360) × 2 × π × 35, which simplifies to 44 cm.
What was the result of calculating the sector area in the example?
-The sector area was calculated as 770 cm² using the formula: Sector Area = (72 / 360) × π × 35².
Why is 22/7 used for π in the example calculations?
-22/7 is used for π because the radius (35 cm) is a multiple of 7, making 22/7 a more convenient approximation for π in the calculation.
What steps are involved in simplifying the calculation of the arc length and sector area?
-To simplify the calculations, we divide the central angle and 360 by common factors, cancel out terms where possible, and use a convenient value for π (like 22/7) based on the radius of the circle.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

Lingkaran [Part 3] - Panjang Busur dan Luas Juring
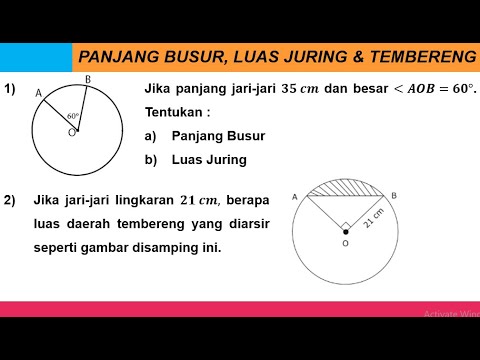
Cara Menghitung Panjang Busur, Luas Juring dan Luas Tembereng Pada Lingkaran

Materi Matematika Kelas 8: Lingkaran
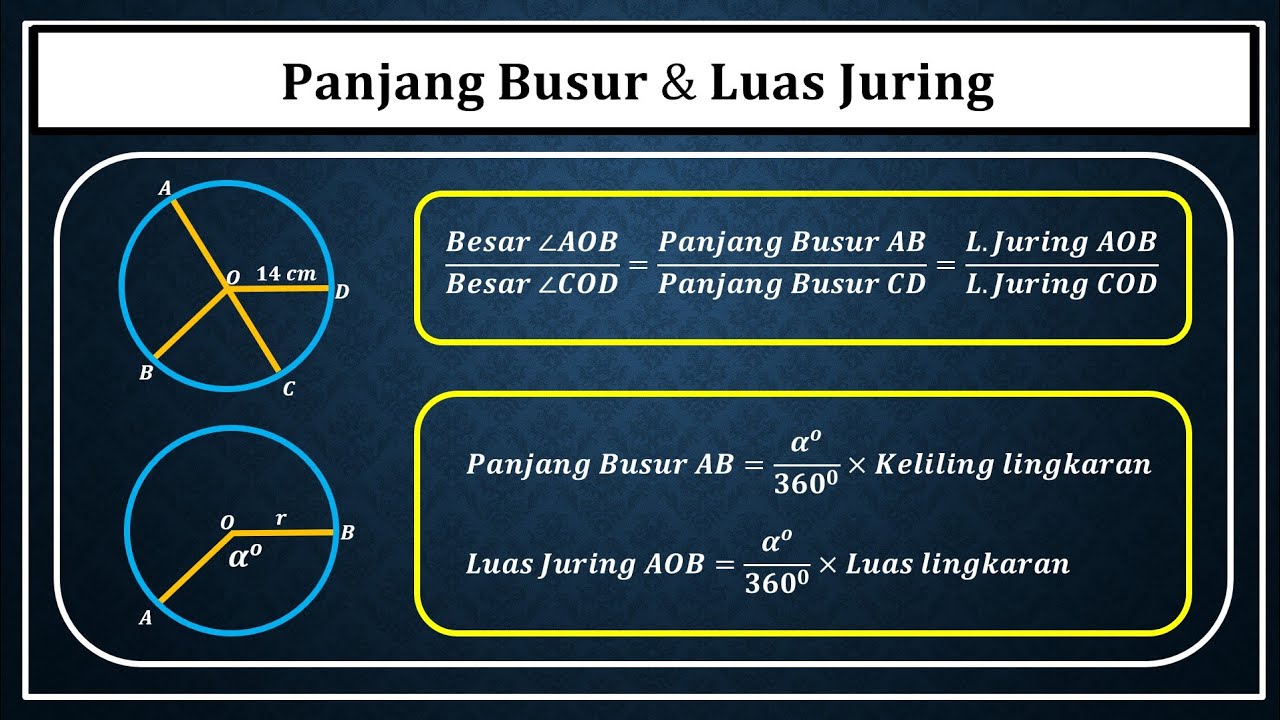
Panjang Busur dan Luas Juring Lingkaran
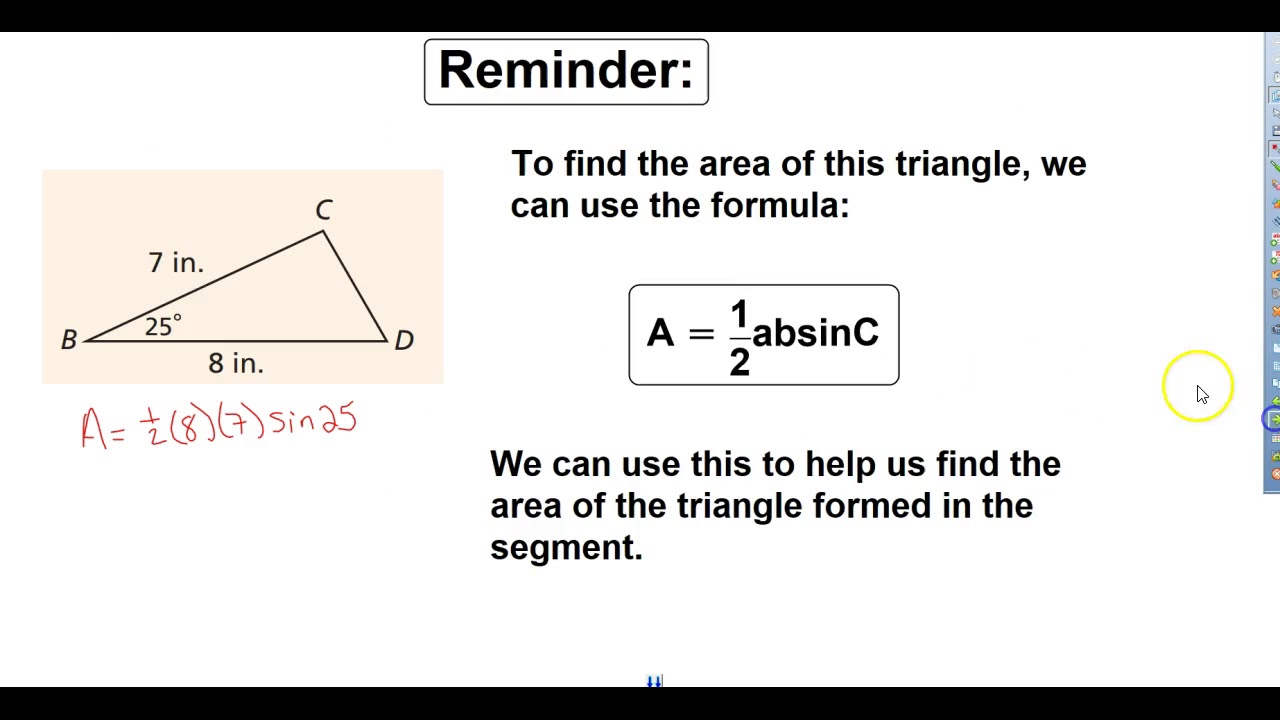
12-3 Sector Area and Arc Length

Lingkaran (Bagian 4) - Panjang Busur, Luas Juring & Luas Tembereng | Soal dan Pembahasan SMP MTs
5.0 / 5 (0 votes)
