Ultimate Gauss' Law review
Summary
TLDRThe video delves into Gauss's Law, explaining its application in various symmetrical charge distributions, particularly cylindrical and planar symmetries. It details how to calculate electric fields using Gaussian surfaces, emphasizing the role of charge density and the need for integration in non-uniform scenarios. The presentation outlines key formulas and concepts, such as the relationship between enclosed charge and electric field strength, demonstrating the consistent nature of the electric field around infinite planes. Overall, it serves as a comprehensive guide for understanding the practical applications of Gauss's Law in electrostatics.
Takeaways
- 😀 Gauss's Law relates the electric flux through a closed surface to the charge enclosed by that surface.
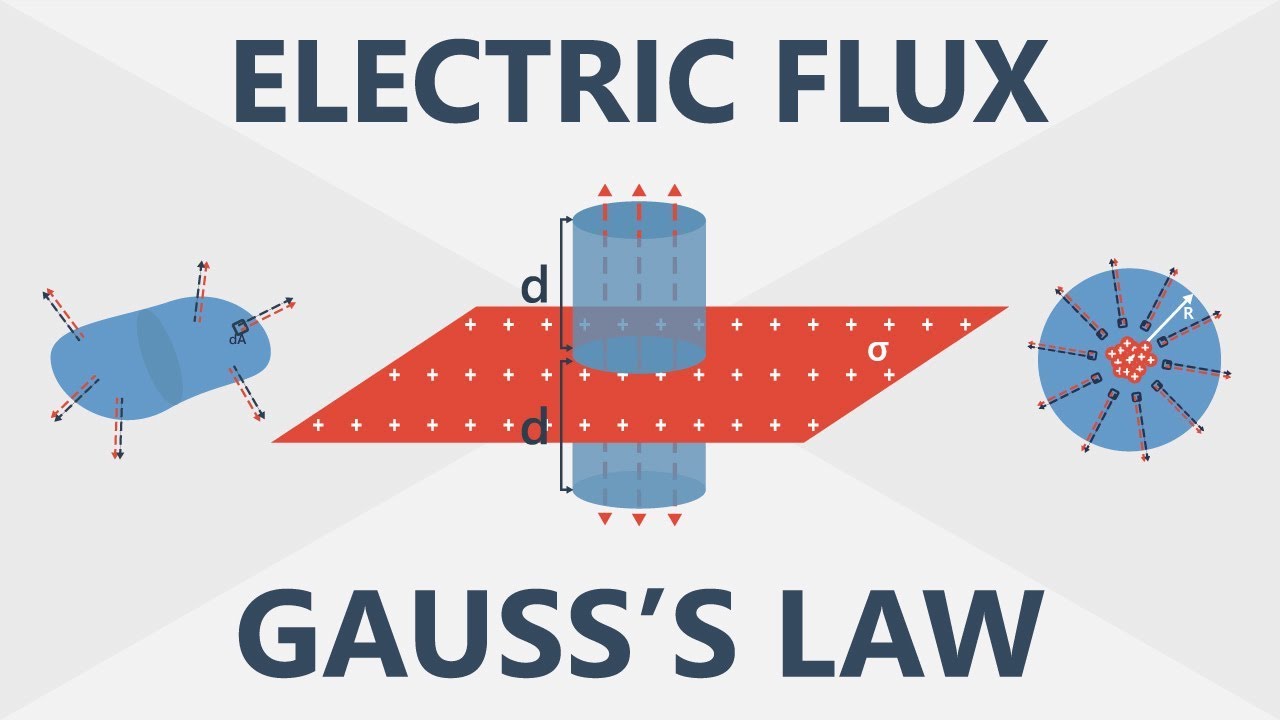
- 📏 For cylindrical symmetry, the electric field can be calculated using a Gaussian surface that simplifies the problem.
- 🔌 A line of charge has a linear charge density λ, and the enclosed charge can be found using λ times the length of the cylinder.
- 📦 When dealing with insulating cylinders, the charge density ρ is used with the volume to determine the charge enclosed.
- 🔄 The electric field outside a charged cylinder can be derived from the total charge, while the field inside can be zero depending on the radius.
- 📏 For non-uniform charge distributions, integration is required to calculate total charge by integrating charge density over volume.
- 🛠️ The volume differential for cylindrical coordinates involves the formula 2πRH dR for calculations.
- 📏 Planar symmetry leads to a uniform electric field that remains constant regardless of the distance from the plane.
- 📊 The surface charge density σ determines the electric field produced by an infinite plane, calculated as E = σ / (2ε₀).
- 📉 The electric field from an infinite plane does not diminish with distance, a unique feature that distinguishes it from other configurations.
Q & A
What is Gauss's law and how is it applied in the context of electric fields?
-Gauss's law relates the electric flux through a closed surface to the charge enclosed by that surface. It is applied by selecting a Gaussian surface that simplifies the calculation of electric fields based on symmetry.
What is the significance of using cylindrical symmetry when applying Gauss's law?
-Cylindrical symmetry simplifies the calculations for electric fields around long, charged cylinders, allowing us to determine the electric field as a function of distance from the axis.
How do you calculate the total charge enclosed by a Gaussian surface around a charged cylinder?
-The total charge enclosed is calculated by multiplying the linear charge density (λ) by the height of the cylinder (H), resulting in Q = λ × H.
What is the formula for the electric field inside a uniformly charged insulating cylinder?
-The electric field inside a uniformly charged insulating cylinder is derived by considering the charge density (ρ) and integrating it over the volume enclosed by the Gaussian surface.
When the radius of the Gaussian surface is less than the radius of the charged cylinder, how is the charge calculated?
-For a Gaussian surface with a radius less than the charged cylinder, the charge is calculated using the volume of the cylinder portion enclosed, leading to Q = ρ × πr²H, where r is the radius of the Gaussian surface.
What challenges arise when dealing with non-uniform charge distributions?
-With non-uniform charge distributions, one must integrate the charge density (ρ) over the appropriate volume, taking care to account for variations in density throughout the volume.
In the case of an infinite plane of charge, how is the electric field derived?
-For an infinite plane of charge, the electric field is derived by calculating the flux through the top and bottom faces of a Gaussian box, leading to E = σ / (2ε₀), where σ is the surface charge density.
Why is the electric field constant at any distance from an infinite plane?
-The electric field remains constant at any distance from an infinite plane because the contributions from all parts of the plane add up uniformly, leading to no dependence on distance.
How do you calculate the electric field for multiple planes of charge?
-For multiple planes, calculate the electric field due to each plane independently and then add the contributions vectorially, considering the direction of each field.
What is the role of charge density in determining the electric field?
-Charge density (whether linear, surface, or volume) directly influences the total charge enclosed and thus the strength of the electric field calculated using Gauss's law.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
5.0 / 5 (0 votes)